prove using vectors: The quadrilateral obtained by joining mid-points of adjacent sides a rectangle is a rhombus.
Video Solution
Text Solution
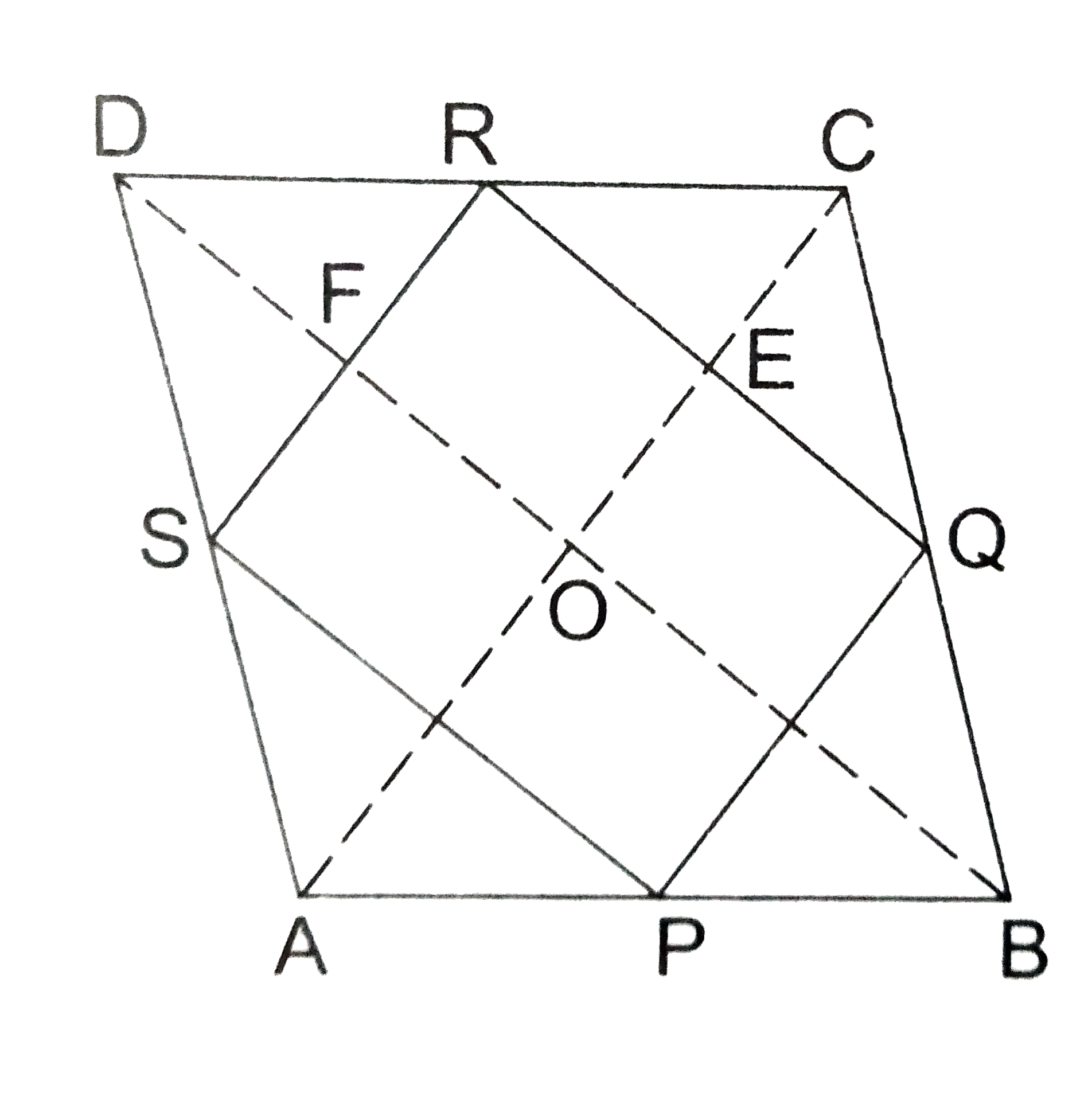
Let ABCD be a rhombus and P, Q, R, S be the midpoints of AB, BC, CD and DA respectively. Then, PQRS is a ||gm [prove it].
Diagonals of a rhombus bisect each other at right angles.
∴∠EOF=90∘.
Now, RQ||DB ⇒ RE||FO, SR||AC ⇒ FR||OE.
∴∠EOF=90∘.
∴OERF is a ||gm. So, ∠FRE=∠EOF=90∘.
Thus, PQRS is a ||gm. with ∠R=90∘.
Hence, PQRS is a rectangle.
|
Similar Questions
Knowledge Check
The figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8 cm and 6 cm, is
Aa rectangle of area 24cm2Ba square of area 25cm2Ca trapezium of area 24cm2Da rhombus of area 24cm2Consider the following statements
1. The bisectors of all the four angles of a parallelogram enclose a rectangle.
2. The figure formed by joining the midpoints of the adjacent sides of a rectangle is a rhombus.
3. The figure formed by joining the midpoints of the adjacent sides of a rhombus is a square.
Which of these statements are correct ?A1 and 2B2 and 3C3 and 1D1, 2 and 3The figure formed by joining the mid-points of adjacent sides of a rhombus is
Aa squareBrectangleCtrapeziumDnone of these
Show that the quadrilateral formed by joining the mid-points of the consecutive sides of a rectangle is a rhombus.
View SolutionShow that the quadrilateral formed by joining the mid-points of the consecutive sides of a rectangle is a rhombus.
View SolutionThe diagonals of a quadrilateral ABCD are equal. Prove that the quardilateral formed by joining the midpoints of its sides is a rhombus.
View SolutionThe diagonals of a quadrilateral ABCD are mutually perpendicular . Prove that the quadrilateral formed by joining the mid-points of its consecutive sides is a reactangle.

View SolutionThe figure formed by joining the midpoints of the adjacent sides of a rectangle of sides 8 cm and 6 cm is a
View Solution
