Two concentric circles of radii $a$ and $b (a > b)$ are given. Find the length of the chord of the larger circle which touches the smaller circle.

A

$2 \sqrt{a^{2} + b^{2}}$

B

$2 \sqrt{b^{2} - a^{2}}$

C

$2 \sqrt{a^{2} - b^{2}}$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

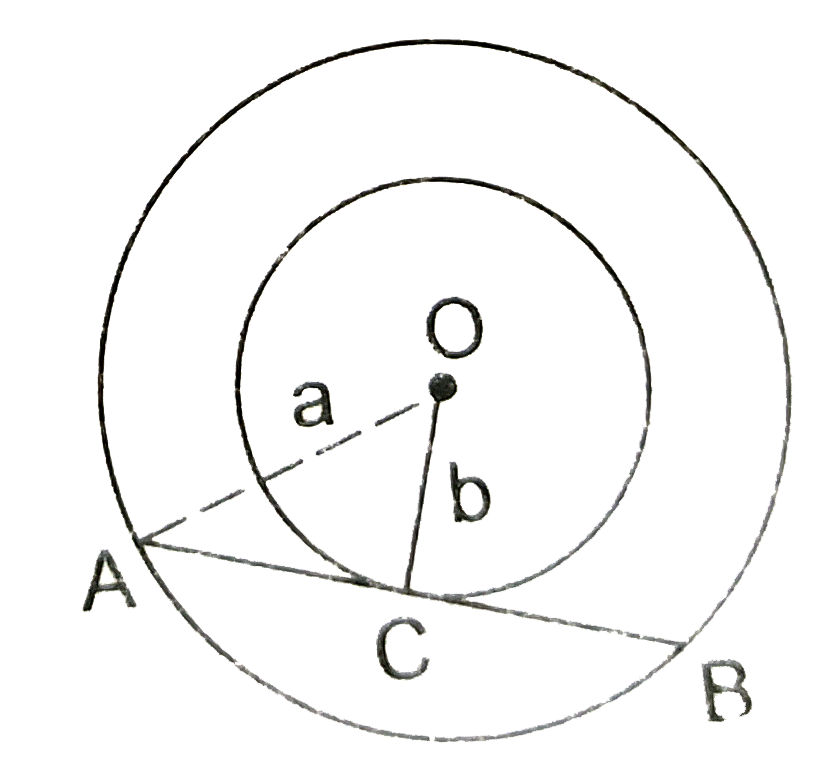
Let $O$ be the comon centre of the two circles and $A B$ be the chord of the larger circle which touches the samller circle at $C$ . Join $O A$ and $O C$ . Then, $O A = a$ and $O C = b$ .
Now, $O C ⊥ A B$ and $O C$ bisects $A B$ [ $∵$ the chord of the larger circle touching the smaller circle, is bisected at the point of contact]
In right, $Δ A C O$ , we have
$O A^{2} = O C^{2} + A C^{2}$ [ by Pythagoras' theorem]
$\Rightarrow A C = \sqrt{O A^{2} - O C^{2}} = \sqrt{a^{2} - b^{2}}$ .
$∴ A B = 2 A C = 2 \sqrt{a^{2} - b^{2}}$ [ $∵ C$ is the midpoint of $A B$ ]
i.e., required length of the chord $A B = 2 \sqrt{a^{2} - b^{2}}$ .

Updated on:21/07/2023

|

Class 10 MATHS CIRCLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Two concentric circles are of radii 13 cm and 5 cm. The length of the chord of the larger circle which touches the smaller circle is:
दो संकेंद्री वृत्तों की त्रिज्या 13 सेमी तथा 5 सेमी है। बड़े वृत्त की जीवा की लंबाई ज्ञात कीजिए, जो छोटे वृत्त को स्पर्श करती है।
A6 cm
B7 cm
C10 cm
D8 cm

RS AGGARWAL-CIRCLES-Test Yourself

Home
Ask Doubt
Profile