[Telugu] Rate of disappearance of A (g) is 5.0xx10^(-2)"mol"t^(-1)

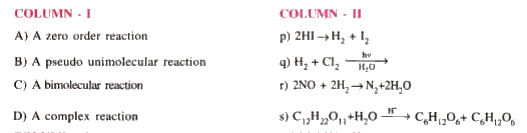Match the following columns ocr_image

In a gaseous phase reaction $A_{2} (g) \to B (t) + 1 (1 / 2) C (g)$ , the increase in pressure from 100 mm to 120 mm is noticed in 5 minute.The rate of disappearance of $A_{2}$ in mm ${min}^{- 1}$ is

View Solution

For the reaction $N_{2} + 3 H_{2} \to 2 N H_{3}$ , the rate $\frac{d [N H_{3}]}{d t} = 2 \times 10^{- 4} M s^{- 1}$ . Therfore the rate $- \frac{d [N_{2}]}{d t}$ is given

View Solution

Discuss on the kinetic spontaneity of a chemical reaction. Is a slow reaction spontaneous? Why?

View Solution

Which among the following is not an exact differential?

View Solution

The rate and mechamical reaction are studied in chemical kinetics. The elementary reactions are single step reaction having no mechanism. The order of reaction and molecularity are same for elementary reactions. The rate of forward reaction $a A + b B \to c C + d D$ is given as:
$r a t e = (\frac{d x}{d t}) = - \frac{1}{a} \frac{d [A]}{d t} = - \frac{1}{b} \frac{d [B]}{d t} = \frac{1}{c} \frac{d [C]}{d t} = \frac{1}{d} \frac{d [D]}{d t}$ or expression can be written as : rate $= K_{1} {[A]}^{a} {[B]}^{b} - K_{2} {[C]}^{c} {[D]}^{d}$ . At equilibrium, rate = $0$ . The constants $K, K_{1}, K_{2}$ are rate constants of respective reaction. In case of reactions governed by two or more steps reaction mechanism, the rate is given by the slowest step of mechanism.
The rate of formation of $S O_{3}$ in the following reaction,
$2 S O_{2} + O_{2} \to 2 S O_{3}$ is $10 g {sec}^{- 1}$
The rate of disappearance of $O_{2}$ will be:

View Solution

The rate and mechamical reaction are studied in chemical kinetics. The elementary reactions are single step reaction having no mechanism. The order of reaction and molecularity are same for elementary reactions. The rate of forward reaction $a A + b B \to c C + d D$ is given as:
$r a t e = (\frac{d x}{d t}) = - \frac{1}{a} \frac{d [A]}{d t} = - \frac{1}{b} \frac{d [B]}{d t} = \frac{1}{c} \frac{d [C]}{d t} = \frac{1}{d} \frac{d [D]}{d t}$ or expression can be written as : rate $= K_{1} {[A]}^{a} {[B]}^{b} - K_{2} {[C]}^{c} {[D]}^{d}$ . At equilibrium, rate = $0$ . The constants $K, K_{1}, K_{2}$ are rate constants of respective reaction. In case of reactions governed by two or more steps reaction mechanism, the rate is given by the slowest step of mechanism.
For the reaction, $a A \to b B$ ,
$log [\frac{- d A}{d t}] = log [\frac{d B}{d t}] + 0.6$ , then $a : b$ is:

View Solution

The rate and mechamical reaction are studied in chemical kinetics. The elementary reactions are single step reaction having no mechanism. The order of reaction and molecularity are same for elementary reactions. The rate of forward reaction $a A + b B \to c C + d D$ is given as:
$r a t e = (\frac{d x}{d t}) = - \frac{1}{a} \frac{d [A]}{d t} = - \frac{1}{b} \frac{d [B]}{d t} = \frac{1}{c} \frac{d [C]}{d t} = \frac{1}{d} \frac{d [D]}{d t}$ or expression can be written as : rate $= K_{1} {[A]}^{a} {[B]}^{b} - K_{2} {[C]}^{c} {[D]}^{d}$ . At equilibrium, rate = $0$ . The constants $K, K_{1}, K_{2}$ are rate constants of respective reaction. In case of reactions governed by two or more steps reaction mechanism, the rate is given by the slowest step of mechanism.
For the reaction, $A + 2 B \Leftrightarrow A B_{2}$ , the rate of forward reaction is $\frac{d x}{d t} = 1 \times 10^{5} [A] {[B]}^{2} - 1 \times 10^{4} [A B_{2}]$ . The rate constants for forward and backward reactions are:

View Solution

The rate and mechamical reaction are studied in chemical kinetics. The elementary reactions are single step reaction having no mechanism. The order of reaction and molecularity are same for elementary reactions. The rate of forward reaction aA + bBrarr cC+dD is given as: rate =((dx)/(dt))=-1/a(d[A])/(dt)=-1/b(d[B])/(dt)=1/c(d[C])/(dt)=1/d(d[D])/(dt) or expression can be written as : rate =K_(1)[A]^(a)[B]^(b)-K_(2)[C]^(c )[D]^(d) . At equilibrium, rate = 0 . The constants K, K_(1), K_(2) are rate constants of respective reaction. In case of reactions governed by two or more steps reaction mechanism, the rate is given by the slowest step of mechanism. At the point of intersection of the two curve shown for the reaction: Ararr nB the concentration of B is given by:

View Solution

The rate and mechamical reaction are studied in chemical kinetics. The elementary reactions are single step reaction having no mechanism. The order of reaction and molecularity are same for elementary reactions. The rate of forward reaction aA + bBrarr cC+dD is given as: rate =((dx)/(dt))=-1/a(d[A])/(dt)=-1/b(d[B])/(dt)=1/c(d[C])/(dt)=1/d(d[D])/(dt) or expression can be written as : rate =K_(1)[A]^(a)[B]^(b)-K_(2)[C]^(c )[D]^(d) . At equilibrium, rate = 0 . The constants K, K_(1), K_(2) are rate constants of respective reaction. In case of reactions governed by two or more steps reaction mechanism, the rate is given by the slowest step of mechanism. For a hypothetical reaction aA+bBrarr Product, the rate law is: rate =K[A]^(x)[B]^(y) , then:

View Solution

The rate and mechamical reaction are studied in chemical kinetics. The elementary reactions are single step reaction having no mechanism. The order of reaction and molecularity are same for elementary reactions. The rate of forward reaction $a A + b B \to c C + d D$ is given as:
$r a t e = (\frac{d x}{d t}) = - \frac{1}{a} \frac{d [A]}{d t} = - \frac{1}{b} \frac{d [B]}{d t} = \frac{1}{c} \frac{d [C]}{d t} = \frac{1}{d} \frac{d [D]}{d t}$ or expression can be written as : rate $= K_{1} {[A]}^{a} {[B]}^{b} - K_{2} {[C]}^{c} {[D]}^{d}$ . At equilibrium, rate = $0$ . The constants $K, K_{1}, K_{2}$ are rate constants of respective reaction. In case of reactions governed by two or more steps reaction mechanism, the rate is given by the slowest step of mechanism.
For a gaseous reaction, the rate is expressed in terms of $\frac{d P}{d t}$ in place of $\frac{d C}{d t}$ or $\frac{d n}{d t}$ where $C$ is concentration, $n$ is number of moles and 'P' is pressure of reactant. The three are related as:

View Solution

In terms of rate constants for forward and backward reactions $(k_{f} and k_{b})$ , equilibrium constant of a reaction is equal to $\dots \dots \dots \dots . .$

View Solution

Statement-1: For the reaction 2A +B to C , the rate of disappearance of A is twice the rate of disappearance of A is twice the rate of disappearance of B. Statement-2: For the reaction : 2A +B to C Rate of reactions is (d[C])/(dt)

View Solution

The instantaneous rate of an elementary chemical reaction aA+bB (hArr)cC+dD can be given by: rate =k_(f)[A]^(a)[B]^(b)-k_(b)[C]^(c)[D]^(d) where k_(f) and k_(b) are rate constants for forward and backward reactions respectively for the reversible reaction if the reaction is an irreversible one , the rate is expressed as rate =k[A]^(a)[B]^(b) where k is rate constant for the given irreversible reaction and (a+b) is the order of reaction it is also evident from the stoichiometry of reaction that rates of disappearance of A is a/b times the rate of disappearance of B. The variation of rate constant k with temperature is expressed in terms of Arrhenius equation: k=Ae^-(E_(a)//RT) whereas the ratio k_(f)/k_(b) is expressed in terms of van't Hoff isochore: K_(f)/K_(b)=Ae^(-DeltaH//RT) where E_(a) and DeltaH are energy of activation and enthalpy of reaction respectively For a chemical reaction : aA rarr bB log[(d[A])/(dt)]=log [(d[B])/(dt)+0.3 Then the ratio of a and b is approximately:

View Solution

The instantaneous rate of an elementary chemical reaction aA+bB (hArr)cC+dD can be given by: rate =k_(f)[A]^(a)[B]^(b)-k_(b)[C]^(c)[D]^(d) where k_(f) and k_(b) are rate constants for forward and backward reactions respectively for the reversible reaction if the reaction is an irreversible one , the rate is expressed as rate =k[A]^(a)[B]^(b) where k is rate constant for the given irreversible reaction and (a+b) is the order of reaction it is also evident from the stoichiometry of reaction that rates of disappearance of A is a/b times the rate of disappearance of B. The variation of rate constant k with temperature is expressed in terms of Arrhenius equation: k=Ae^-(E_(a)//RT) whereas the ratio k_(f)/k_(b) is expressed in terms of van't Hoff isochore: K_(f)/K_(b)=Ae^(-DeltaH//RT) where E_(a) and DeltaH are energy of activation and enthalpy of reaction respectively The variation of K and K_(f)/K_(b) with increase in temperature shows the following effects: (P) For endothermic reaction K increases, K_(f)/K_(b) also increases (Q) For exothermic reaction K decreases K_(f)/K_(b) also decreases (R) For exothermic reaction K and K_(f)/K_(b) both increases (S) For exothermic reaction K increases and K_(f)/K_(b) decreases (T) For exothermic reaction K and K_(f)/K_(b) both decreases.

View Solution

The instantaneous rate of an elementary chemical reaction aA+bB (hArr)cC+dD can be given by: rate =k_(f)[A]^(a)[B]^(b)-k_(b)[C]^(c)[D]^(d) where k_(f) and k_(b) are rate constants for forward and backward reactions respectively for the reversible reaction if the reaction is an irreversible one , the rate is expressed as rate =k[A]^(a)[B]^(b) where k is rate constant for the given irreversible reaction and (a+b) is the order of reaction it is also evident from the stoichiometry of reaction that rates of disappearance of A is a/b times the rate of disappearance of B. The variation of rate constant k with temperature is expressed in terms of Arrhenius equation: k=Ae^-(E_(a)//RT) whereas the ratio k_(f)/k_(b) is expressed in terms of van't Hoff isochore: K_(f)/K_(b)=Ae^(-DeltaH//RT) where E_(a) and DeltaH are energy of activation and enthalpy of reaction respectively For a gaseous phase Ist order reaction A(g) rarr B(g)+2C(g) (rate constant K=10^(-2)time^(-1)) in a closed vessel of 2 litre containing 5 mole of A(g) at 27^(@) C which of the following is incorrect?

View Solution

The correct Answer is:A

$r = \frac{r_{A}}{1} = \frac{r_{B}}{1} = \frac{r_{C}}{2}$

Topper's Solved these Questions

Similar Questions

AAKASH SERIES-CHEMICAL KINETICS-PRACTICE SHEET-1 (LINKED COMPREHENSION TYPE QUESTIONS)

The correct Answer is:A

r = r A 1 = r B 1 = r C 2

Topper's Solved these Questions

Similar Questions

AAKASH SERIES-CHEMICAL KINETICS-PRACTICE SHEET-1 (LINKED COMPREHENSION TYPE QUESTIONS)

$r = \frac{r_{A}}{1} = \frac{r_{B}}{1} = \frac{r_{C}}{2}$