A source of emf having internal resistance of 6Ω dissipates maximum power in a circuit consisting of three resistors R1,R2andR3 as shown. Then

V=24V,I3=0.8A
V=6V,I3=0.4A
V=4V,I3=0.8A
V=24V,I3=0.4A
The correct Answer is:A
According to Kirchhoff.s junction rule
2A=0.8A+0.4A+I3orI3=(2−1.2)A=0.8A
For maximum dissipation of power the external resistance of the circuit must be equal to the internal resistance of the source of emf. Hence, the equivalent resistance is 6Ω. Since the total resistance is 12Ω and the total current is 2 A,
∴V=(12Ω)(2A)=24V
Topper's Solved these Questions
- CURRENT ELECTRICITY BOOK - MTG GUIDECHAPTER - CURRENT ELECTRICITY EXERCISE - CHECK YOUR NEET VITALS
16 Videos
- CURRENT ELECTRICITY BOOK - MTG GUIDECHAPTER - CURRENT ELECTRICITY EXERCISE - MCQs AIPMT / NEET
32 Videos
- CURRENT ELECTRICITY BOOK - MTG GUIDECHAPTER - CURRENT ELECTRICITY EXERCISE - MCQs AIPMT / NEET
32 Videos
- ATOMS AND NUCLEIBOOK - MTG GUIDECHAPTER - ATOMS AND NUCLEIEXERCISE - AIPMT/NEET(MCQs)
40 Videos
- DUAL NATURE OF MATTER AND RADIATION BOOK - MTG GUIDECHAPTER - DUAL NATURE OF MATTER AND RADIATION EXERCISE - AIPMT/ NEET MCQs
30 Videos
Similar Questions
Passage :
A battery EA of 123 volts emf internal resistance 0.4Ω and a battery EB of 117 V emf and internal resistance 0.6Ω supplies a current to load resistance RL through four wires of resistors RA=1.2Ω,RB=1Ω,RC=1.4ΩandRD=1.4Ω as shown in figure.
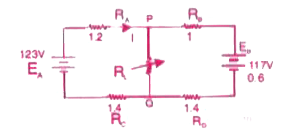
The value of load resistance for maximum load power is
View SolutionTwo sources of equal emf are connected to an external resistance R. The internal resistance of the two sources areR1andR2(R1>R1). If the potential difference across the source having internal resistance R2 is zero, then
View Solution
Knowledge Check
A cell of e.m.f. 2V and negligible internal resistance is connected to resistor R1 and R2 as shown in the figure. The resistance of the Voltmeter R1 and R2 are 80Ω,40Ω and 80Ω respectively. The reading of the voltmeter is:-
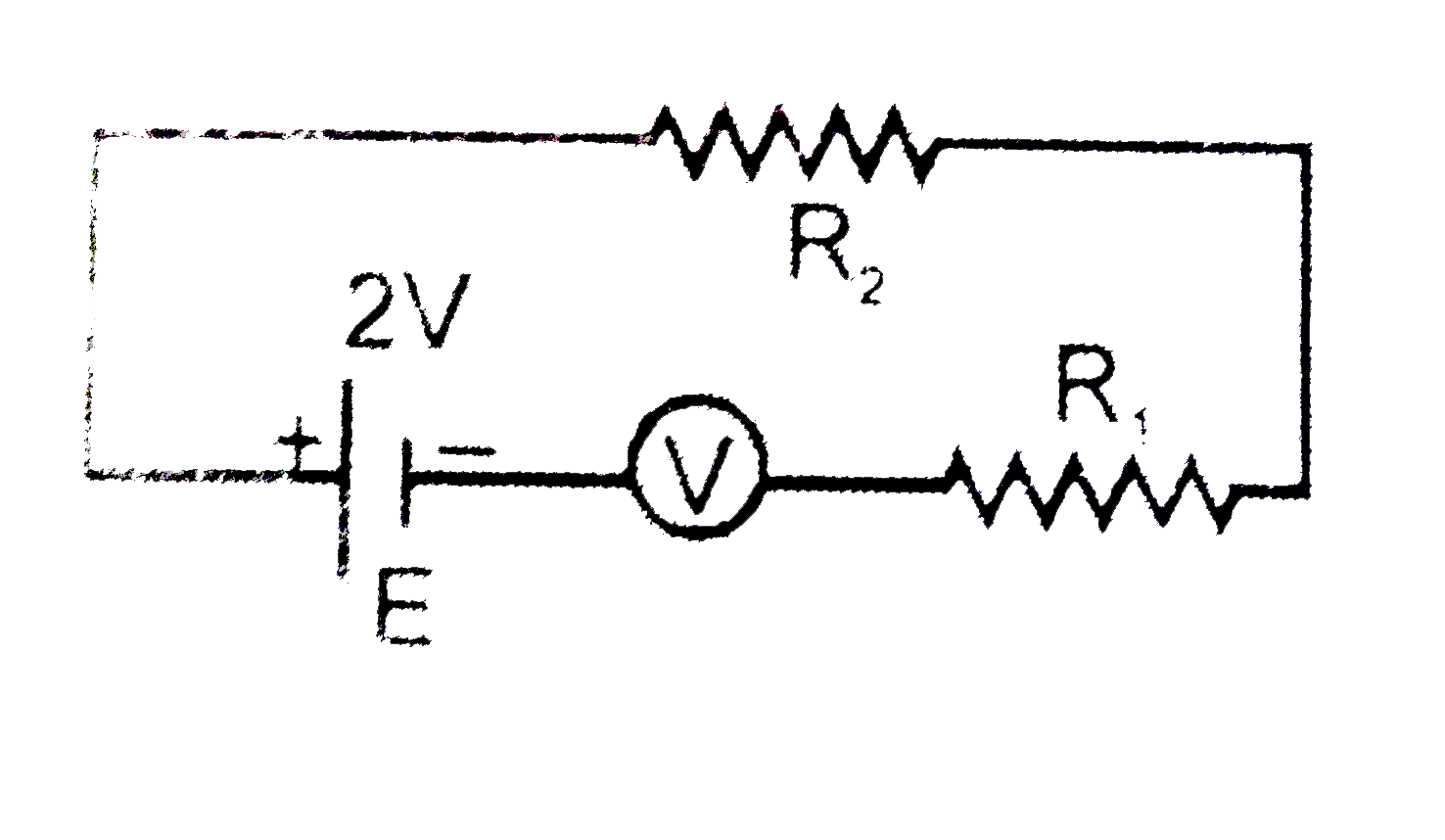 A1.78VB1.60VC0.80VD1.33V
A1.78VB1.60VC0.80VD1.33VTwo sources of emf 6V and internal resistance 3Ω and 2Ω are connected to anexternal resistance R as shown. If potential difference across battery A is zero, then the value of R ( in Ω) is
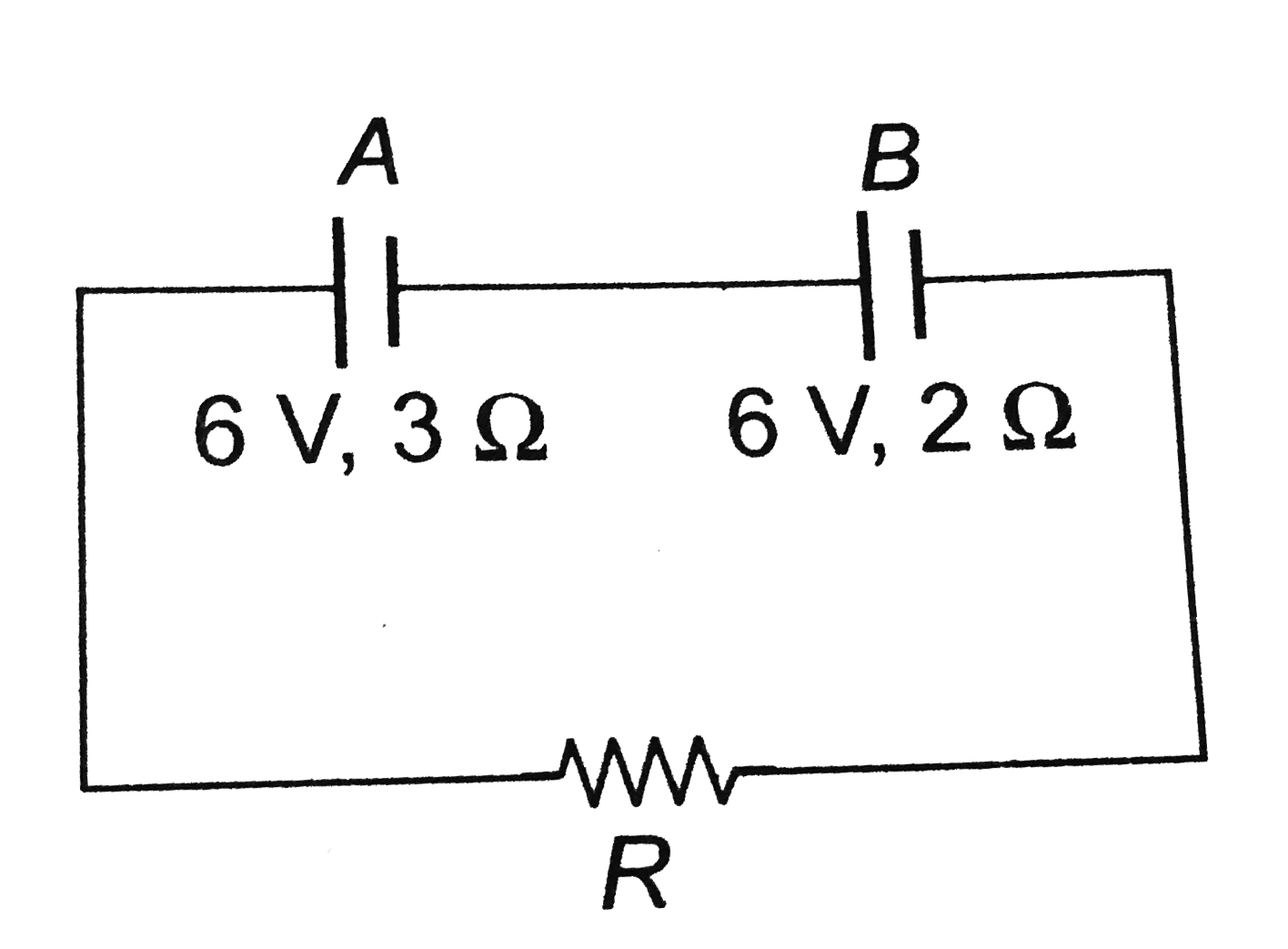 A1ΩB2ΩC3ΩD4Ω
A1ΩB2ΩC3ΩD4ΩThree cells of emf 16V, 2V and 3V and internal resistance 2Ω,1Ω and 1/2Ω respectively are connected with an unknown resistance R as shown in the figure. What is the value of R such that power developed through R is maximum in steady state condition?
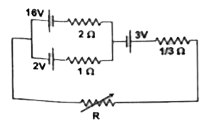 A1/2ΩB1ΩC2ΩDCannot be determined
A1/2ΩB1ΩC2ΩDCannot be determined
Two sources of equal emf are connected to an external resistance R. The internal resistance of the two sources areR1andR2(R1>R1). If the potential difference across the source having internal resistance R2 is zero, then
View SolutionA cell of e.m.f. 2V and negligible internal resistance is connected to resistor R1 and R2 as shown in the figure. The resistance of the Voltmeter R1 and R2 are 80Ω,40Ω and 80Ω respectively. The reading of the voltmeter is:-
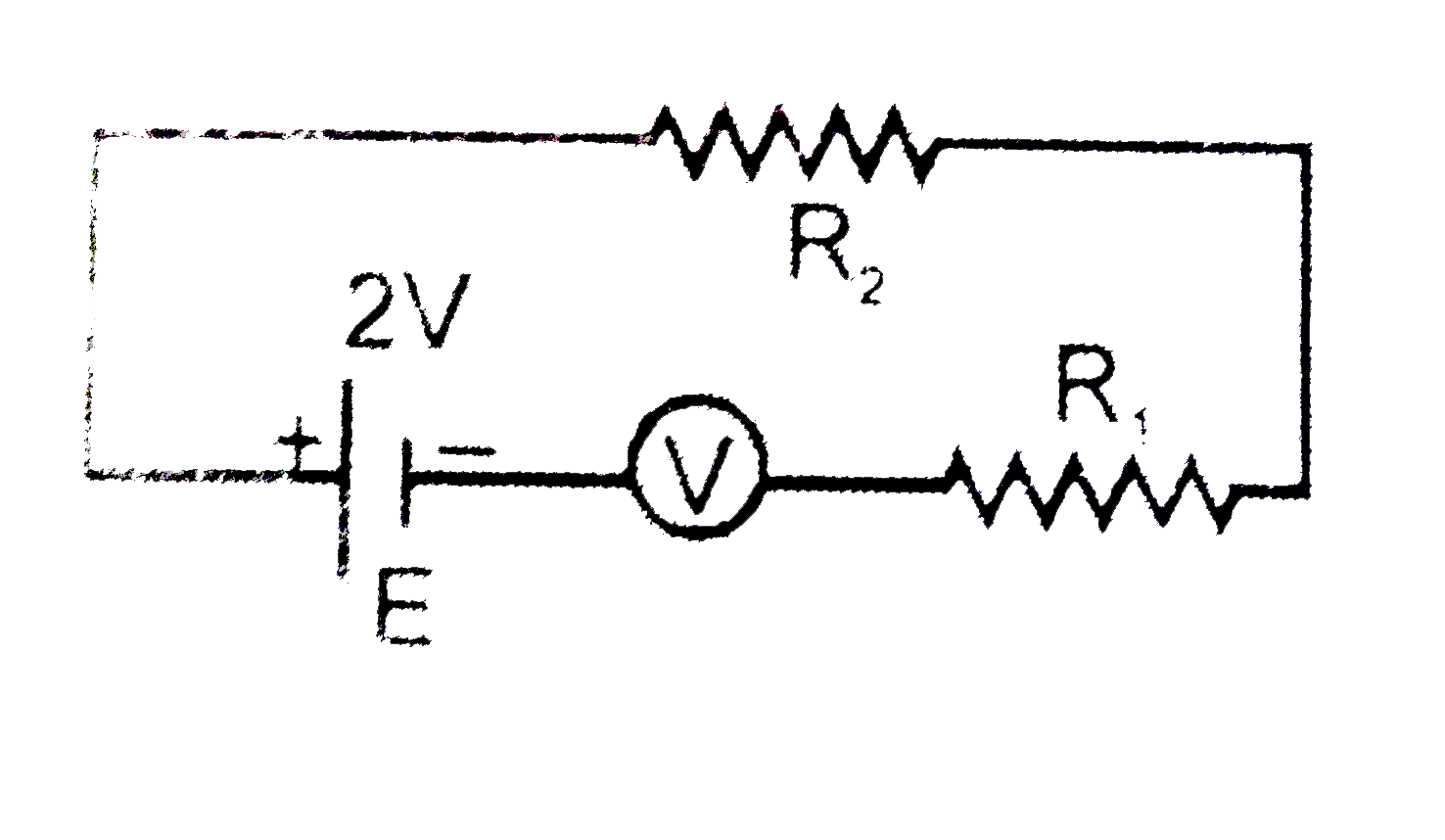
View SolutionThree cells of emf 16V, 2V and 3V and internal resistance 2Ω,1Ω and 1/2Ω respectively are connected with an unknown resistance R as shown in the figure. What is the value of R such that power developed through R is maximum in steady state condition?

View SolutionIn the circuit shown, both cells are ideal and of fixed emf, the resistance of resistance R1 has fixed resistance and the resistance of resistor R2 can be varied (but the value of R2 is not zero). Then:
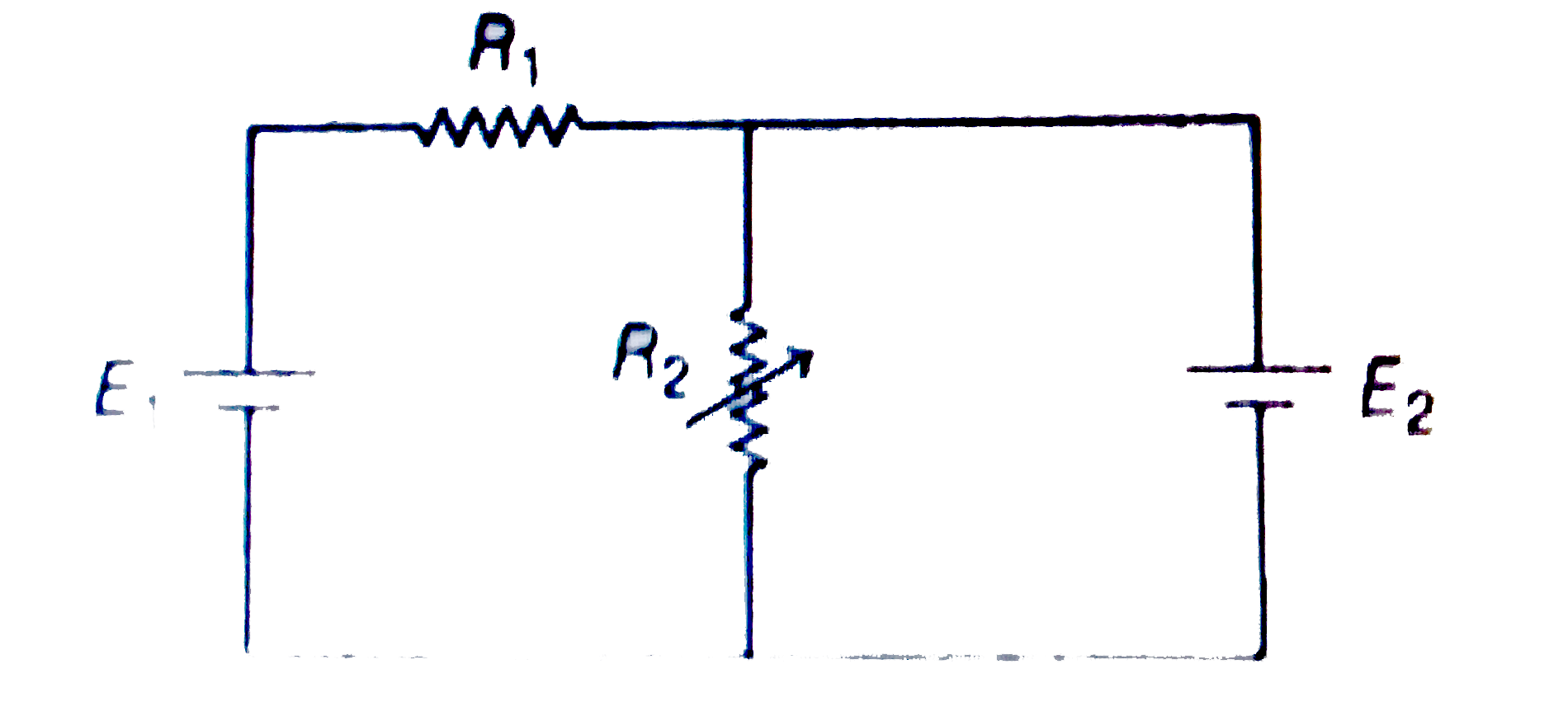
View SolutionIn the circuit shown, both cells are ideal and of fixed emf, the resistance of resistance R1 has fixed resistance and the resistance of resistor R2 can be varied (but the value of R2 is not zero). Then:

View Solution
