A long solenoid of diameter 0.1 m has $2 \times 10^{4}$ turns per meter. At centre of the solenoid is 100 turns coil of radius 0.01 m placed with its axis coinciding with solenoid axis. The current in the solenoid reduce at a constant rate to 0A from 4 a in 0.05 s . If the resistance of the coil is $10 π^{2} Ω$ , the total charge flowing through the coil during this time is

A

$16 μ C$

B

$32 μ C$

C

$16 π μ C$

D

$32 π μ C$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Given $n = 2 \times 10^{4}, I = 4 A$
Initial I = 0A
$∴ B_{i} = 0 or ϕ_{i} = 0$
Finally , the magnetic field at the centre of the solenoid is given as
$B_{f} = μ_{0} n I = 4 π \times 10^{- 7} \times 2 \times 10^{4} \times 4 = 32 π \times 10^{- 3} T$
Finl magnetic flux through the coil is given as
$ϕ_{f} = N B A = 100 \times 32 π \times 10^{- 3} \times π \times {(0.01)}^{2}$
$ϕ_{f} = 32 π^{2} \times 10^{- 5} T m^{2}$
Induced charge $q = \frac{| Δ ϕ |}{R} = \frac{∣ ∣ ϕ_{f} - ϕ_{i} ∣ ∣}{R} = \frac{32 π^{2} \times 10^{- 5}}{10 π^{2}}$
$= 32 \times 10^{- 6} C = 32 μ C$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A long solenoid of diameter 0.1 m has $2 \times 10^{4}$ turns per meter. At centre of the solenoid is 100 turns coil of radius 0.01 m placed with its axis coinciding with solenoid axis. The current in the solenoid reduce at a constant rate to 0A from 4 a in 0.05 s . If the resistance of the coil is $10 π^{2} Ω$ , the total charge flowing through the coil during this time is
A $16 μ C$
B $32 μ C$
C $15 π μ C$
D $32 π μ C$
Question 2 - Select One
A 0.100 m long solenoid has a radius of 0.050 m and $1.50 \times 10^{4}$ turns. The current in the solenoid changes at a rate of 6.0 A/s. A conducting loop of radius 0.0200 m is placed at the center of the solenoid with its axis the same as that of the solenoid as shown.

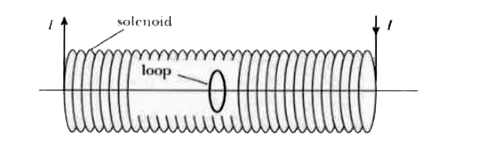
Determine the induced emf in the loop.
A $0.7 \times 10^{- 3}$ V
B $2.8 \times 10^{- 3}$ V
C $1.4 \times 10^{- 3}$ V
D $5.6 \times 10^{- 3}$ V

MTG GUIDE-ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS-AIPMT/NEET MCQS

Home
Ask Doubt
Profile