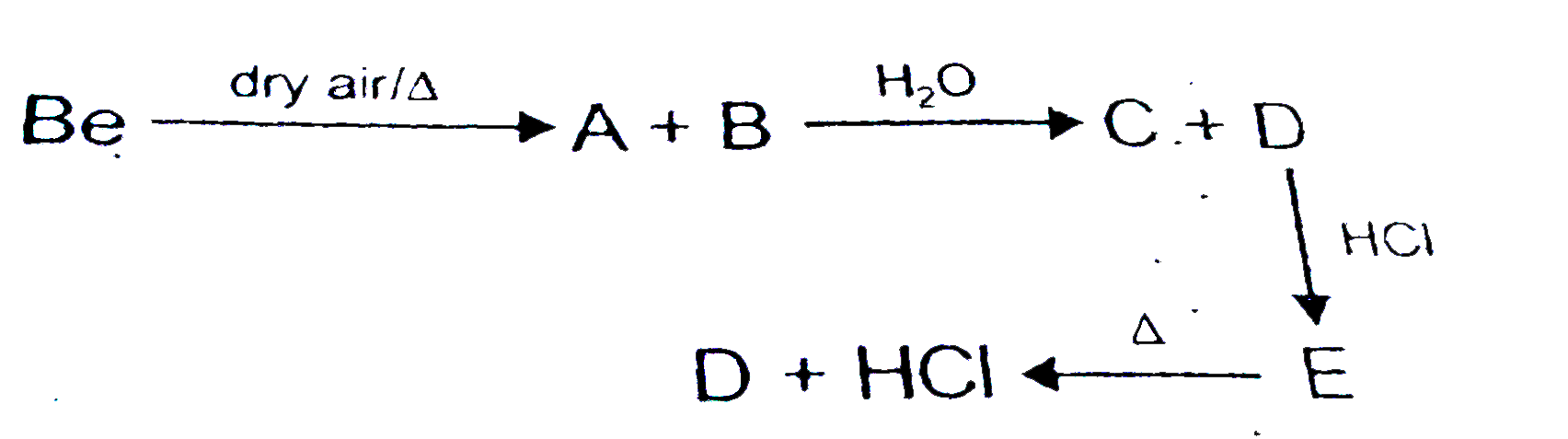
Q. A & B are
BeO&Be3N2
BeO&BeO2
BeO&Be(OH)2
Be3N2&Be(OH)2
The correct Answer is:A
Step by step video & image solution for Q. A & B are by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- THE S-BLOCK ELEMENTSBOOK - AAKASH INSTITUTECHAPTER - THE S-BLOCK ELEMENTSEXERCISE - Assignment (Section-E)
7 Videos
- THE S-BLOCK ELEMENTSBOOK - AAKASH INSTITUTECHAPTER - THE S-BLOCK ELEMENTSEXERCISE - Assignment (Section-F)
3 Videos
- THE S-BLOCK ELEMENTSBOOK - AAKASH INSTITUTECHAPTER - THE S-BLOCK ELEMENTSEXERCISE - Assignment (Section-C)
11 Videos
- THE P-BLOCK ELEMENTSBOOK - AAKASH INSTITUTECHAPTER - THE P-BLOCK ELEMENTSEXERCISE - Assignment Section-J)
16 Videos
- THE SOLID STATE BOOK - AAKASH INSTITUTECHAPTER - THE SOLID STATE EXERCISE - Assignment (SECTION - D) (ASSERTION-REASON TYPE QUESTION)
20 Videos
Similar Questions
If A,B,C are three real numbers and p=[A+b+C] and q=[A]+[B]+[C]. (where [.] represents greatest integer function). Then maximum value of p−q is: 1 (b) 0 (c) 2 (d) 3
View Solution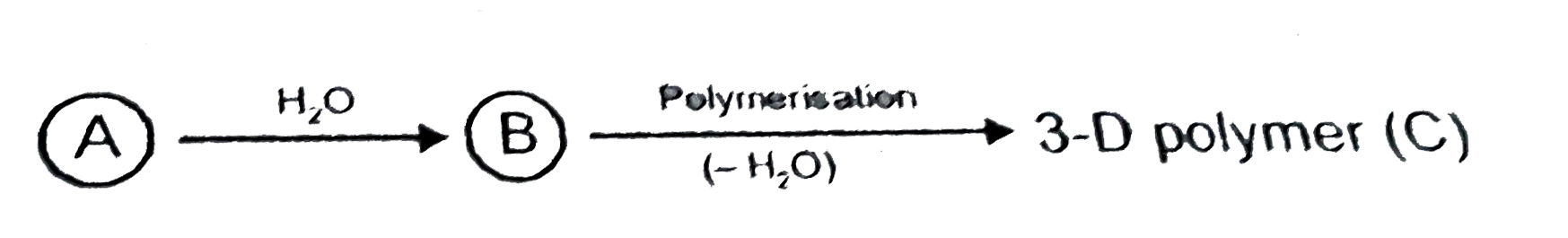
If (C) is silicone, then answer the following questions.
Q. (A) and (B) are
View SolutionIn Figure, line l is the bisector of angle A and B is any point on l . BP and BQ are perpendiculars from B to the arms of A. Show that : APB≅AQB BP=BQ or B is equidistant from the arms of ∠A . Figure
View SolutionIf AB is a chord of a circle, Pand Q are the two points on the circle different from A and B, then ∠APB= ∠AQB ∠APB+∠AQB=180∘ or∠APB= ∠AQB
View SolutionIf pa+qb+rc=1 & ap+bq+cr=0 find p2a2+q2b2+r2c2= ?
View SolutionIf ABC≅PQR and ABC is not congruent to RPQ, then which of the following not true: BC=PQ (b) AC=PR (c) AB=PQ (D) QR=BC
View SolutionIf X={S,T,U,V} and Y = {s,t,u,v}, then P and Q are _______ sets. (equal/equivalent)
View SolutionIf P={A,B,C,D} and Q={a,b,c,d} then P and Q are ______ set (equal/equivalent)
View SolutionThree discs, A, B and C having radii 2m, 4m and6m respectively are coated with carbon black on their outer surfaces. The wavelengths corresponding to maximum intensity are 300nm,400nm and 500nm, respectively. The power radiated by them are QA,QBandQC respectively
(a) QA is maximum (b) QB is maximum (c) QC is maximum (d) QA=QB=QC
View SolutionABCD and APQR are two parallelograms. Prove that ∠C=∠Qand∠B=∠R
View Solution
