Question

Show that the oscillations due to a spring are simple harmonic oscillations and obtain the expression of periodic time.

Explanation

According to figure a block of mass m fixed to a spring, which in turn is fixed to a rigid wall.

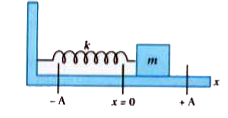
The block is placed on a friction less horizontal surface.
If the block is pulled on one side and is released it then executs a to and fro motion about a mean position.
Let x=0, indicate the position of the centre of the block when the spring is in equilibrium.
the positions -A and +A indicate the maximum displacement to the left and the right of the mean position.
For spring Robert Hooke law, ..Spring when deformed, is subject to a restoring force, the magnitude of which is proportional to the deformation or the displacement and acts is opposite direction...
Let any time t, if the displacement of the block form its mean position is x, the restoring force F acting on the block it
`F(x) = -kx" ""............"(1)`
Where k is constant of proportionality and is called the spring constant or spring force constant.
Equation (1) is same as the force law for SHM and therefore the system executes a simple hormonic motion.
But `F(x) = ma(x)`
`therefore ma(x) = kx`
`therefore m (-omega^(2)x)= -kx " "[therefore a(x)= -omega^(2) x]`
`therefore omega = sqrt((k)/(m))`
`therefore (2pi)/(T)= sqrt((k)/(m))`
`therefore T = 2pi sqrt((k)/(m))`
is the period of block attached with spring having small oscillations
`therefore T propto (1)/(sqrt(k))` (for a given block) and `T propto sqrt(m)` (for a given spring) and frequency `v= (1)/(T) = (1)/(2pi) sqrt((m)/(k))`
Stiff spring have high value of k and period is small and frequency of oscillation is large. So the oscillation becomes rapid and for soft spring k is small and hence period is large and frequency of oscillation is small so oscillation becomes slowly.