When a mass m is connected individually to two springs S1 and S2 the oscillation frequencies are v1 and v2. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be
 Solution in Gujrati
Solution in Gujrati
v1+v2
√v21+v22
(1v1+1v2)−1
√v21−v22
The correct Answer is:B

The block is considered with two springs considered as parallel. Hence equivalent spring constant k=k1+k2 where k1 and k2 are spring constant of spring S1 and S2 respectively.

Time period of oscillation of the spring block system is
T=2π√mk=2π√m(k1)+(k2)
where k = equivalent spring constant
Frequency of the system,
v=12π√k1+k2m ........(1)
Frequency of oscillation of a body of mass m with spring S1 is
v1=12π√k1m .........(2)
and frequency of oscillation of this body with sprin S2 is
v2=12π√k2m .........(3)
From equation (2),
k1m=4π2v1 .........(4)
and from equation (3),
k2m=4π2v2 .........(5)
From equation (1),
v=12π[k1m+k2m]12
v=12π[4π2v21+4π2v2]12 [∴ From equations (4) and (5)]
∴v=2π2π[v21+v22]12
∴v=√v21+v22.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - OSCILLATIONSEXERCISE - SECTION-D (NCERT EXEMPLAR SOLUTION VERY SHORT ANSWER QUESTIONS)10videos
OSCILLATIONS
- BOOK - KUMAR PRAKASHANCHAPTER - OSCILLATIONSEXERCISE - SECTION-D (NCERT EXEMPLAR SOLUTION SHORT ANSWER QUESTIONS)6videos
OSCILLATIONS
- BOOK - KUMAR PRAKASHANCHAPTER - OSCILLATIONSEXERCISE - SECTION-D (NCERT EXEMPLAR SOLUTION MCQs)10videos
OSCILLATIONS
- BOOK - KUMAR PRAKASHANCHAPTER - OBJECTIVE QUESTIONS AS PER NEW PAPER STYLE EXERCISE - CHAPTER - 8 (Match Type questions)5videos
OBJECTIVE QUESTIONS AS PER NEW PAPER STYLE
- BOOK - KUMAR PRAKASHANCHAPTER - PHYSICAL WORLDEXERCISE - SECTION-E (QUESTIONS FROM MODULE)9videos
PHYSICAL WORLD
Similar Questions
Displacement versus time curve for a particle executing SHM is shown in figure. Idendify the points marked at which (i) velocity of the oscillator is zero, (ii) speed of the oscillator is maximum.
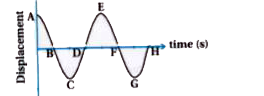 02:24View Solution
02:24View SolutionFind the time period of mass M when displaced from its equilibrium position and then released for the system shown in figure.
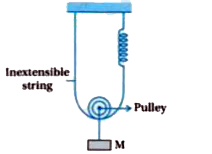 .02:07View Solution
.02:07View SolutionThe displacement of a particle represented by the equation y=3cos(π4−2ωt). The motion of the particle is
02:23View SolutionType of satellites are given in Column-I and their uses are given in Column-II. Match them properly :
Column-IColumn-II(1) Polar satellite(a) Tele communication(2) Geo-stationary satellite(b) Investigation(c) For information about atmosphere01:50View Solution.…. state of substance at very high temperature has generated hope of energy source for mankind.
02:29View SolutionIn figure S_(1) and S_(1) are identical springs. The oscillation frequency of the mass m is f . if one spring is removed, the frequency will become
03:22View SolutionThe frequency of oscillation of a mass m suspended by a spring is v_(1) . If the length of the spring is cut to on-half, the same mass oscillates with frequency v_(2) . Determing the value of v_(2)//v_(1) .
02:38View SolutionThe frequency of oscillations of a mass m suspended by spring of v1. If the length of the spring is cut to one-third, the same mass oscillates with frequency v2. Determing the value of v2/v1
03:35View SolutionWhen a mass m is connected individually to two spring S1 and S2 , the oscillation frequencies are v1andv2. If the same mass is attached to the two springs as shwon in figure., the oscillation frequecy would be
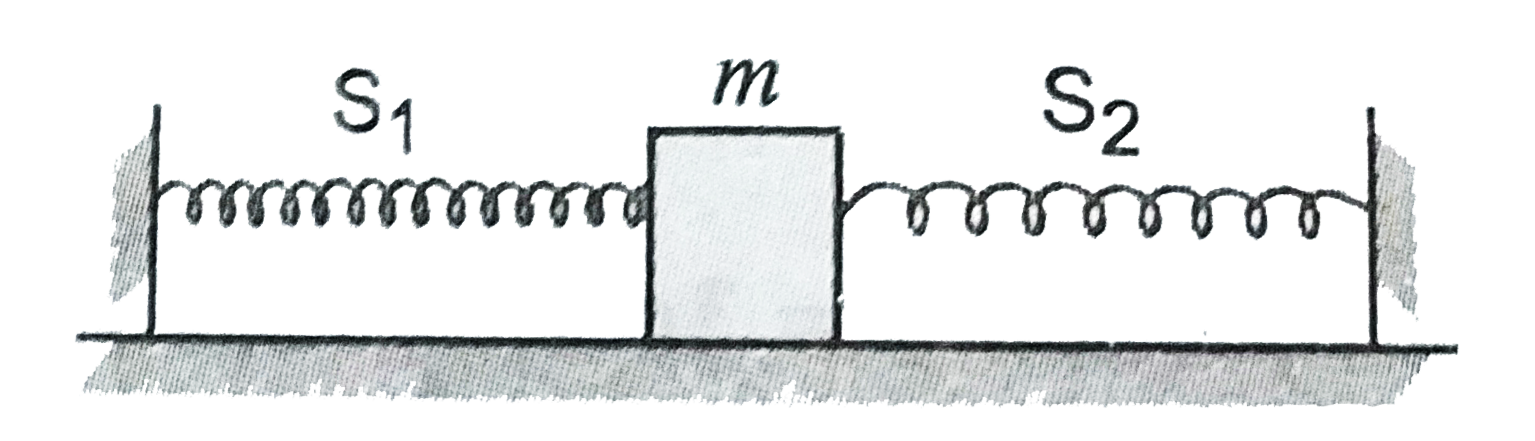 04:36View Solution
04:36View SolutionThe frequency of oscillations of a mass m suspended by a spring is v_(1) . If the length of the spring is cut to one-half the same mass oscillates with frequency v_(2) . Determine the value of v_(2)//v_(1)
02:23View SolutionWhen a mass m is connected individually to two springs S1 and S2, the oscillation frequencies are v1 and v2. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be
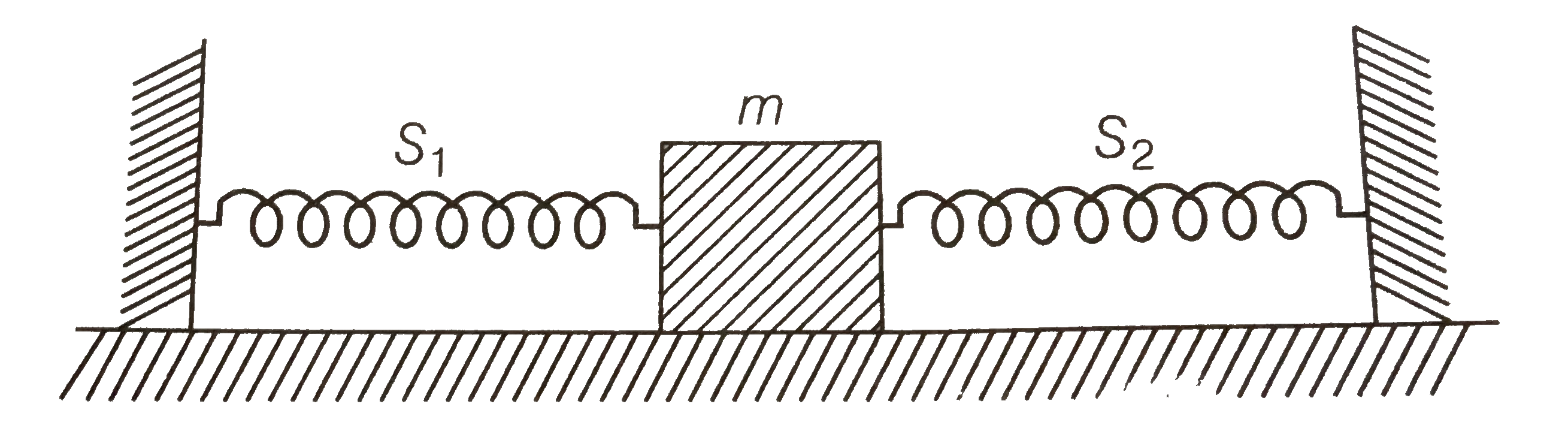 02:46View Solution
02:46View SolutionThe frequency of oscillation of amass m suspended by a spring is v1. If length of spring is cut to one third then the same mass oscillations with frequency v2. Then
02:49View Solution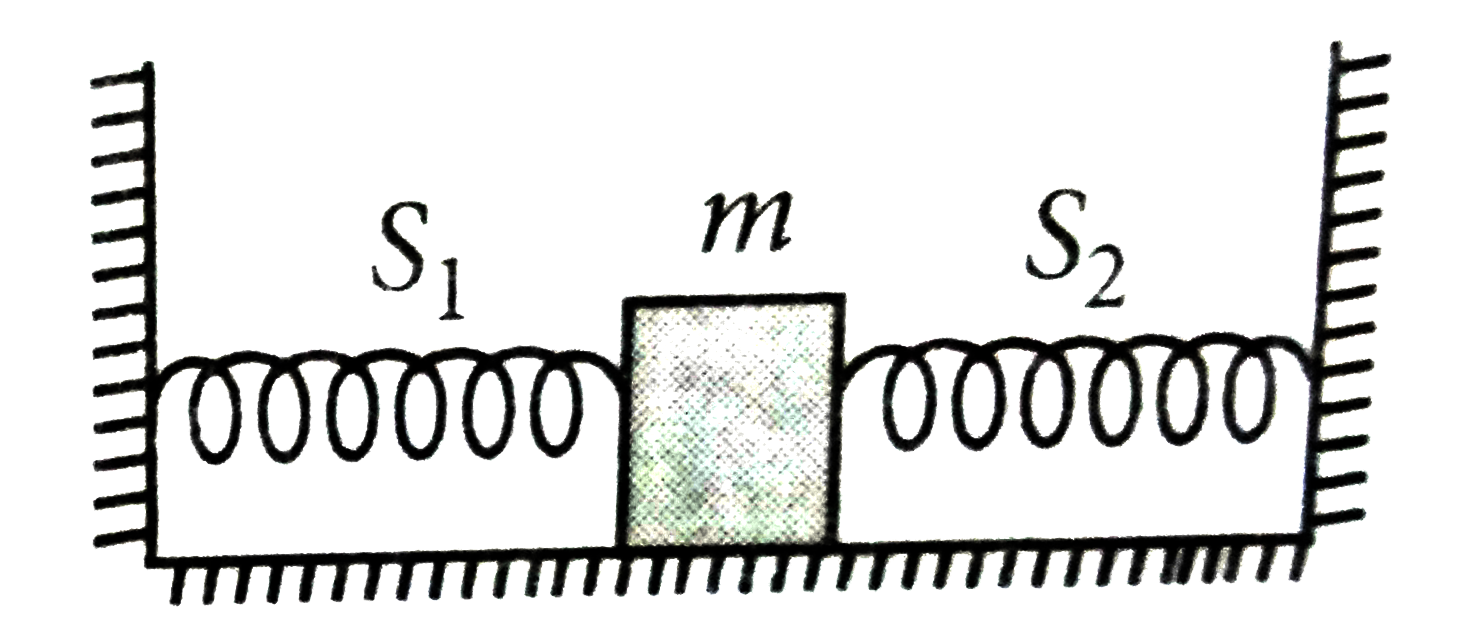
When a mass m is connected individually to two springs S1andS2, the oscillation frequencies are υ1andυ2. If the same mass is attached to the two springs as shown in figure, the oscillation frequency would be04:33View SolutionTwo identical springs S_(1) and S_(2) are joined as shown in the figure. The oscillation frequency of the mass m is v. What will be the frequency of mass m, if one spring is removed ?
01:22View SolutionThe frequency of oscillations of a mass m suspended by spring is v_(1) . If the length of the spring is cut to one- third, the same mass oscillates with frequency v_(2) . Determine the value of (v_(2))/(v_(1)) .
03:36View Solution

