A cricket ball of mass 150g moving with a speed of 126km/h hits at the middle of the bat, held firmly at its position by the batman. The ball moves straight back to the bowler after hitting the bat. Assuming that collision between ball and bat is completely elastic and the two remain in contact for 0.001s, the force that the batsman had to apply to hold the bat firmly at its place would be
10.5N
21N
1.05×104N
2.1×104N
To solve the problem step by step, follow these instructions:
Step 1: Convert the mass of the cricket ball from grams to kilograms.
Given:
- Mass of the cricket ball = 150 g
To convert grams to kilograms:
Mass in kg=150 g1000=0.150 kg
Step 2: Convert the speed of the cricket ball from km/h to m/s.
Given:
- Speed of the cricket ball = 126 km/h
To convert km/h to m/s:
Speed in m/s=126×1000 m1 km×1 h3600 s=35 m/s
Step 3: Determine the final velocity of the ball after it hits the bat.
Since the collision is completely elastic and the ball moves straight back to the bowler, the final velocity will be the negative of the initial velocity:
Final velocity(v)=−35 m/s
Step 4: Calculate the change in momentum (ΔP) of the ball.
The change in momentum is calculated using the formula:
ΔP=m(v−u)
Where:
- m=0.150 kg
- u=35 m/s (initial velocity)
- v=−35 m/s (final velocity)
Substituting the values:
ΔP=0.150×(−35−35)=0.150×(−70)=−10.5 kg m/s
Step 5: Calculate the force (F) applied by the batsman.
Using the formula:
F=ΔPΔt
Where:
- Δt=0.001 s
Substituting the values:
F=−10.50.001=−10500 N
Step 6: Interpret the result.
The negative sign indicates that the force exerted by the batsman is in the opposite direction to the initial motion of the ball. Therefore, the magnitude of the force that the batsman had to apply to hold the bat firmly at its place is:
F=1.05×104 N
Final Answer:
The force that the batsman had to apply to hold the bat firmly at its place is 1.05×104 N.
---
Given:
- Mass of the cricket ball = 150 g
Mass in kg=150 g1000=0.150 kg
Given:
- Speed of the cricket ball = 126 km/h
Speed in m/s=126×1000 m1 km×1 h3600 s=35 m/s
Since the collision is completely elastic and the ball moves straight back to the bowler, the final velocity will be the negative of the initial velocity:
Final velocity(v)=−35 m/s
The change in momentum is calculated using the formula:
ΔP=m(v−u)
Where:
- m=0.150 kg
- u=35 m/s (initial velocity)
- v=−35 m/s (final velocity)
ΔP=0.150×(−35−35)=0.150×(−70)=−10.5 kg m/s
Using the formula:
F=ΔPΔt
Where:
- Δt=0.001 s
F=−10.50.001=−10500 N
The negative sign indicates that the force exerted by the batsman is in the opposite direction to the initial motion of the ball. Therefore, the magnitude of the force that the batsman had to apply to hold the bat firmly at its place is:
F=1.05×104 N
The force that the batsman had to apply to hold the bat firmly at its place is 1.05×104 N.
Similar Questions
A cricket ball of mass 100 g moving with a speed of 30ms−1 is brought to rest by a player in 0.03 s. Find the change in momentum of ball.
View SolutionA cricket ball of mass 150 g moving at a speed of 25 ms−1 is brought to rest by a player in 0.03 s. Find the average force applied by the player.
View SolutionA cricket ball of mass 150 g is moving with a speed of 12ms−1 and is hit by a bat so that the ball is turned back with velocity of 20ms−1 if duration of contact between bat and ball is 0.01 s then:
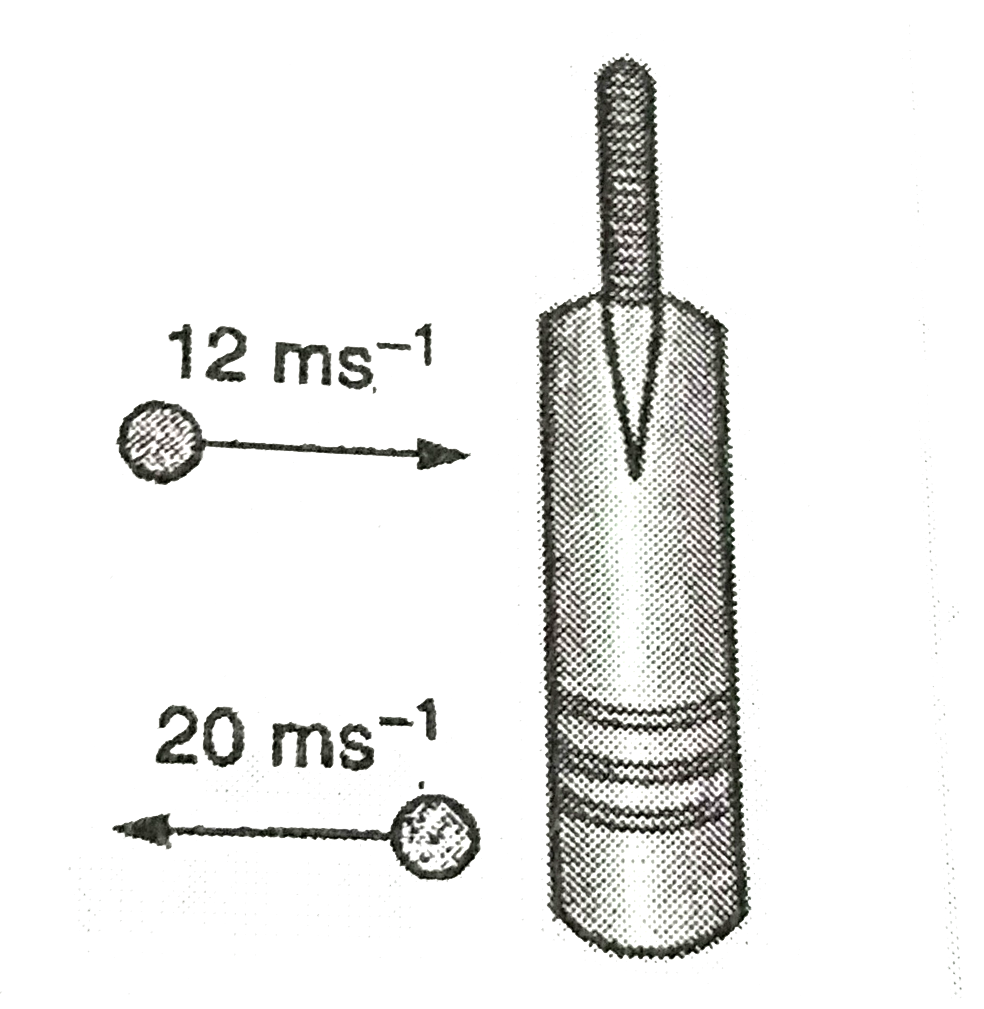
View SolutionA cricket ball of mass 150 kg is moving with a velocity of 12m/s and is hit by a bat so that ball is turned back with a velocity of 20m/s . The force of the blow acts for 0.01 s on the ball . Find the average force exerted by the bat on the ball.
View SolutionA player caught a cricket ball of mass 150g moving at a rate of 20m/s. If the catching process is completed in 0.1s, the force of the blow exerted by the ball on the hand of the player is equal to
View SolutionA metal ball of mass 2 kg moving with a velocity of 36km/h has a head on collision with a stationery ball of mass 3 kg. If after the collision, the two balls move together, the loss in kinetic energy dur to collision is
View SolutionA cricket baal of mass 0.25 kg with speed 10 m/s collides with a bat and returns with same speed with in 0.01 s. The force acted on bat is
View SolutionA ball of mass 150 g moving with an acceleration 20 m/s2 is hit by a force, which acts on it for 0.1 s. The impulsive force is
View SolutionA ball of mass 0⋅2 kg moving with a speed of 30 m/s strikes a bat returns in opposite direction with speed of 90m/s . Magnitude of change in momentu,m of ball will be
View SolutionA ball of mass 0.50 kg moving at a speed of 5.0 m/s collides with another ball of mas 1.0 kg. After the collision the balls stick together ane remain motionless. What was the velocity of the 1.0 kg block before the collision?
View Solution
