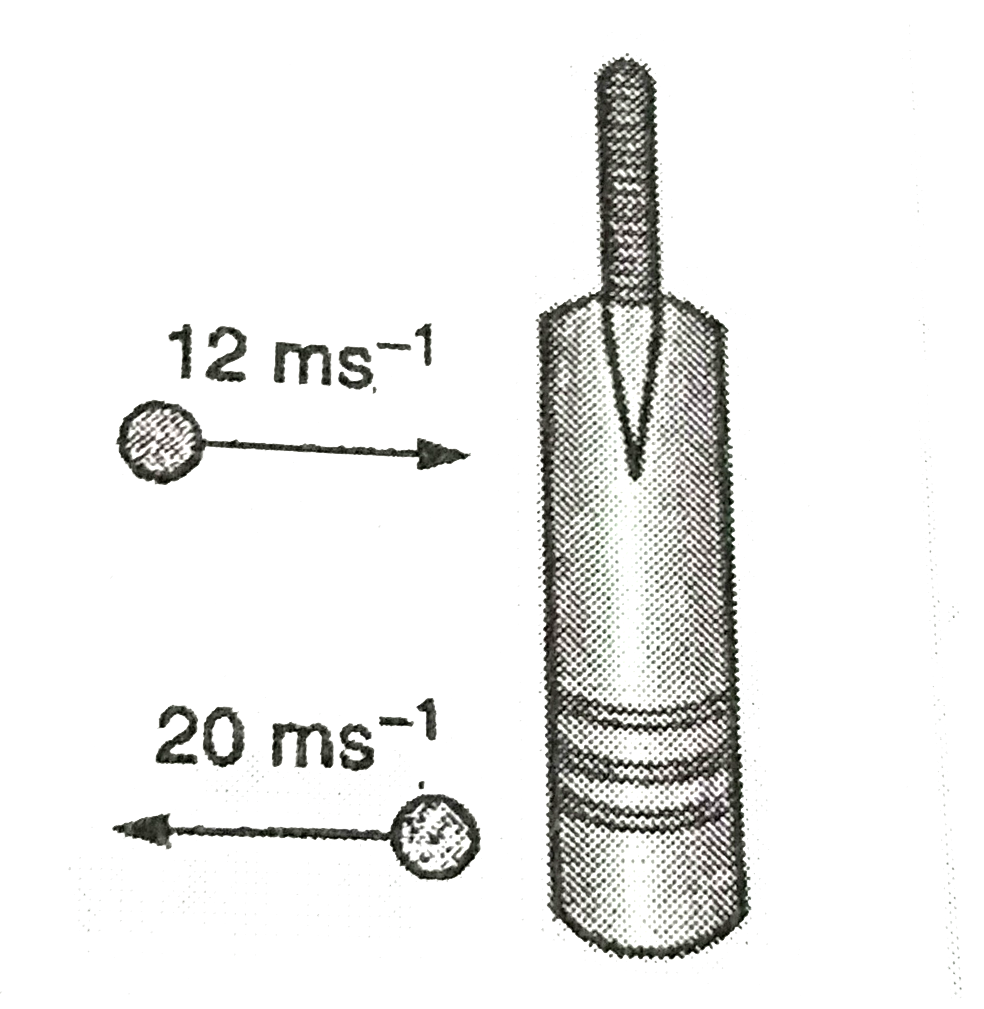
A cricket ball of mass 150 kg is moving with a velocity of 12m/s and is hit by a bat so that ball is turned back with a velocity of 20m/s . The force of the blow acts for 0.01 s on the ball . Find the average force exerted by the bat on the ball.
the change in momentum of the ball is 48 kg-m/s.
the average force exerted by the bat on the ball is 480 N.
the change in momentum of the ball is 1.2 kg-m/s
the average force exerted by the bat on the ball is 120 N.
The correct Answer is:A, B
To solve the problem, we need to find the average force exerted by the bat on the cricket ball. We will use the concept of momentum and the formula for average force.
1. Identify the Given Data:
- Mass of the cricket ball, m=150grams=0.15kg (since 1 kg = 1000 grams)
- Initial velocity of the ball, u=12m/s
- Final velocity of the ball after being hit, v=−20m/s (the negative sign indicates that the ball is moving in the opposite direction)
- Time duration of the force acting on the ball, Δt=0.01s
2. Calculate the Change in Momentum:
- The change in momentum (Δp) is given by the formula:
Δp=m(v−u)
- Substituting the values:
Δp=0.15kg×(−20m/s−12m/s)=0.15kg×(−32m/s)
- This simplifies to:
Δp=0.15×−32=−4.8kg m/s
3. Calculate the Average Force:
- The average force (F) can be calculated using the formula:
F=ΔpΔt
- Substituting the values:
F=−4.8kg m/s0.01s=−480N
- The negative sign indicates the direction of the force is opposite to the initial motion of the ball. Therefore, the magnitude of the average force is:
|F|=480N
4. Conclusion:
- The average force exerted by the bat on the ball is 480N.
- Mass of the cricket ball, m=150grams=0.15kg (since 1 kg = 1000 grams)
- Initial velocity of the ball, u=12m/s
- Final velocity of the ball after being hit, v=−20m/s (the negative sign indicates that the ball is moving in the opposite direction)
- Time duration of the force acting on the ball, Δt=0.01s
- The change in momentum (Δp) is given by the formula:
Δp=m(v−u)
- Substituting the values:
Δp=0.15kg×(−20m/s−12m/s)=0.15kg×(−32m/s)
- This simplifies to:
Δp=0.15×−32=−4.8kg m/s
- The average force (F) can be calculated using the formula:
F=ΔpΔt
- Substituting the values:
F=−4.8kg m/s0.01s=−480N
- The negative sign indicates the direction of the force is opposite to the initial motion of the ball. Therefore, the magnitude of the average force is:
|F|=480N
- The average force exerted by the bat on the ball is 480N.
Similar Questions
A cricket ball of mass 150 g is moving with a speed of 12ms−1 and is hit by a bat so that the ball is turned back with velocity of 20ms−1 if duration of contact between bat and ball is 0.01 s then:
View SolutionA ball of mass 0.2 kg moves with a velocity of 20m/sec and it stops in 0.1 sec , then the force on the ball is
View SolutionA ball of mass 0.2 kg moves with a velocity of 20m/sec and it stops in 0.1 sec , then the force on the ball is
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA ball of mass 10 g is moving with a velocity of 50ms−1. On applying a constant force on ball for 2.0 s, it acquires a velocity of 70ms−1. Calculate the acceleration of ball
View SolutionA ball of mass 10 g is moving with a velocity of 50ms−1. On applying a constant force on ball for 2.0 s, it acquires a velocity of 70ms−1. Calculate the final momentum of ball
View SolutionA ball of mass 10 g is moving with a velocity of 50ms−1. On applying a constant force on ball for 2.0 s, it acquires a velocity of 70ms−1. Calculate the rate of change of momentum
View SolutionA 150 g ball, moving horizontally at 20m/s was hit straight back to bowler at 12m/s. If contact with bat lasted for 0.04 sec, then average force exerted by the bat on the ball is:-
View SolutionA 150 g ball, moving horizontally at 20 m/s was hit straight back to bowler at 35 m/s. If contact with bat lasted for 0.04sec, than average force exerted by the bat on the ball is:

View Solution