The temperature of a wire is doubled. The Young's modulus of elasticity
will also double
will become four times
will remain same
will decrease
The correct Answer is:D
To solve the problem regarding the effect of temperature on the Young's modulus of elasticity of a wire, we can follow these steps:
1. Understanding Young's Modulus:
Young's modulus (Y) is defined as the ratio of normal stress to longitudinal strain. Mathematically, it can be expressed as:
Y=Normal StressLongitudinal Strain=F/AΔL/L
where:
- F is the force applied,
- A is the cross-sectional area,
- ΔL is the change in length,
- L is the original length.
2. Relating Strain to Temperature Change:
The longitudinal strain (ΔL/L) due to a change in temperature can be expressed using the coefficient of linear expansion (α):
ΔLL=αΔT
where:
- ΔT is the change in temperature.
3. Applying the Given Condition:
In this problem, the temperature of the wire is doubled. If we assume the initial temperature change is ΔT, then doubling the temperature means:
ΔT=2T
Therefore, substituting this into the strain equation gives:
ΔLL=α(2T)=2αT
4. Substituting into Young's Modulus:
Now, substituting the expression for strain back into the equation for Young's modulus:
Y=F/AΔL/L=F/A2αT
This shows that Young's modulus is inversely proportional to the temperature change.
5. Conclusion:
Since Young's modulus is inversely proportional to the temperature change, if the temperature is doubled, the Young's modulus will be halved:
Y′=Y2
where Y′ is the new Young's modulus after the temperature change.
Final Answer:
When the temperature of the wire is doubled, the Young's modulus of elasticity becomes half of its original value.
---
Young's modulus (Y) is defined as the ratio of normal stress to longitudinal strain. Mathematically, it can be expressed as:
Y=Normal StressLongitudinal Strain=F/AΔL/L
where:
- F is the force applied,
- A is the cross-sectional area,
- ΔL is the change in length,
- L is the original length.
The longitudinal strain (ΔL/L) due to a change in temperature can be expressed using the coefficient of linear expansion (α):
ΔLL=αΔT
where:
- ΔT is the change in temperature.
In this problem, the temperature of the wire is doubled. If we assume the initial temperature change is ΔT, then doubling the temperature means:
ΔT=2T
Therefore, substituting this into the strain equation gives:
ΔLL=α(2T)=2αT
Now, substituting the expression for strain back into the equation for Young's modulus:
Y=F/AΔL/L=F/A2αT
This shows that Young's modulus is inversely proportional to the temperature change.
Since Young's modulus is inversely proportional to the temperature change, if the temperature is doubled, the Young's modulus will be halved:
Y′=Y2
where Y′ is the new Young's modulus after the temperature change.
When the temperature of the wire is doubled, the Young's modulus of elasticity becomes half of its original value.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LONG SHORT ANSWER TYPE QUESTION16videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Long Answer Type Questions3videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - Multiple Choice Questions37videos
MOTION IN A PLANE
Similar Questions
Assertion: From the relation Y =FlAΔl, we can say that, if length of a wire is doubled, its young's modulus of elasticity will also becomes two times.
Reason : Modulus of elasticity is a material property.View Solutionisobaric modulus of elasticity is
View SolutionAssertion If length of a wire is halved, its Young's modulus of elasticity will become two times.
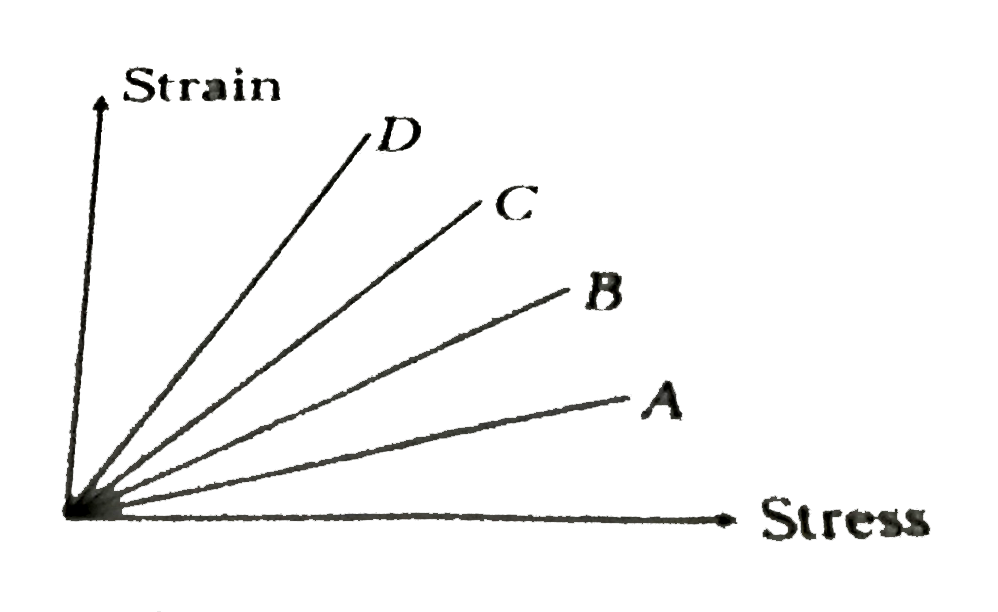
Reason The ratio of longitudinal stress and longitudinal strain is called Young's modulus of elasticity.View SolutionStress-strain curve for four metals are shown in figure. The maximum Young's modulus of elasticity is for metal
 View Solution
View SolutionA steel wire is stretched by 1kgwt. If the radius of the wire is doubled, its Young's modulus will
View SolutionYoung modulus of elasticity for a gas is
View SolutionA bar of mass m and length l is hanging from point A as shown in the figure . If the Young's modulus of elasticity of the bar is Y and area of cross - section of the wire is A, then the increase in its length due to its own weight will be
 View Solution
View SolutionAssertion Bulk modulus of elasticity of gases is pressure dependent.
Reason More the pressure of gas, more is the Bulk modulus of elasticity of gas.View SolutionAssertion The materials having low value of Young's modulus of elasticity are more ductile.
Reason If Young's modulus is less, they can be easily stretched as wires.View SolutionA metallic wire of length l is held between two rigid supports. If the wire is cooled through a temperature t. (Y= Young's modulus of elasticity of wire, ρ= density, α= thermal coefficient of linear expansion). Then the frequency of oscillation is proportional to
View Solution

