Can the instantaneous power output of an ac source ever be negative ? Can the average power output be negative ?
The correct Answer is:A
To answer the question regarding the instantaneous and average power output of an AC source, we can break down the solution into clear steps.
1. Understanding Instantaneous Power:
The instantaneous power P of an AC source can be expressed using the formula:
P=V0I0sin(ωt)sin(ωt+ϕ)
where V0 is the peak voltage, I0 is the peak current, ω is the angular frequency, and ϕ is the phase difference.
Hint: Remember that instantaneous power varies with time and depends on the sine functions of the voltage and current.
2. Using Trigonometric Identities:
We can use the product-to-sum identities to simplify the expression for instantaneous power:
P=V0I02(cos(ϕ)−cos(2ωt+ϕ))
Hint: Familiarize yourself with trigonometric identities, especially the product-to-sum formulas.
3. Analyzing the Terms:
In the expression cos(ϕ)−cos(2ωt+ϕ), we note that cos(ϕ) is a constant value, while cos(2ωt+ϕ) oscillates between -1 and 1.
Hint: Consider the range of cosine functions and how they affect the overall expression.
4. Determining Conditions for Negative Instantaneous Power:
Since cos(2ωt+ϕ) can take values from -1 to 1, there will be instances when:
cos(ϕ)<cos(2ωt+ϕ)
This means that the term cos(ϕ)−cos(2ωt+ϕ) can become negative, leading to:
P<0
Therefore, the instantaneous power can indeed be negative.
Hint: Think about how the oscillating nature of AC affects power calculations.
5. Understanding Average Power:
The average power Pavg over one complete cycle is given by:
Pavg=V0√2⋅I0√2⋅cos(ϕ)=VrmsIrmscos(ϕ)
where Vrms and Irms are the root mean square values of voltage and current, respectively.
Hint: Average power is calculated over a full cycle, which smooths out the instantaneous variations.
6. Analyzing Average Power:
The term cos(ϕ) (the power factor) is always between -1 and 1, but since it represents the cosine of the phase difference, it will always be non-negative when considering resistive loads. Thus, Pavg cannot be negative.
Hint: Remember that average power reflects the effective power consumed by the circuit, which cannot be negative.
Conclusion:
- The instantaneous power of an AC source can be negative due to the oscillating nature of the sine functions involved.
- The average power output of an AC source cannot be negative as it is always dependent on the non-negative power factor cos(ϕ).
The instantaneous power P of an AC source can be expressed using the formula:
P=V0I0sin(ωt)sin(ωt+ϕ)
where V0 is the peak voltage, I0 is the peak current, ω is the angular frequency, and ϕ is the phase difference.
We can use the product-to-sum identities to simplify the expression for instantaneous power:
P=V0I02(cos(ϕ)−cos(2ωt+ϕ))
In the expression cos(ϕ)−cos(2ωt+ϕ), we note that cos(ϕ) is a constant value, while cos(2ωt+ϕ) oscillates between -1 and 1.
Since cos(2ωt+ϕ) can take values from -1 to 1, there will be instances when:
cos(ϕ)<cos(2ωt+ϕ)
This means that the term cos(ϕ)−cos(2ωt+ϕ) can become negative, leading to:
P<0
Therefore, the instantaneous power can indeed be negative.
The average power Pavg over one complete cycle is given by:
Pavg=V0√2⋅I0√2⋅cos(ϕ)=VrmsIrmscos(ϕ)
where Vrms and Irms are the root mean square values of voltage and current, respectively.
The term cos(ϕ) (the power factor) is always between -1 and 1, but since it represents the cosine of the phase difference, it will always be non-negative when considering resistive loads. Thus, Pavg cannot be negative.
- The instantaneous power of an AC source can be negative due to the oscillating nature of the sine functions involved.
- The average power output of an AC source cannot be negative as it is always dependent on the non-negative power factor cos(ϕ).
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Short Answer types questions1videos
ALTERNATING CURRENT
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Long Answe types Questions5videos
ALTERNATING CURRENT
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Long Answe types Questions5videos
ALTERNATING CURRENT
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ATOMSEXERCISE - Long Answer Type6videos
ATOMS
Similar Questions
Can scalar product be ever negative ?
View SolutionCan scalar product be ever negative ?
View SolutionCan the instantaneous velocity of an object during a time interval ever be greater in magnitude than average celocity over the entire interval ? Can it be less?
View SolutionCan kinetic energy be negative? Explain.
View SolutionCan specific heat of a gas be negative?
View SolutionIn a normal adult human, the average cardiac output is
View SolutionCan the experimental probability of an event be a negative number? If not, why?
View SolutionStatement-1 : Instantaneous power across capacitor under AC source in a RC circuits will be having positive and negative values alternately.
Statement-2 : emf of the alternating source varies sinusoidally.View SolutionCan a reaction have negative activation energy?
View SolutionA device 'X' is connected to an AC source. The variation of voltage, current and power in one complete cycle is shown in figure.
a) Which curve shows power consumption over a full cycle?
b) What is the average power consumption over a cycle?
c) Identify the device X.
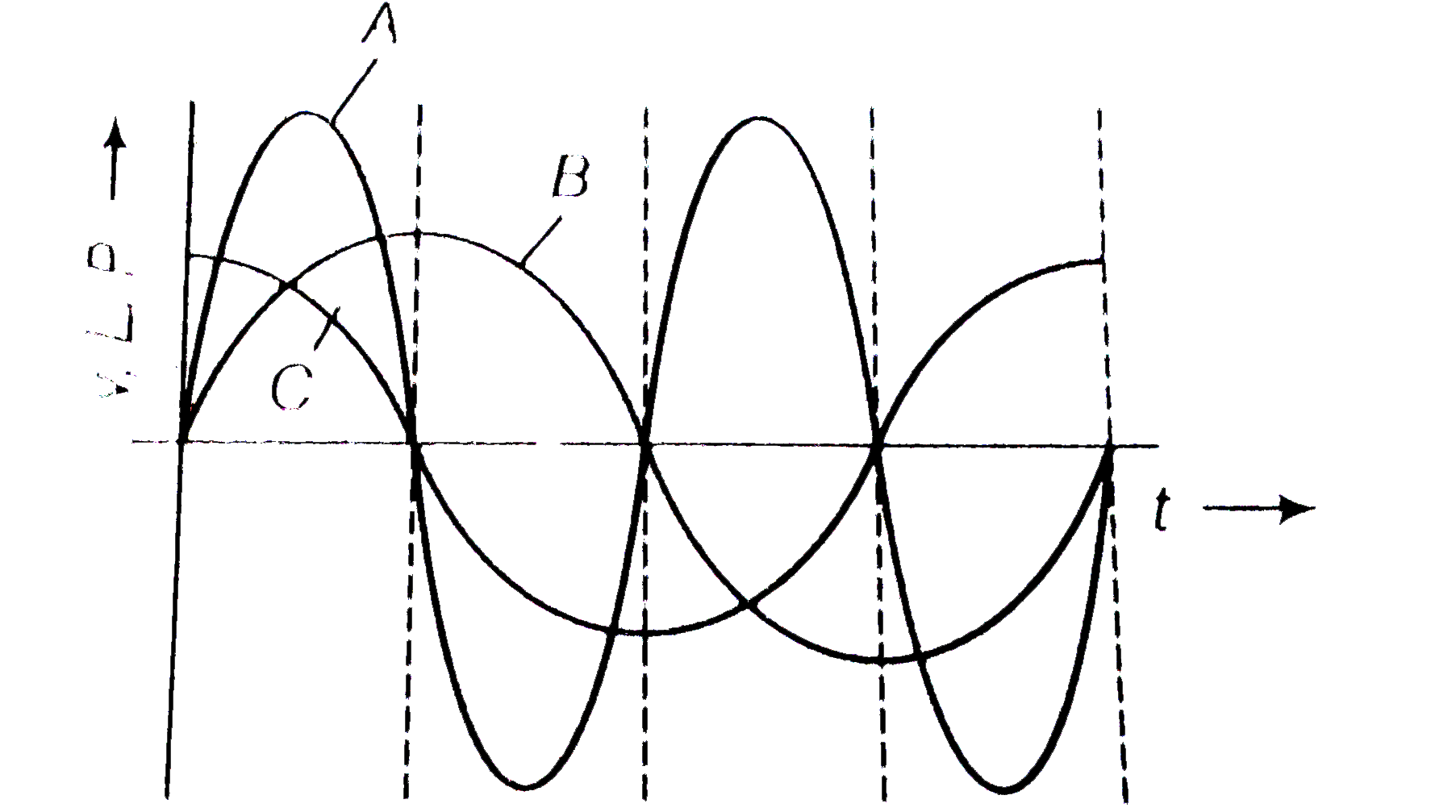 View Solution
View Solution

