An electrical device draws 2 kW power form AC mains [voltage 223 V (rms) =√50,000V]. The current differs (lags) in phase by ϕ(tan=−34) as compared to voltage. Find (i) R, (ii) XC−XL, and (iii) IM. Another device has twice the values for R, XC and XL. How are the answers affected ?
The correct Answer is:A
To solve the problem step by step, we will follow the given information and apply the relevant formulas.
Given Data:
1. Power (P) = 2 kW = 2000 W
2. RMS Voltage (V) = 223 V
3. Phase difference (tan φ) = -3/4
Step 1: Calculate Impedance (Z)
Using the formula for power in an AC circuit:
P=V2Z
We can rearrange this to find Z:
Z=V2P
Substituting the values:
Z=(223)22000=497292000=24.8645≈25Ω
Step 2: Calculate Resistance (R)
We know from the relationship involving tan φ:
tanφ=XC−XLR
Given that tanφ=−34:
−34=XC−XLR
Thus,
XC−XL=−34R
Step 3: Use the Impedance Formula
The impedance Z can also be expressed as:
Z2=R2+(XL−XC)2
Substituting the known value of Z:
252=R2+(XL−XC)2
This gives us:
625=R2+(XL−XC)2
Step 4: Substitute for XC−XL
From the equation XC−XL=−34R, we can express XL−XC as:
XL−XC=34R
Substituting this into the impedance equation:
625=R2+(34R)2
Calculating (34R)2:
(34R)2=916R2
So, we have:
625=R2+916R2
Combining terms:
625=(1+916)R2=2516R2
Thus:
R2=625×1625=400
Taking the square root:
R=20Ω
Step 5: Calculate XC−XL
Now substituting R back into the equation for XC−XL:
XC−XL=−34×20=−15Ω
Step 6: Calculate Maximum Current IM
The RMS current I can be calculated as:
I=VZ=22325=8.92A
To find the maximum current IM:
IM=√2×I=√2×8.92≈12.6A
Summary of Results:
1. Resistance R=20Ω
2. XC−XL=−15Ω
3. Maximum Current IM≈12.6A
Part (ii): Effect of Doubling R, XC, and XL
If another device has twice the values for R, XC, and XL:
- New R=40Ω
- New XC−XL=−30Ω
The impedance Z will also increase, leading to:
- New Z will be higher, thus the current will decrease (half of the original).
- Power will also be half since P=I2R and I is halved.
1. Power (P) = 2 kW = 2000 W
2. RMS Voltage (V) = 223 V
3. Phase difference (tan φ) = -3/4
Using the formula for power in an AC circuit:
P=V2Z
We can rearrange this to find Z:
Z=V2P
Substituting the values:
Z=(223)22000=497292000=24.8645≈25Ω
We know from the relationship involving tan φ:
tanφ=XC−XLR
Given that tanφ=−34:
−34=XC−XLR
Thus,
XC−XL=−34R
The impedance Z can also be expressed as:
Z2=R2+(XL−XC)2
Substituting the known value of Z:
252=R2+(XL−XC)2
This gives us:
625=R2+(XL−XC)2
From the equation XC−XL=−34R, we can express XL−XC as:
XL−XC=34R
Substituting this into the impedance equation:
625=R2+(34R)2
Calculating (34R)2:
(34R)2=916R2
So, we have:
625=R2+916R2
Combining terms:
625=(1+916)R2=2516R2
Thus:
R2=625×1625=400
Taking the square root:
R=20Ω
Now substituting R back into the equation for XC−XL:
XC−XL=−34×20=−15Ω
The RMS current I can be calculated as:
I=VZ=22325=8.92A
To find the maximum current IM:
IM=√2×I=√2×8.92≈12.6A
1. Resistance R=20Ω
2. XC−XL=−15Ω
3. Maximum Current IM≈12.6A
If another device has twice the values for R, XC, and XL:
- New R=40Ω
- New XC−XL=−30Ω
- New Z will be higher, thus the current will decrease (half of the original).
- Power will also be half since P=I2R and I is halved.
Topper's Solved these Questions
Similar Questions
An electrical device draws 0.968 kW form AC mains of 220 V.If current lags voltage in phase by ϕ=tan−1(23).The value of resistance is
View SolutionA device X is connected across an ac source of voltage V=V0sinωt. The current throught X is given as I=I0sin(ωt+π2)
(a) Identify the device X and write the expression for its reactance.
(b) Draw graph showing variation of voltage and current with time over one cycle of ac, for X.
How does the reactance of the device X vary with frequency of the ac? Show this variation graphically.
(d) Draw the phasor diagram for the device X.
View Solution
Knowledge Check
An electrical device draws 2 kW power from ac mains voltage 223 V(rms). The current differs lags in phase by ϕ=tan−1(−34) as compared to valtage. The resistance R in the circuit is
A15ΩB20ΩC25ΩD30Ω
(a) Draw graphs showing the variations of inductive reactnace and capacitive reactance with frequency of the applied ac source .
(b) Draw the phasor diagram for a series RC circuit connected to an ac source.
(c ) An alternating voltage of 220 V is applied across a device X , a current of 0.25 A flows , which lag behind the applied voltage in phase by π2 radian . If the same voltage is applied across another device Y , the same current flows but now it is in phase with the applied voltage .
(i) Name the devices X and Y.
(ii) Calculate the current flowing in the circuit when the same voltage is applied across the series combination of X and Y.

View SolutionA capacitor (C) and a resistor (R) are connected in series with an a.c, source of voltage of frequency 50 Hz. The potential difference across C and R are respectively 120 V and 90 V. The current in the circuit is 3A. Calculate () impedance of the circuit, (i) value of the inductance which when connected in series with Cand R will make the power fador of the circuit unity.
View SolutionIn an alternating circuit applied voltage is 200V if R=8WXL=XC=6Ωthen write the value of the following (a)Root mean square value of voltage , (b)Impedance of circuit.
View SolutionIn an alternating circuit applied voltage is 200V, if R=8WXL=XC=6Ω then write the value of the following
(a)Root mean square value of voltage
(b)Impedance of circuit.
View SolutionIn the circuit shown in figure :
R=10Ω,L=√310H,R2=20Ω and C=√32mF. Current in L−R1 circuit is I1 in C−R1 circuit is I2 and the main current is I
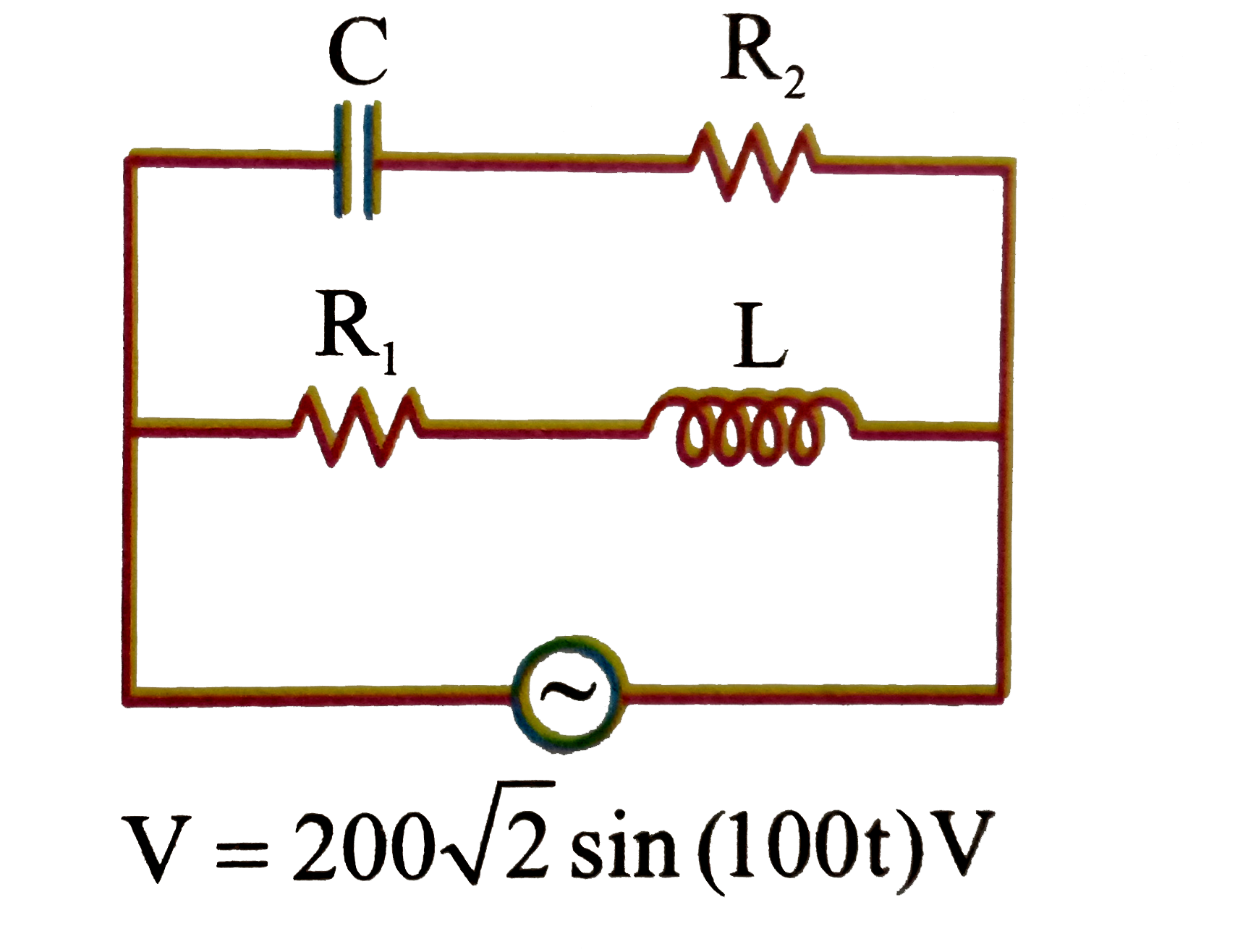
Phase difference between I1 and I2 is
View SolutionIn the circuit shown in figure :
R=10Ω,L=√310H,R2=20Ω and C=√32mF. Current in L−R1 circuit is I1 in C−R1 circuit is I2 and the main current is I
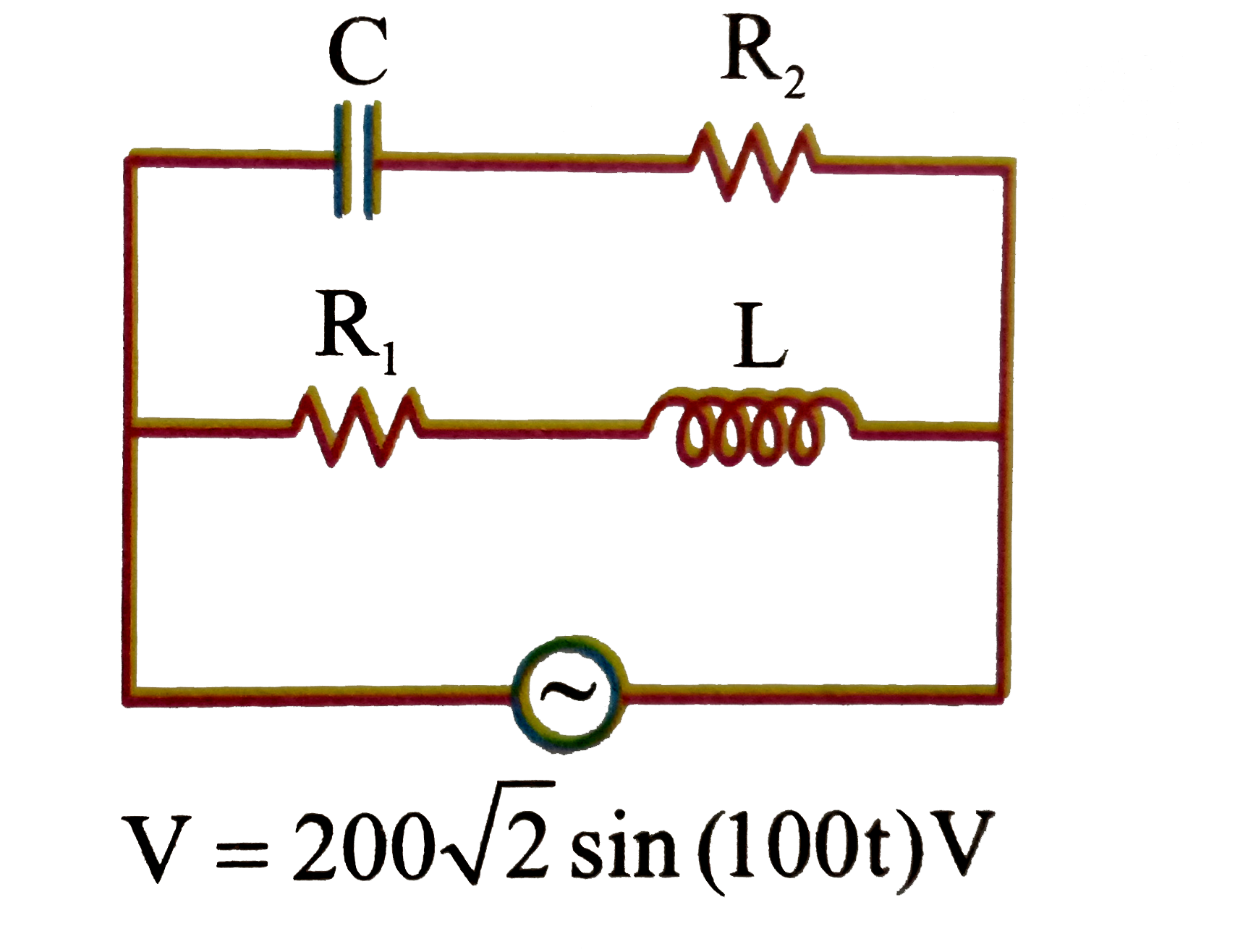
Phase difference between I1 and I2 is
View SolutionIn an AC, series L-C-R circuit, R=XL=XC and applied AC, voltage is V. Then match the following two columns.
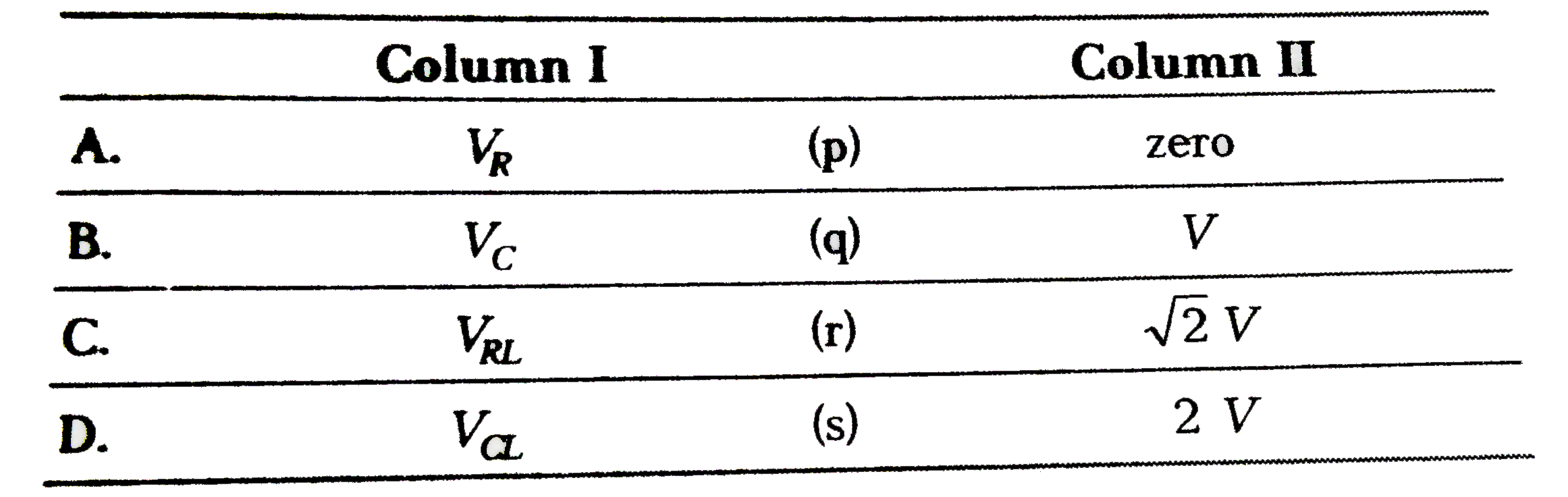
View Solution
