14 In Fig. 6.21, PA, QB Rc and SD are all perpendiculars to a line l, AB 6 cm, Bc 9 cm, CD 12 cm and SP 36 cm Find PO, QR an RS. Fig. 6.21
To solve the problem step by step, we will use the properties of similar triangles and the concept of proportionality.
Given:
- AB=6cm
- BC=9cm
- CD=12cm
- SP=36cm
To Find:
- PQ
- QR
- RS
Step 1: Establish the Proportional Relationships
Since PA,QB,RC, and SD are all perpendicular to line l, the segments PQ,QR, and RS are parallel to AB,BC, and CD. Therefore, we can use the Base Proportionality Theorem (also known as Thales' theorem) which states that if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally.
Step 2: Set Up the Proportions
From the problem, we can set up the following proportions:
PQAB=QRBC=RSCD
Let PQ=6x, QR=9x, and RS=12x.
Step 3: Write the Equation for Total Length
The total length from P to S is given as:
PQ+QR+RS=PS
Substituting the expressions we found:
6x+9x+12x=36
Step 4: Combine Like Terms
Combine the terms on the left side:
(6+9+12)x=36
27x=36
Step 5: Solve for x
Now, divide both sides by 27:
x=3627=43
Step 6: Calculate PQ,QR, and RS
Now substitute x back into the expressions for PQ,QR, and RS:
1. PQ=6x=6×43=8cm
2. QR=9x=9×43=12cm
3. RS=12x=12×43=16cm
Final Answers:
- PQ=8cm
- QR=12cm
- RS=16cm
- AB=6cm
- BC=9cm
- CD=12cm
- SP=36cm
- PQ
- QR
- RS
Since PA,QB,RC, and SD are all perpendicular to line l, the segments PQ,QR, and RS are parallel to AB,BC, and CD. Therefore, we can use the Base Proportionality Theorem (also known as Thales' theorem) which states that if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally.
From the problem, we can set up the following proportions:
PQAB=QRBC=RSCD
Let PQ=6x, QR=9x, and RS=12x.
The total length from P to S is given as:
PQ+QR+RS=PS
Substituting the expressions we found:
6x+9x+12x=36
Combine the terms on the left side:
(6+9+12)x=36
27x=36
Now, divide both sides by 27:
x=3627=43
Now substitute x back into the expressions for PQ,QR, and RS:
1. PQ=6x=6×43=8cm
2. QR=9x=9×43=12cm
3. RS=12x=12×43=16cm
- PQ=8cm
- QR=12cm
- RS=16cm
Topper's Solved these Questions
Similar Questions
In the given figure, AB and DE are perpendiculars to BC
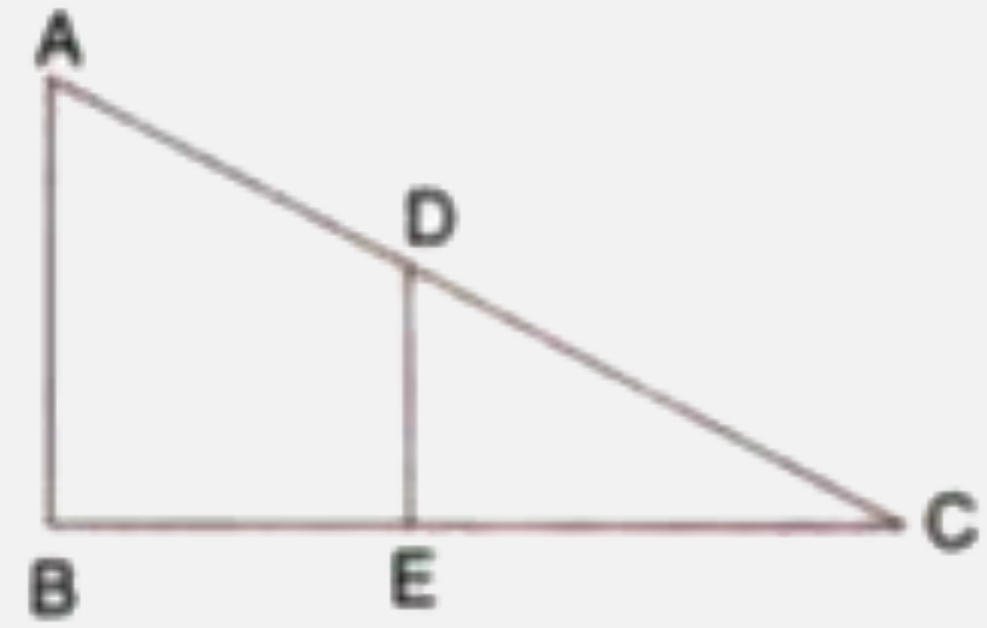
If AB = 6 cm, DE = 4 cm and AC = 15 cm. Calculate CD.
View SolutionIn the given figure, AB and DE are perpendicular to BC.

(iI) If AB = 6 cm, DE = 4 cm and AC = 15 cm. Calculate CD.
View SolutionConstruct a ΔABC such
BC=6cm,CA=4cmandAB=3.5cm
View SolutionIn the figure, given alongside, PB and QA are perpendiculars to the line segment AB. If PO = 6 cm, QO = 9 cm and area of ΔPOB−120cm2. find the area of ΔQOA.

View SolutionConstruct a ΔABC such
AB=5cm,BC=4.6cmandAC=4.3cm
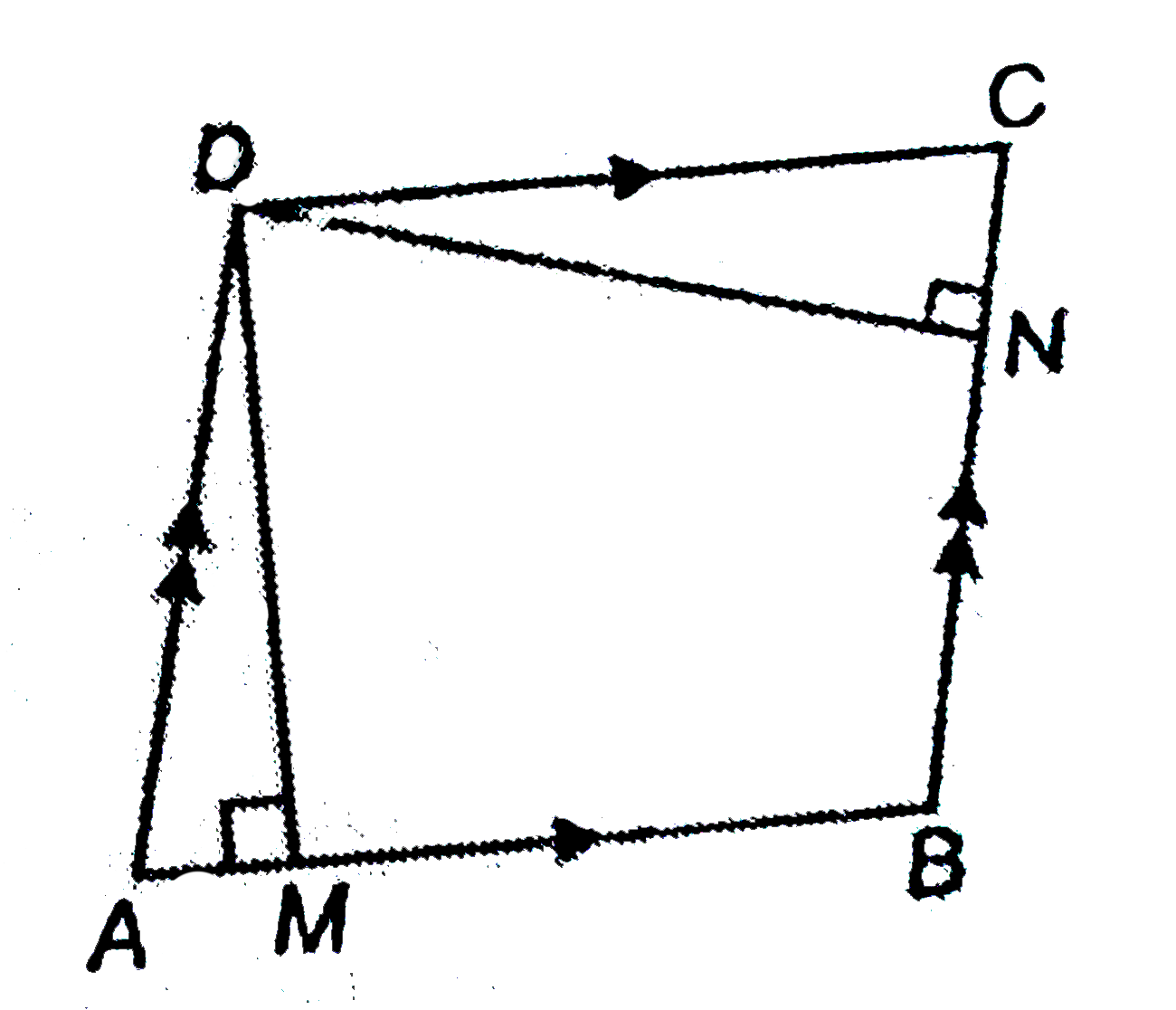
View SolutionIn the adjoining figure, AB=8cm,DM=6cmandBC=6cm. Find the length of DN
View SolutionIn the given figure, AB and DE are perpendicular to BC. If AB = 6cm, DE = 4cm and AC = 15cm.

(iii) Find the ratio of the area of ΔABC : area of ΔDEC.
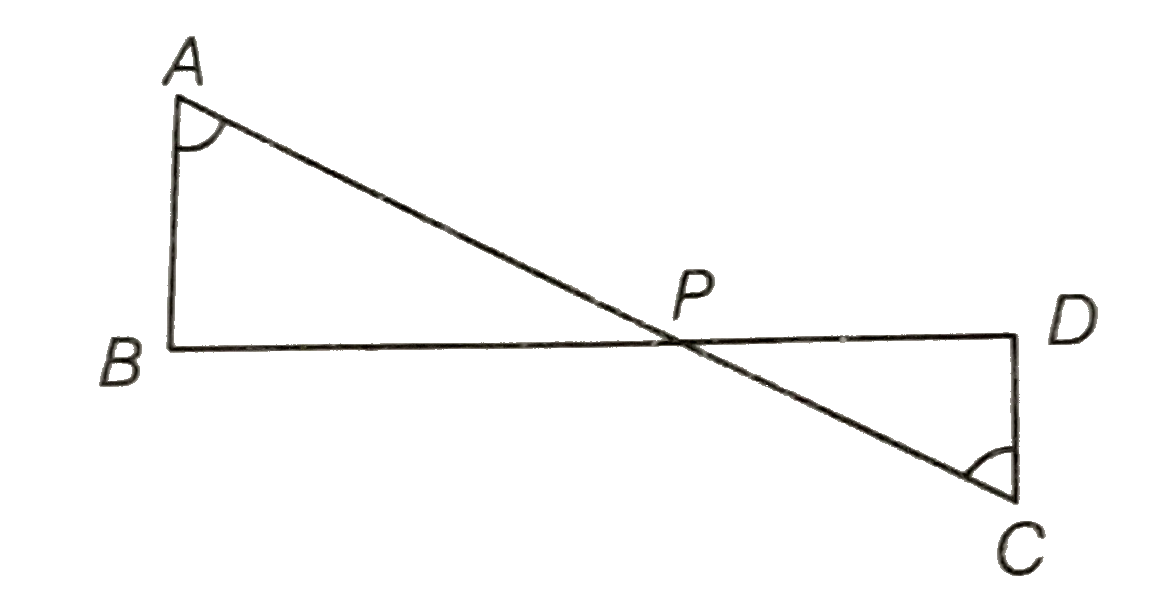
View SolutionIn given figure, if ∠A=∠C, AB= 6 cm, BP = 15 cm, AP = 12 cm and CP= 4 cm, then find the lengths of PD and CD.
View SolutionIn the given figure, if DE || BC, AE = 8 cm, EC = 2 cm and BC = 6 cm, then find DE
View SolutionConstruct Δ ABC with AB = 4.5 cm, BC = 7 cm and CA = 6 cm.
View Solution
