Find the coordinates of one vertex of an equilateral triangle with centroid at the origin and the opposite side x+y−2=0.
(−1,−1)
(2,2)
(−2,−2)
(2,−2)
The correct Answer is:C
Let ABC be the equilateral triangle iwth vertex A(h,k).
Let the coordinates of D are (α,β).
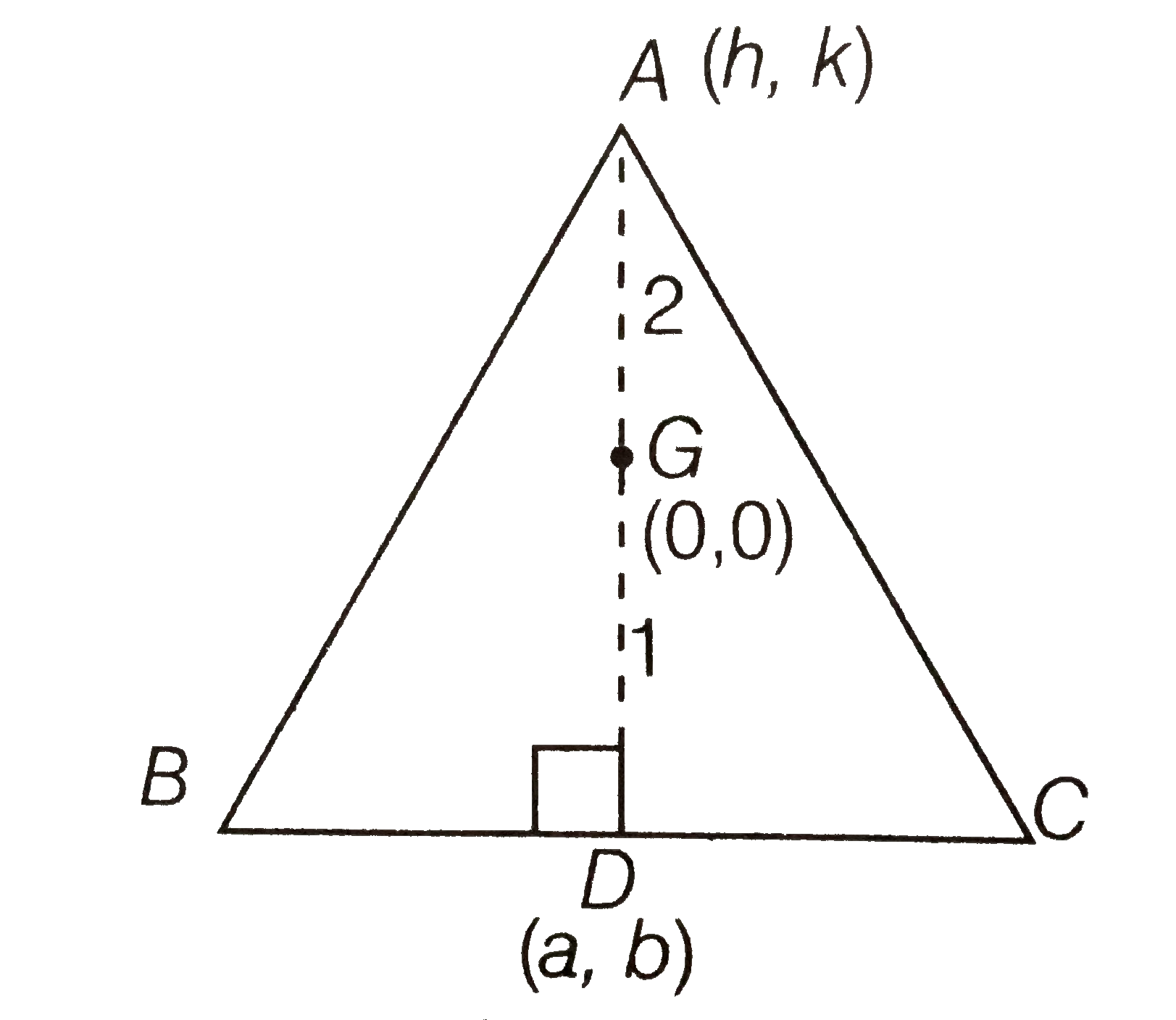
We know that 2:1 from the vertexA.
∴0=2α+h3 and 0=2β+k3
⇒2α=−h ......(i)
and 2β=−k
Also, D (α,β)lies on the line x+Y−2=0......(ii) .
∴α+β−2=0
AD⊥BC
Since, the slope of line BC i.e., mBC=−1
and slope of the line AG i.e., mAG=k−0h−0=kh
⇒(−1).(kh)=−1
⇒h=k ......(iii)
From Eqs. (i) and (iii),
2α=−h and 2β=−h
∴α=β
From Eq. (ii), 2α−2=0⇒α=1
If α=1 then β=1
So, the vertex A is (−2,−2).
Topper's Solved these Questions
- STRAIGHT LINESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Fillers
6 Videos
- STRAIGHT LINESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - TRUE/FALSE
9 Videos
- STRAIGHT LINESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Long Answer type
9 Videos
- STATISTICSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICSEXERCISE - FILLERS
7 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - TRUE/FALSE
9 Videos
Similar Questions
One vertex of the equilateral triangle with centroid at the origin and one side as x+y−2=0 is :
View SolutionFind the coordinates of the vertices of an equilateral triangle of side 2a as shown in Figure.
View SolutionFind the coordinates of vertex of the parabola y2=4(x+y)
View SolutionWrite the coordinates of third vertex of a triangle having centroid at the origin and two vertices at (3,-5, 7) and (3,0,1).
View SolutionABC is an equilateral triangle whose centroid is origin and base BC is along the line 11x+60y=122. Then
View SolutionFind the area of an equilateral triangle, whose one side is a cm.
View SolutionIf one vertex of an equilateral triangle of side 'a' lie at the origin and the other lies on the line x−√3y=0, the co-ordinates of the third vertex are:
View SolutionA vertex of an equilateral triangle is 2,3 and the opposite side is x+y=2. Find the equations of other sides.
View SolutionA vertex of an equilateral triangle is (2,3) and the opposite side is x+y=2. Find the equations of other sides.
View SolutionThe co-ordinates of the vertex of an equilateral triangle are (2,−1) and equation of its base is x+y−1=0. Find the equations of its other two sides.
View Solution
