An a.c. source generating a voltage $v = v + (m) sin ω t i s c o \cap e c t e d \to a \cap a c i \to r o f \cap a c i tan c e C . F \in d t h e exp r e s s i o n f or t h e c u r r e n t, I, f l o w \in g t h r o u g h i t . P l o t a g r a p h o f$ v $and I v e r s u s$ omega $t \to s h o w t ˆ t h e c u r r e n t i s$ pi//2 $a h e a d o f t h e v o < a \geq . < b r > A r e s i s \to r o f$ 200 Omega $and a \cap a c i \to r o f$ 15.0 mu F are connected in series to a 220 V, 50 Hz a.c. source. Calculate the current in the circuit and the rms voltage across the resistor and the capacitor. Is the algebraic sum of these voltages more than the source voltages ? If yes, resolve the paradox.

Text Solution

Verified by Experts

A.C. source containing capacitor : Let a source of alternating e.m.f. $V = V_{m} sin ω t$ be connected to a capacitor of capacitance C only
$V = V_{m} sin ω t$
At every instant, the potential V is given by
$V = \frac{q}{c} \Rightarrow V_{m}$
$sin ω t = \frac{q}{c} ∴ q = C V_{m} sin ω t$ .
If i is instantaneous value of current in the circuit at instant t, then
$i = \frac{d q}{d t} = \frac{d}{d t} (C V_{m} sin ω t)$
$i = C V_{m} (cos ω t) . ω = \frac{1 / m}{1 / ω c} sin (ω t + \frac{π}{2})$
The current will be maximum . When ( $sin ω t + \frac{π}{2}) = 1$
$∴ i_{m} = \frac{V_{m}}{1 / ω c} \times 1 = \frac{V_{m}}{1 / ω c}$
$i = i_{m} sin (ω t + \frac{π}{2})$ .
Therefore, alternating current I leads the alternating voltage by a phase angle of $\frac{π}{2}$ .

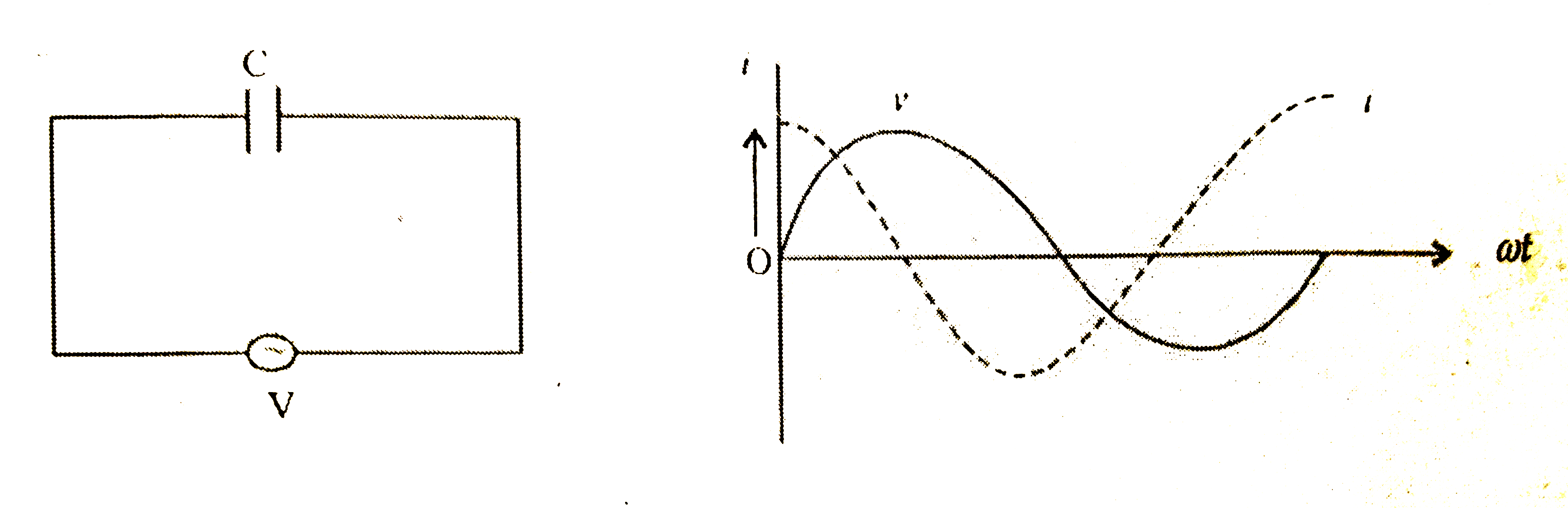
Numerical :
Here $R = 200 Ω$
$C = 15.0 μ F = 15 \times 10^{- 6} F, V_{r m s} = 220 V$ ,
$υ = 50 H z$
$I_{r m s} = ?$
$X_{c} = \frac{1}{ω c} = \frac{1}{2 π υ c}$
$= \frac{1}{2 \times \frac{22}{7} \times 50 \times 15 \times 10^{- 6}} = \frac{7 \times 10^{6}}{33000}$
$212.12 \approx 212 Ω$ .
$∴ Z = \sqrt{R^{2} + X_{c}^{2}} = \sqrt{{(200)}^{2} + {(212)}^{2}}$
$= \sqrt{40000 + 44944} = \sqrt{84944}$
$= 291.45 Ω$
$∴ I_{r m s} = \frac{V_{r m s}}{Z} = \frac{220}{291.45}$
$= 0.75 A$
$V_{R} = I_{r m s} R = 0.75 \times 200$
$= 150 V$
$V_{C} = I_{r m s} . X_{C}$
$= 0.75 \times 212 = 159 V$
$∴ V_{R} + V_{C} = 150 + 159 = 309$
$∴ V_{R} + V_{C} < V$
This is because these voltages are not in same phase and they cannot be added like ordinary numbers.
$V = \sqrt{V_{R}^{2} + V_{C}^{2}}$
$= \sqrt{{(150)}^{2} + {(159)}^{2}} = \sqrt{22500 + 25281}$
$= \sqrt{47781} = 218.58 V$ .

Updated on:21/07/2023

A resistance of $200 Ω$ and capacitor of $15 μ F$ are connected in series to a 220 V, 50 Hz AC source. The voltage (rms) across the resistor and capacitor is that
A151 V, 160.4 V
B150 V, 100.3 V
C220 V, 91.8 V
D145 V, 311.3 V
A $0.2 k Ω$ resistor and $15 μ F$ capacitor are connected in series to a 220 V, 50 Hz ac source. The impedance of the circuit is
A $250 Ω$
B $268 Ω$
C $29.15 Ω$
D $291.5 Ω$
A $0.2 k Ω$ resistor and $15 μ F$ capacitor are connected in series to a 220 V, 50 Hz ac source. The impadance of the circuit is
A $250 Ω$
B $268 Ω$
C $29.15 Ω$
D $291.5 Ω$