Two wires of equal length one of copper and other of manganin have the same resistance. Which wire is thicker?
To solve the problem, we will use the formula for resistance and the relationship between resistivity, length, and cross-sectional area.
1. Understanding the Resistance Formula:
The resistance R of a wire is given by the formula:
R=ρLA
where:
- R is the resistance,
- ρ is the resistivity of the material,
- L is the length of the wire,
- A is the cross-sectional area of the wire.
2. Cross-Sectional Area:
The cross-sectional area A can be expressed in terms of the radius r of the wire:
A=πr2
3. Given Conditions:
- The two wires (copper and manganin) have the same length L.
- The resistances of both wires are equal, i.e., RCu=RMg.
4. Setting Up the Equations:
Since the resistances are equal, we can write:
ρCuLACu=ρMgLAMg
Since the lengths L are the same, they can be canceled out:
ρCuACu=ρMgAMg
5. Substituting the Area:
Substitute A with πr2:
ρCuπr2Cu=ρMgπr2Mg
The π cancels out:
ρCur2Cu=ρMgr2Mg
6. Rearranging the Equation:
Rearranging gives:
r2Cu⋅ρCu=r2Mg⋅ρMg
7. Analyzing Resistivities:
We know that:
- The resistivity of copper ρCu is lower than that of manganin ρMg (i.e., ρCu<ρMg).
- Therefore, for the equation r2Cu⋅ρCu=r2Mg⋅ρMg to hold true, r2Cu must be smaller than r2Mg.
8. Conclusion:
Since r2Cu<r2Mg, it follows that:
rCu<rMg
This means that the manganin wire is thicker than the copper wire.
Final Answer:
The manganin wire is thicker than the copper wire.
The resistance R of a wire is given by the formula:
R=ρLA
where:
- R is the resistance,
- ρ is the resistivity of the material,
- L is the length of the wire,
- A is the cross-sectional area of the wire.
The cross-sectional area A can be expressed in terms of the radius r of the wire:
A=πr2
- The two wires (copper and manganin) have the same length L.
- The resistances of both wires are equal, i.e., RCu=RMg.
Since the resistances are equal, we can write:
ρCuLACu=ρMgLAMg
Since the lengths L are the same, they can be canceled out:
ρCuACu=ρMgAMg
Substitute A with πr2:
ρCuπr2Cu=ρMgπr2Mg
The π cancels out:
ρCur2Cu=ρMgr2Mg
Rearranging gives:
r2Cu⋅ρCu=r2Mg⋅ρMg
We know that:
- The resistivity of copper ρCu is lower than that of manganin ρMg (i.e., ρCu<ρMg).
- Therefore, for the equation r2Cu⋅ρCu=r2Mg⋅ρMg to hold true, r2Cu must be smaller than r2Mg.
Since r2Cu<r2Mg, it follows that:
rCu<rMg
This means that the manganin wire is thicker than the copper wire.
The manganin wire is thicker than the copper wire.
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) [SET - II]
6 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) [SET - III]
9 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - [Set-III]
3 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
Two wires, one of copper and the other of iron, are of the same length and same radius. Which will have more resistance ? Give reason.
View SolutionTwo copper wires are of the same length, but one is thicker than the other :
(a) Which wire will have more resistance?
(b) Which wire will have more specific resistance?
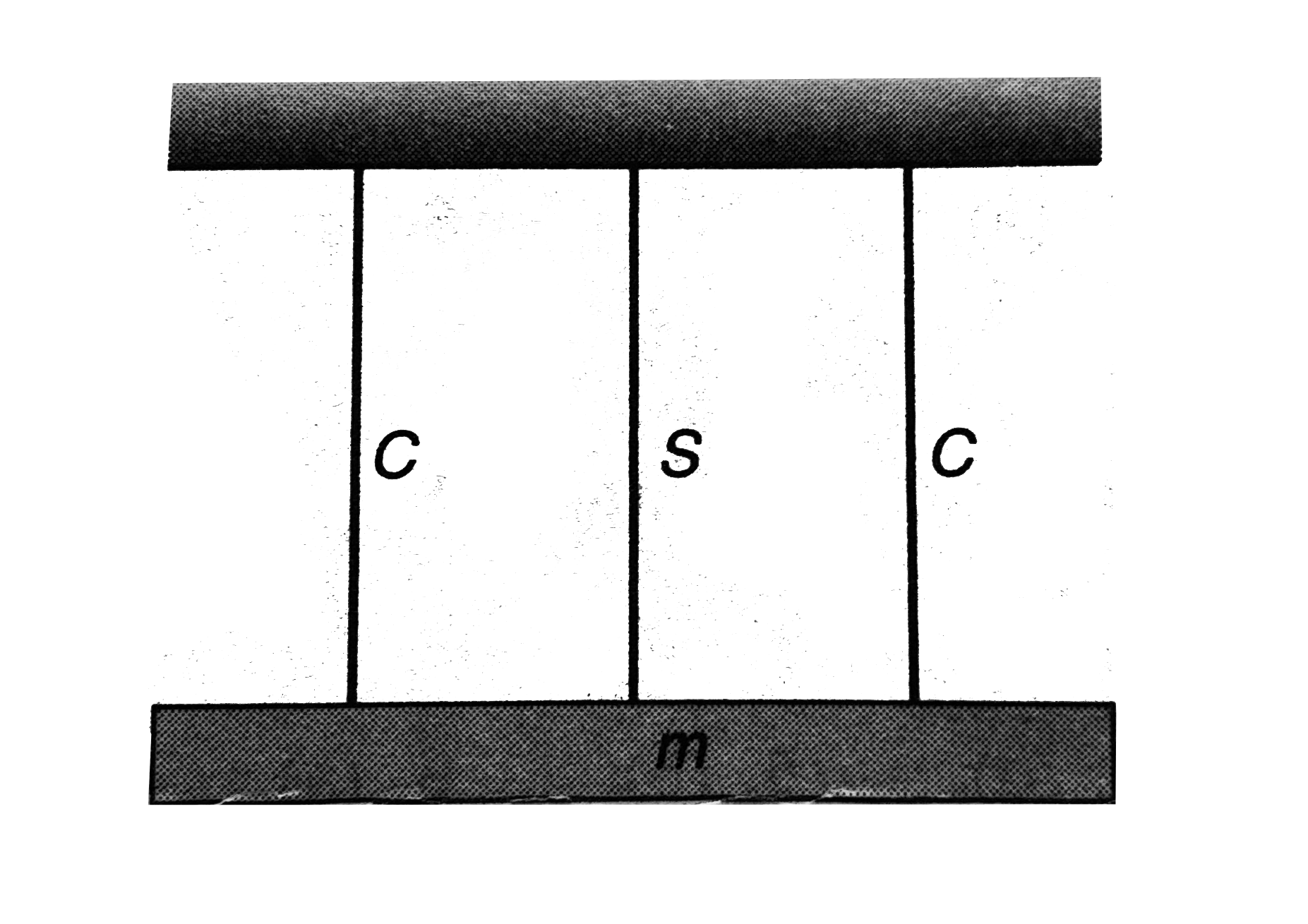
View SolutionA homogeneous block with a mass m hangs on three vertical wires of equal length arranged symmetrically . Find the tensions of the wires if the middle wire is of steel and the other two are of copper . All the wires have the same cross - section . Consider the modulus of elasticity of steel to be double than that of copper .
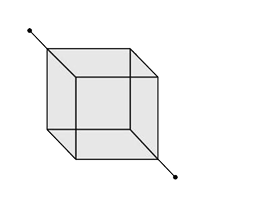
View SolutionTwelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R, and the effective resistance between the two diagonal ends is Re, then the ratio RRe is equal to __.
View SolutionTwo copper wires are of the same length, but one is thicker than the other.
(1) Which wire will have more resistance ?
(2) Which wire will have more specific resistance ?
View SolutionA metal wire of resistance R is cut into three equal pieces that are then connected side by side to form a new wire, the length of which is equal to one third of the original length. The resistance of this new wire is:-
View SolutionTwo wires of equal cross section but one made of steel and the other of copper, are joined end to end. When the combination is kept under tension, the elongations in the two wires are found to be equal. Find the ratio of the lengths of the two wires. Young modulus of steel =2.0×1011Nm−2 and that of copper =1.1×1011Nm−2
View SolutionTwo wires of equal length are stretched by the same force. One wire is heavy while the other is light which one will have less frequency
View SolutionTwo wires A and B have equal lengths and aremade of the same material , but diameter of wire A is twice that of wire B. Then, for a given load,
View Solution(a) Name two factors on which the specific resistance of a wire depends ?
(b) Two wires A and B are made of copper. The wire A is long and thin, while the wire B is short and thick. Which will have more specific resistance ?
View Solution
