(i) Derive the expression for electric field at a point on the equatorial line of an electric dipole.
(ii) Depiet the orientation of the dipole in (i) stable, (ii) unstable equilibrium in a uniform electric field.
Step-by-Step Solution
1. Understanding the Dipole Configuration:
- Consider an electric dipole consisting of two charges, +q and -q, separated by a distance of 2a. The dipole moment →p is defined as →p=q⋅2a.
2. Identifying the Point of Interest:
- Let point P be located on the equatorial line of the dipole at a distance r from the midpoint of the dipole.
3. Distances from Charges to Point P:
- The distance from the positive charge (+q) to point P is r2+a2 (using the Pythagorean theorem), and similarly, the distance from the negative charge (-q) to point P is also r2+a2.
4. Calculating Electric Fields Due to Each Charge:
- The electric field E1 due to the positive charge (+q) at point P is given by:
E1=14πϵ0q(r2+a2)
- The electric field E2 due to the negative charge (-q) at point P is:
E2=14πϵ0q(r2+a2)
5. Direction of Electric Fields:
- The electric field E1 due to the positive charge points away from the charge, while E2 due to the negative charge points towards the charge. Thus, the two fields will have components that can be resolved.
6. Resolving the Electric Fields:
- The vertical components of E1 and E2 will cancel each other out due to symmetry. Only the horizontal (cosine) components will add up.
- The horizontal component of E1 is E1cosθ and for E2 it is E2cosθ.
7. Calculating the Resultant Electric Field:
- The resultant electric field E at point P is:
E=E1cosθ+E2cosθ=2E1cosθ
- Substituting E1:
E=2(14πϵ0q(r2+a2))cosθ
8. Substituting for cosθ:
- Using the geometry of the dipole, cosθ=a√r2+a2:
E=2(14πϵ0q(r2+a2))a√r2+a2
9. Final Expression:
- Rearranging gives:
E=p4πϵ0(r2+a2)3/2
- where p=q⋅2a is the dipole moment.
(ii) Orientation of the Dipole in Stable and Unstable Equilibrium
1. Stable Equilibrium:
- When the dipole is aligned with the electric field (θ = 0), the potential energy U is given by:
U=−pEcos(0)=−pE
- This is the minimum potential energy, indicating stable equilibrium.
2. Unstable Equilibrium:
- When the dipole is aligned opposite to the electric field (θ = π), the potential energy U is:
U=−pEcos(π)=pE
- This represents maximum potential energy, indicating unstable equilibrium.
- Consider an electric dipole consisting of two charges, +q and -q, separated by a distance of 2a. The dipole moment →p is defined as →p=q⋅2a.
- Let point P be located on the equatorial line of the dipole at a distance r from the midpoint of the dipole.
- The distance from the positive charge (+q) to point P is r2+a2 (using the Pythagorean theorem), and similarly, the distance from the negative charge (-q) to point P is also r2+a2.
- The electric field E1 due to the positive charge (+q) at point P is given by:
E1=14πϵ0q(r2+a2)
- The electric field E2 due to the negative charge (-q) at point P is:
E2=14πϵ0q(r2+a2)
- The electric field E1 due to the positive charge points away from the charge, while E2 due to the negative charge points towards the charge. Thus, the two fields will have components that can be resolved.
- The vertical components of E1 and E2 will cancel each other out due to symmetry. Only the horizontal (cosine) components will add up.
- The horizontal component of E1 is E1cosθ and for E2 it is E2cosθ.
- The resultant electric field E at point P is:
E=E1cosθ+E2cosθ=2E1cosθ
- Substituting E1:
E=2(14πϵ0q(r2+a2))cosθ
- Using the geometry of the dipole, cosθ=a√r2+a2:
E=2(14πϵ0q(r2+a2))a√r2+a2
- Rearranging gives:
E=p4πϵ0(r2+a2)3/2
- where p=q⋅2a is the dipole moment.
- When the dipole is aligned with the electric field (θ = 0), the potential energy U is given by:
U=−pEcos(0)=−pE
- This is the minimum potential energy, indicating stable equilibrium.
- When the dipole is aligned opposite to the electric field (θ = π), the potential energy U is:
U=−pEcos(π)=pE
- This represents maximum potential energy, indicating unstable equilibrium.
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-I (SECTION-D)
1 Video
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-I (SECTION-E)
5 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-I (SECTION-B)
5 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
Derive the expression for the electric potential at any point along the axial line of an electric dipole.
View SolutionElectric potential at any point in equatorial plane of a dipole is ………. .
View SolutionThe electric field at a point on equatorial of a dipole and direction of the dipole moment
View SolutionDerive an expression for intensity of electric field are a point in broadside position or on an equatorial line of an electric dipole.
View SolutionThe electric field at a point at a distance r from an electric dipole is proportional to ......
View SolutionObtain an expression for intensity of electric field in end on position, i.e., axial position of an electric dipole.
View SolutionIn which orientation,a diole placed, in a uniform field is in (i) stable (ii) unstable equilibrioum?
View SolutionAt what angle between dipole moment and electric field, electric dipole remains in stable equilibrium in uniform electric field ?
View SolutionIf there is uniform magnetic field of in the positive z direction , in which orientations the loop would be in (i) stable equilibrium and (ii) unstable equilibrium ?
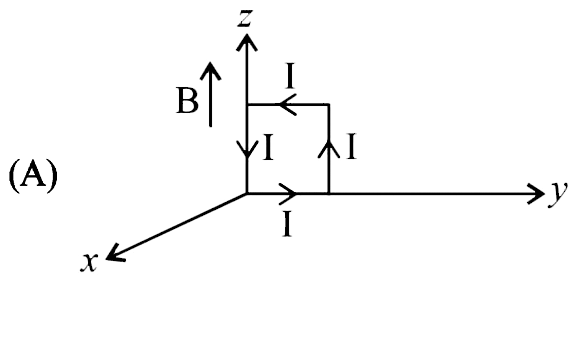
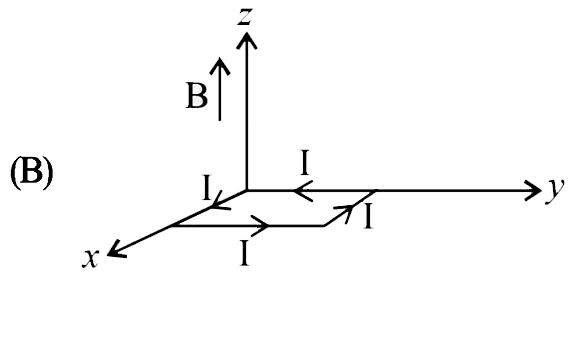
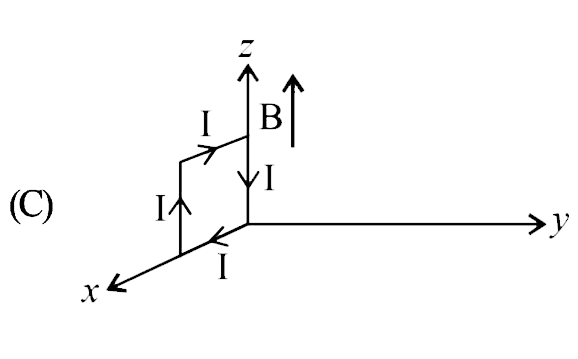
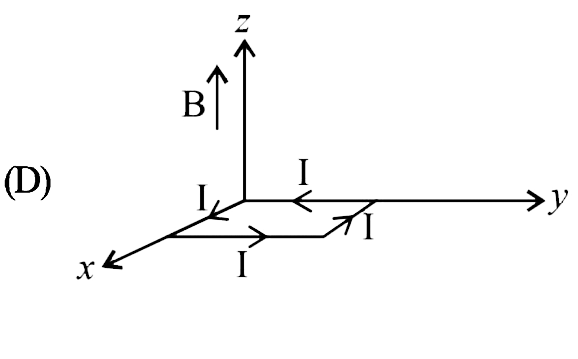
View Solutionwhat is the net force on a dipole in a uniform electric field ?
View Solution
