How does one explain the emission of electron from a photosensitive surface with te help of Einstein's photoelectric equation ?
To explain the emission of electrons from a photosensitive surface using Einstein's photoelectric equation, we can follow these steps:
Step 1: Understanding Photon Energy
The energy of a photon is given by the equation:
E=hν
where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34Js),
- ν is the frequency of the incident light.
Step 2: Absorption of Photon Energy
When a photon strikes a photosensitive surface, it can be absorbed by the electrons in the material. The energy absorbed by the electron is equal to the energy of the photon, E.
Step 3: Work Function
The photosensitive material has a characteristic minimum energy required to release an electron, known as the work function (ϕ0). This is the energy barrier that must be overcome for an electron to be emitted from the surface.
Step 4: Energy Distribution
When the photon energy is absorbed by the electron, it is utilized in two ways:
1. To overcome the work function: This is the minimum energy required to free the electron from the material.
2. To provide kinetic energy to the emitted electron: The remaining energy after overcoming the work function is converted into kinetic energy of the emitted electron.
Step 5: Einstein's Photoelectric Equation
The relationship can be expressed mathematically as:
E=ϕ0+K.E.
where:
- K.E. is the kinetic energy of the emitted electron.
- Rearranging gives us:
K.E.=E−ϕ0
or substituting for E:
K.E.=hν−ϕ0
Step 6: Conclusion
This equation shows that if the energy of the incoming photon (hν) is greater than the work function (ϕ0), electrons will be emitted from the surface with a certain kinetic energy. If hν is less than ϕ0, no electrons will be emitted.
The energy of a photon is given by the equation:
E=hν
where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34Js),
- ν is the frequency of the incident light.
When a photon strikes a photosensitive surface, it can be absorbed by the electrons in the material. The energy absorbed by the electron is equal to the energy of the photon, E.
The photosensitive material has a characteristic minimum energy required to release an electron, known as the work function (ϕ0). This is the energy barrier that must be overcome for an electron to be emitted from the surface.
When the photon energy is absorbed by the electron, it is utilized in two ways:
1. To overcome the work function: This is the minimum energy required to free the electron from the material.
2. To provide kinetic energy to the emitted electron: The remaining energy after overcoming the work function is converted into kinetic energy of the emitted electron.
The relationship can be expressed mathematically as:
E=ϕ0+K.E.
where:
- K.E. is the kinetic energy of the emitted electron.
- Rearranging gives us:
K.E.=E−ϕ0
or substituting for E:
K.E.=hν−ϕ0
This equation shows that if the energy of the incoming photon (hν) is greater than the work function (ϕ0), electrons will be emitted from the surface with a certain kinetic energy. If hν is less than ϕ0, no electrons will be emitted.
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-I (SECTION-D)
1 Video
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-I (SECTION-E)
5 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-I (SECTION-B)
5 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
(a) Why photoelectric effect cannot be explained on the basis of wave nature of light?
(b) Write the basic features of photon picture of electromagnetic radiation on which Einstein's photoelectric equation is based.
View SolutionWhen a high frequency electromagnetic radiation is incident on a metallic surface, electrons are emitted from the surface. Energy of emitted photoelectrons depends only on the frequency of incident electromagnetic radiation and the number of emitted electrons depends only on the intensity of incident light.
Einstein's protoelectron equation [Kmax=hv−ϕ} correctly explains the PE, where υ= frequency of incident light and ϕ= work function.
Q. The slope of the graph between stopping potential and frequency is [here h is the Planck's constant and e is the charge of an electron] is
View Solution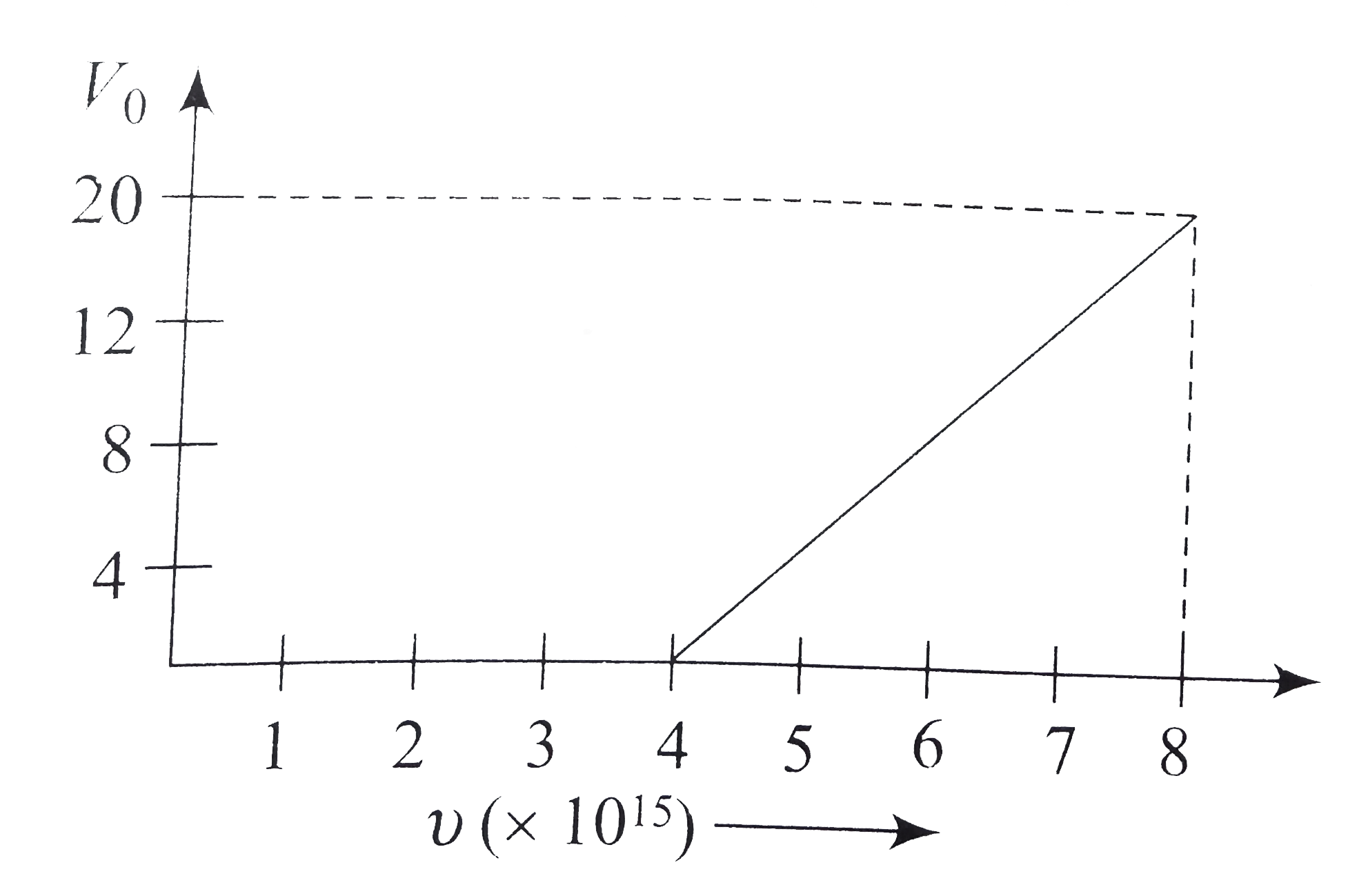
When a high frequency electromagnetic radiation is incident on a metallic surface, electrons are emitted from the surface. Energy of emitted photoelectrons depends only on the frequency of incident electromagnetic radiation and the number of emitted electrons depends only on the intensity of incident light.
Einstein's protoelectron equation [Kmax=hv−ϕ] correctly ecplains the PE, where υ= frequency of incident light and ϕ= work function.
Q. For photoelectric effect in a metal, the graph of the stopping potential V0 (in volt) versus frequency υ (in hertz) of the incident radiation is shown in Fig. The work function of the metal (in eV) is.
View SolutionStatement-1 : Light described at a place by the equation E=E0(sinωt+sin7ωt) falls on a metal surface having work funcation ϕ0. The macimum kinetic energy of the photoelectrons is KEmax=7hω2π−ϕ0
Statement-2 : Maximum kinetic energy of photoelectron depends on the maximum frequency present in the incident light according to Einstein's photoelectric effect equation.
View SolutionUsing Einstein's photoelectric equation show how the cut -off voltage and threshold frequency for a given photosensitive material can be determined with the help of a suitable plot/graph.
View SolutionHow does over exploitation of beneficial species affect biodiversity ? Explain with the help of one example.
View SolutionExplain giving reasons for the following :
(a) Photoelectric current in a photocell increases with the increase in intensity of the incident radiation.
(b) The stopping potential V0 varies linearly with the frequency ν of the incident radiation for a given photosensitive surface with the slope remaining the same for different surfaces.
(c) Maximum kinetic energy of the photoelectrons is independent of the intensity of incident radiation.
View SolutionThe threshold wavelength for ejection of electrons from a metal is 430 nm. The work function for the photoelectric emission from the metal is
View SolutionThe threshold wavelength for ejection of electrons from a metal is 250 nm. The work function for the photoelectric emission from the metal is
View SolutionThe threshold wavelength for ejection of electrons from a metal is 300 nm. The work function for the photoelectric emission from the metal is
View Solution