The grond state energy of hydrogen atom is 13.6eV. If an electron makes a transition
from an energy level - 1.51 eV to - 3.4 e V , calculate the wevelength of the spectrel line
emitted and name the series of hydrogen spectrum to which it belongs.
To solve the problem of calculating the wavelength of the spectral line emitted during the transition of an electron in a hydrogen atom, we will follow these steps:
Step 1: Identify the Energy Levels
We are given two energy levels:
- Initial energy level (E_initial) = -1.51 eV
- Final energy level (E_final) = -3.4 eV
Step 2: Calculate the Energy of the Transition
The energy emitted during the transition can be calculated using the formula:
Ephoton=Einitial−Efinal
Substituting the values:
Ephoton=(−1.51eV)−(−3.4eV)
Ephoton=−1.51+3.4
Ephoton=1.89eV
Step 3: Use the Energy-Wavelength Relationship
The energy of a photon is related to its wavelength by the equation:
E=hcλ
Where:
- E = energy of the photon (in joules)
- h = Planck's constant (6.626×10−34Js)
- c = speed of light (3×108m/s)
- λ = wavelength (in meters)
First, we need to convert the energy from eV to joules:
1eV=1.6×10−19J
Thus,
Ephoton=1.89eV=1.89×1.6×10−19J
Ephoton≈3.024×10−19J
Step 4: Calculate the Wavelength
Now, we can rearrange the energy-wavelength relationship to find the wavelength:
λ=hcE
Substituting the values:
λ=(6.626×10−34Js)(3×108m/s)3.024×10−19J
λ≈1.9878×10−253.024×10−19
λ≈6.57×10−7m
λ≈657nm
Step 5: Identify the Series
The transition from n=3 to n=2 corresponds to the Balmer series of the hydrogen spectrum, which involves transitions to the second energy level.
Final Answer
The wavelength of the spectral line emitted is approximately 657 nm and it belongs to the Balmer series of the hydrogen spectrum.
---
We are given two energy levels:
- Initial energy level (E_initial) = -1.51 eV
- Final energy level (E_final) = -3.4 eV
The energy emitted during the transition can be calculated using the formula:
Ephoton=Einitial−Efinal
Ephoton=(−1.51eV)−(−3.4eV)
Ephoton=−1.51+3.4
Ephoton=1.89eV
The energy of a photon is related to its wavelength by the equation:
E=hcλ
Where:
- E = energy of the photon (in joules)
- h = Planck's constant (6.626×10−34Js)
- c = speed of light (3×108m/s)
- λ = wavelength (in meters)
1eV=1.6×10−19J
Thus,
Ephoton=1.89eV=1.89×1.6×10−19J
Ephoton≈3.024×10−19J
Now, we can rearrange the energy-wavelength relationship to find the wavelength:
λ=hcE
λ=(6.626×10−34Js)(3×108m/s)3.024×10−19J
λ≈1.9878×10−253.024×10−19
λ≈6.57×10−7m
λ≈657nm
The transition from n=3 to n=2 corresponds to the Balmer series of the hydrogen spectrum, which involves transitions to the second energy level.
The wavelength of the spectral line emitted is approximately 657 nm and it belongs to the Balmer series of the hydrogen spectrum.
Topper's Solved these Questions
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION - C15videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION - D3videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - [SET -II, OUTSIDE DELHI]6videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D6videos
SAMPLE PAPER 2019
Similar Questions
The ground state energy of hydrogen atom is -13.6eV. If an electron makes a transition from an energy level -0.85 eV to -1.51 eV, calculate the wavelength of spectral line emitted. To which series of hydrogen spectrum does this wavelength belongs?
View SolutionThe ground state energy of hydrogen atom is −13.6eV. If an electron makes a transition form an energy level −0.85eV to −3.4eV, calculate the wavelength of spectral line emitted. To which series of hydrogen spectrum does this wavelength belongs?
View Solution
Knowledge Check
- Question 1 - Select One
The ground state energy of hydrogen atom is -13.6eV. What is the K.E. of electron in this state?
A2.18×10−14JB2.18×10−16JC2.18×10−18JD2.18×10−19J
Excitations energy of hydrogen atom is 13.6eV match the following
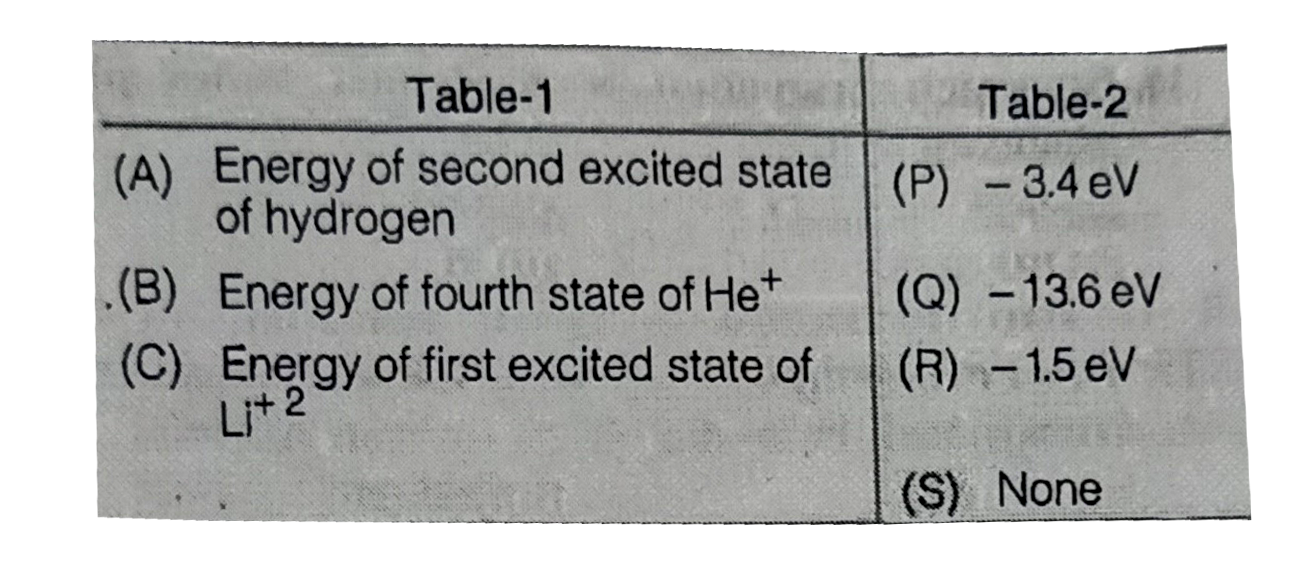 View Solution
View SolutionThe groud state energy of hydrogen atom is −13.6eV. When its electron is in first excited state, its exciation energy is
View SolutionThe ground state energy of hydrogen atom is −13.6eV. The energy of second excited state of He+ ion in eV is
View SolutionThe ground state energy of hydrogen atom is −13.6eV. What is the potential energy of the electron in this state
View SolutionThe ground state energy of hydrogen atom is −13.6eV. If the electron jumps to the ground state from the 3rd excited state, the wavelength of the emitted photon is
View SolutionThe ionization energy of hydrogen atom is 13.6 eV. Calculate Rydberg's constant for hydrogen.
View SolutionGiven that ionization energy of hydrogen atom is 13.6 eV, calculate the wavelength of first line of the Lyman series.
View Solution

