Draw a triangle PQR with base QR = 6 cm, vertical angle P = 60° and median through P to the base is of length 4.5 cm.
To construct triangle PQR with the given conditions, follow these steps:
1. Draw Base QR:
- Draw a straight line segment QR measuring 6 cm. Label the endpoints as Q and R.
Hint: Use a ruler to ensure the line is straight and measure accurately.
2. Find the Midpoint K of QR:
- To find the midpoint K, use a compass to draw arcs above and below the line segment QR from points Q and R. The distance from Q to K and K to R should be equal. Mark the intersection points of the arcs above and below the line and draw a line through these intersection points. This line will intersect QR at point K.
Hint: Ensure the compass width is more than half the length of QR for accurate arcs.
3. Draw the Median PK:
- From point K, measure 4.5 cm using a compass and draw an arc above the line QR. Label this point as P.
Hint: Keep the compass point on K and adjust the pencil side to 4.5 cm before drawing the arc.
4. Construct Angle P:
- At point P, use a protractor to measure a 30° angle on both sides of the line segment PK. Mark these angles as points A and B, where PA and PB are the lines extending from P.
Hint: Make sure the protractor is aligned correctly with line PK to measure the angles accurately.
5. Draw Lines PQ and PR:
- Draw straight lines from point P to points Q and R, creating triangle PQR.
Hint: Use a ruler to connect the points accurately and ensure the lines are straight.
6. Verify the Triangle:
- Check that the angle at P (angle QPR) is 60° and that the median PK is indeed 4.5 cm.
Hint: Use a protractor to measure angle QPR and a ruler to verify the length of PK.
Final Result:
You should now have triangle PQR with base QR = 6 cm, vertical angle P = 60°, and median PK = 4.5 cm.
- Draw a straight line segment QR measuring 6 cm. Label the endpoints as Q and R.
- To find the midpoint K, use a compass to draw arcs above and below the line segment QR from points Q and R. The distance from Q to K and K to R should be equal. Mark the intersection points of the arcs above and below the line and draw a line through these intersection points. This line will intersect QR at point K.
- From point K, measure 4.5 cm using a compass and draw an arc above the line QR. Label this point as P.
- At point P, use a protractor to measure a 30° angle on both sides of the line segment PK. Mark these angles as points A and B, where PA and PB are the lines extending from P.
- Draw straight lines from point P to points Q and R, creating triangle PQR.
- Check that the angle at P (angle QPR) is 60° and that the median PK is indeed 4.5 cm.
You should now have triangle PQR with base QR = 6 cm, vertical angle P = 60°, and median PK = 4.5 cm.
Topper's Solved these Questions
Similar Questions
Construct a triangle PQR in which QR = 6cm, ∠Q=60∘ and PR – PQ = 2cm.
View SolutionConstruct a triangle PQR with PQ = 4 cm, ∠P=75∘,and∠R=65∘.
View SolutionConstruct a triangle PQR with PQ = 9 cm, ∠P=55∘,∠R=35∘.
View SolutionConstruct a triangle PQR with sides PQ = 5 cm, QR = 4 cm, and ∠PQR=100∘.
View SolutionDraw a triangle ABC with side BC = 6 cm, AB = 5 cm and ∠ABC=60∘. Then construct a triangle whose sides are 34 of the corresponding sides of the triangle ABC.
View SolutionConstruct a triangle PQR given that PQ = 6 . 2 cm ∠P=60∘and∠Q=45∘ by using ruler and compass only. Measure ∠R
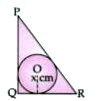
View SolutionIn triangle PQR, PQ = 24 cm, QR = 7 cm and ∠PQR=90∘. Find the radius of the inscribed circle.
View SolutionDraw Δ ABC, with AB = 5 cm, BC = 8 cm, and ∠B=30∘. Draw the medians through A and B. Mark the point of intersection of the median as P. Join CP and extend it to meet AB at T. Measure TA and TB.
View SolutionConstruct a triangle PQR having PQ=6 cm,∠P=∠R=60∘ and draw all possible lines of symmetry.
View SolutionThe area of a triangle with base 4 cm and height 6 cm is 24 cm2.
View Solution
