A group consists of 12 persons, of which 3 are extremely patient, other 6 are extremely honest and rest are extremely kind. A person from the group is selected at random. Assuming that each person is equally likely to be selected, find the probability of selecting a person who is (i) extremely patient (ii) extremely kind or honest. Which of the above values you prefer
To solve the problem, we will follow these steps:
Step 1: Understand the total number of persons in the group.
The total number of persons in the group is given as 12.
Step 2: Identify the number of persons in each category.
- Extremely patient: 3 persons
- Extremely honest: 6 persons
- Extremely kind: To find this, we subtract the sum of extremely patient and extremely honest from the total:
Extremely kind=Total persons−(Extremely patient+Extremely honest)=12−(3+6)=3
Step 3: Calculate the probability of selecting a person who is extremely patient.
The probability P(Patient) is calculated as:
P(Patient)=Number of extremely patient personsTotal number of persons=312=14
Step 4: Calculate the probability of selecting a person who is extremely kind or honest.
To find this probability, we add the number of extremely kind and extremely honest persons:
Number of extremely kind or honest=Extremely kind+Extremely honest=3+6=9
Now calculate the probability P(Kind or Honest):
P(Kind or Honest)=Number of extremely kind or honest personsTotal number of persons=912=34
Step 5: Compare the probabilities.
We have:
- P(Patient)=14
- P(Kind or Honest)=34
Since 34>14, we conclude that selecting a person who is extremely kind or honest is preferred.
Final Answer:
1. Probability of selecting a person who is extremely patient: 14
2. Probability of selecting a person who is extremely kind or honest: 34
3. Preferred value: Extremely kind or honest.
---
The total number of persons in the group is given as 12.
- Extremely patient: 3 persons
- Extremely honest: 6 persons
- Extremely kind: To find this, we subtract the sum of extremely patient and extremely honest from the total:
Extremely kind=Total persons−(Extremely patient+Extremely honest)=12−(3+6)=3
The probability P(Patient) is calculated as:
P(Patient)=Number of extremely patient personsTotal number of persons=312=14
To find this probability, we add the number of extremely kind and extremely honest persons:
Number of extremely kind or honest=Extremely kind+Extremely honest=3+6=9
Now calculate the probability P(Kind or Honest):
P(Kind or Honest)=Number of extremely kind or honest personsTotal number of persons=912=34
We have:
- P(Patient)=14
- P(Kind or Honest)=34
1. Probability of selecting a person who is extremely patient: 14
2. Probability of selecting a person who is extremely kind or honest: 34
3. Preferred value: Extremely kind or honest.
Topper's Solved these Questions
Similar Questions
A group consists of 12 persons, of which 3 are extremely patient, other 6 are extremely honest and rest are extremely kind. A person from the group is selected at random. Assuming that each person is equally likely to be selected, find the probability of selecting a person who is (i) extremely patient (ii) extremely kind or honest. Which of the above you prefer more.
View SolutionJohn invites 12 friends to a dinner party, half of whom are men. Exactly one man and one woman are bringing desserts. If one person from this group is selected at random, what is the probability that it is a man who is not bringing a dessert OR that it is a woman?
View Solution
Knowledge Check
Exactly 70 percent of the people in each of 3 rooms are seniors at university X. If one person is selected at random from each of the the 3 rooms, what is the probability that each of the 3 peple selected is a senior at university X ?
A0.2401B0.343C0.49D0.64
In a group there are 2 men and 3 women ,3 persons are selected at random from the group . Find the probability that 1 man and 2 women or 2 men and 1 women are selected .
View SolutionOut of a group of 30 honest people, 20 always speak the truth. Two persons are selected at random from the group. Find the probability distribution of the number of selected persons who speak the truth. Also find the mean of the distribution
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
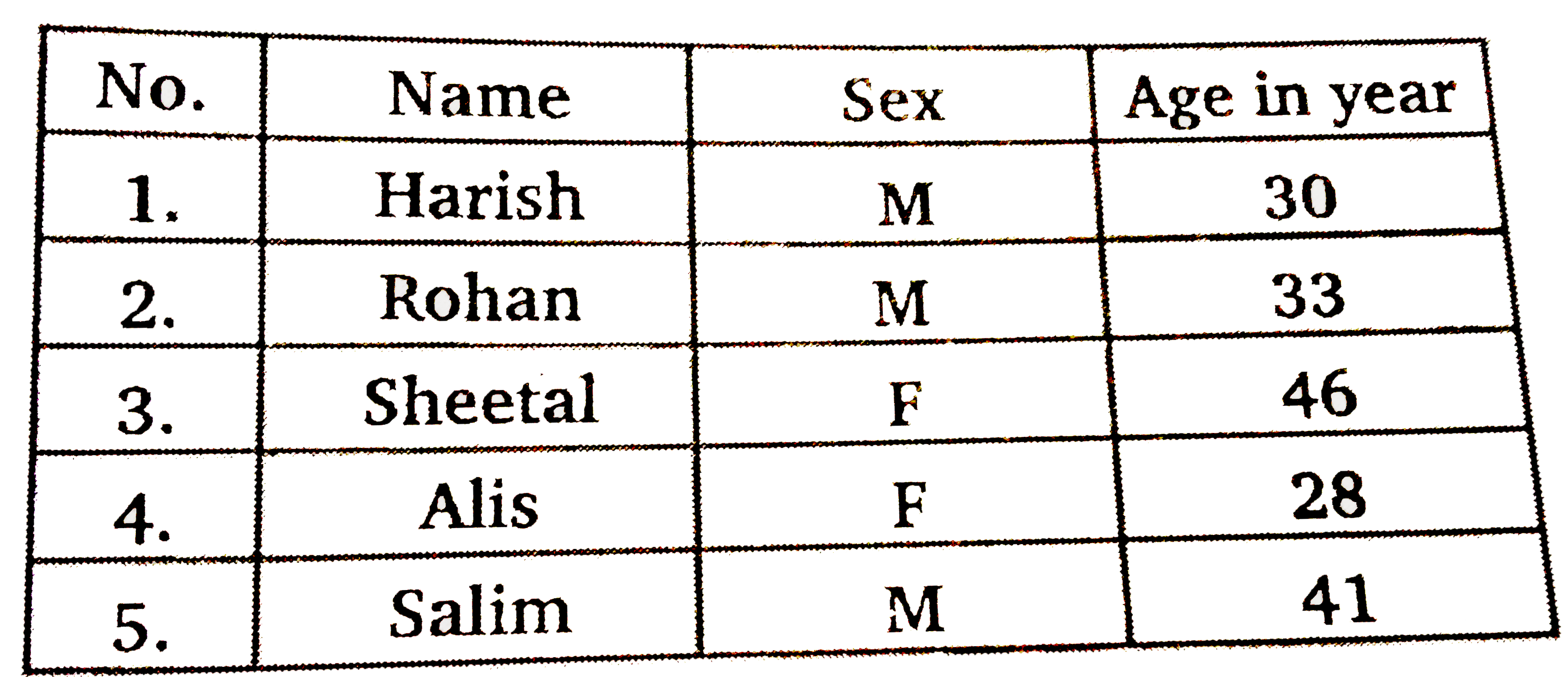
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?
View SolutionIn a group of 50 scouts in a camp, 30 are well trained in first aid techniques while the remaining are well trained in hospitality but not in first aid. Two scouts are selected at random from the group. Find the probability distribution of number of selected scouts who are well trained in first aid. Find the mean of the distribution also. Write one more value which is expected from a well trained scout.
View SolutionA lot consists of 48 mobile phones of which 42 are good, 3 have only minor defected and 3 have major defects. Varnika will buy a phone if its is good but the trader will only buy a mobile, if it has no major defect. One phone is selected at random from the lot. What is the probability that t is
(i) accetable to Varnika?
(ii) acceptable to the trader.
View SolutionFrom a group of 2 men and 3 women , two persons are selected . Describe the sample space of the experiment , if E is the event in which one man and one women are selected , then which are the cases favourable to E?
View SolutionA room has 3 lamps . From a collection of 10 light bulbs of which 6 are no good , a person selects 3 at random and puts them in a socket . What is the probability , that he will have light ?
View Solution
