AP, AQ and BC are tangents to the circle. If AB = 5 cm, AC = 6 cm and BC = 4 cm, then the length of AP (in cm) is
To solve the problem, we need to find the length of AP given that AB=5cm, AC=6cm, and BC=4cm.
1. Understanding the Problem:
We have a circle with points A, B, and C outside the circle. The segments AP, AQ, and BC are tangents to the circle from points A, B, and C.
2. Draw the Figure:
Draw a circle and label the points A, B, and C outside the circle. Draw the tangents AP, AQ, and BC from points A, B, and C respectively.
3. Using Tangent Properties:
Since AP and AQ are tangents from point A to the circle, we have:
AP=AQ
Let AP=AQ=x.
4. Using the Given Lengths:
We know:
- AB=5cm
- AC=6cm
- BC=4cm
5. Perimeter of Triangle ABC:
The perimeter of triangle ABC can be expressed as:
AB+AC+BC=5+6+4=15cm
6. Expressing Perimeter in Terms of Tangents:
The perimeter can also be expressed in terms of the tangents:
AB+BP+CQ+AC=15cm
Here, BP=BD and CQ=CD.
7. Substituting Known Values:
We can substitute the known lengths:
5+BP+CQ+6=15
Simplifying gives:
BP+CQ=15−11=4cm
8. Using the Tangent Properties Again:
Since BP=BD and CQ=CD, we can express BP and CQ in terms of x:
BP=xandCQ=x
Therefore:
x+x=4
This simplifies to:
2x=4
9. Finding x:
Solving for x:
x=42=2cm
10. Conclusion:
Thus, the length of AP is:
AP=2cm
Final Answer:
The length of AP is 2cm.
We have a circle with points A, B, and C outside the circle. The segments AP, AQ, and BC are tangents to the circle from points A, B, and C.
Draw a circle and label the points A, B, and C outside the circle. Draw the tangents AP, AQ, and BC from points A, B, and C respectively.
Since AP and AQ are tangents from point A to the circle, we have:
AP=AQ
Let AP=AQ=x.
We know:
- AB=5cm
- AC=6cm
- BC=4cm
The perimeter of triangle ABC can be expressed as:
AB+AC+BC=5+6+4=15cm
The perimeter can also be expressed in terms of the tangents:
AB+BP+CQ+AC=15cm
Here, BP=BD and CQ=CD.
We can substitute the known lengths:
5+BP+CQ+6=15
Simplifying gives:
BP+CQ=15−11=4cm
Since BP=BD and CQ=CD, we can express BP and CQ in terms of x:
BP=xandCQ=x
Therefore:
x+x=4
This simplifies to:
2x=4
Solving for x:
x=42=2cm
Thus, the length of AP is:
AP=2cm
The length of AP is 2cm.
Topper's Solved these Questions
Similar Questions
In the given figure, AB, BC and CA are tangents to the given circle. If AB= 12cm, BC= 8cm and AC= 10cm, find the length of AD,BE=CF
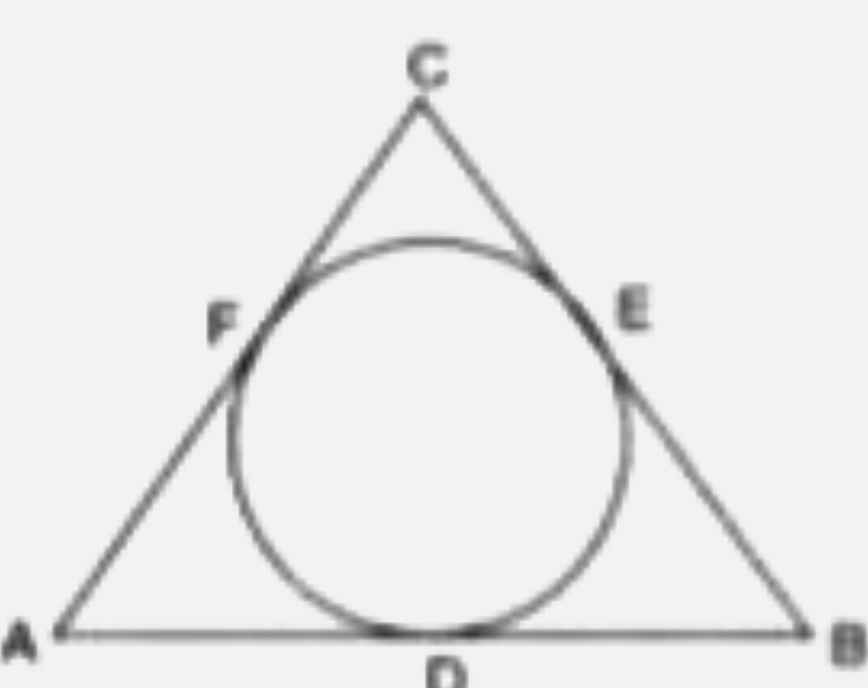
View SolutionIn the adjoining figure, AB=8cm,DM=6cmandBC=6cm. Find the length of DN
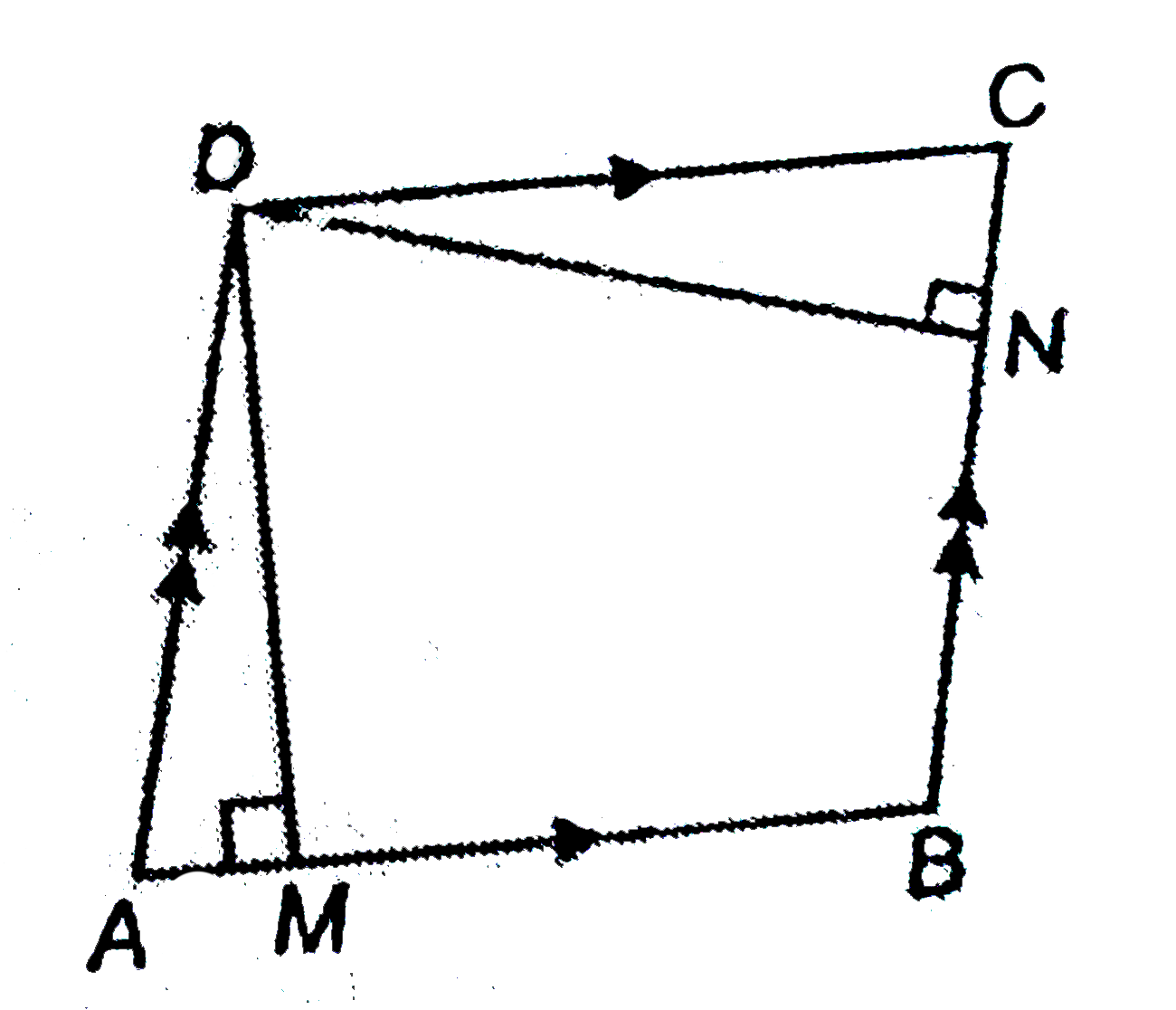
View SolutionIn triangle ABC, given below, AB = 8 cm, BC = 6 cm and AC = 3 cm. Calculate the length of OC

View SolutionABCD is a trapezium such that AB and DC are parallel and BC is perpendicular to them. If BC=1 cm, CD=2 cm and ∠ADB=45∘, then the lengths of AB is (in cm)
View SolutionA line PQ is drawn parallel to the side BC of ΔABC which cuts side AB at P and side AC at Q. If AB = 90 cm, CA = 60 cm and AQ = 4.2 cm, find the length of AP.
View SolutionΔABC is right-angled at C. If AC=5cmandBC=12cm find the length of AB.
View SolutionIn the figure, EF || AC, BC = 10 cm, AB = 13 cm and EC = 2 cm, find AF
View SolutionConstruct a triangle ABC given that :
AB = 5 cm, BC = 4 cm and CA = 6 cm
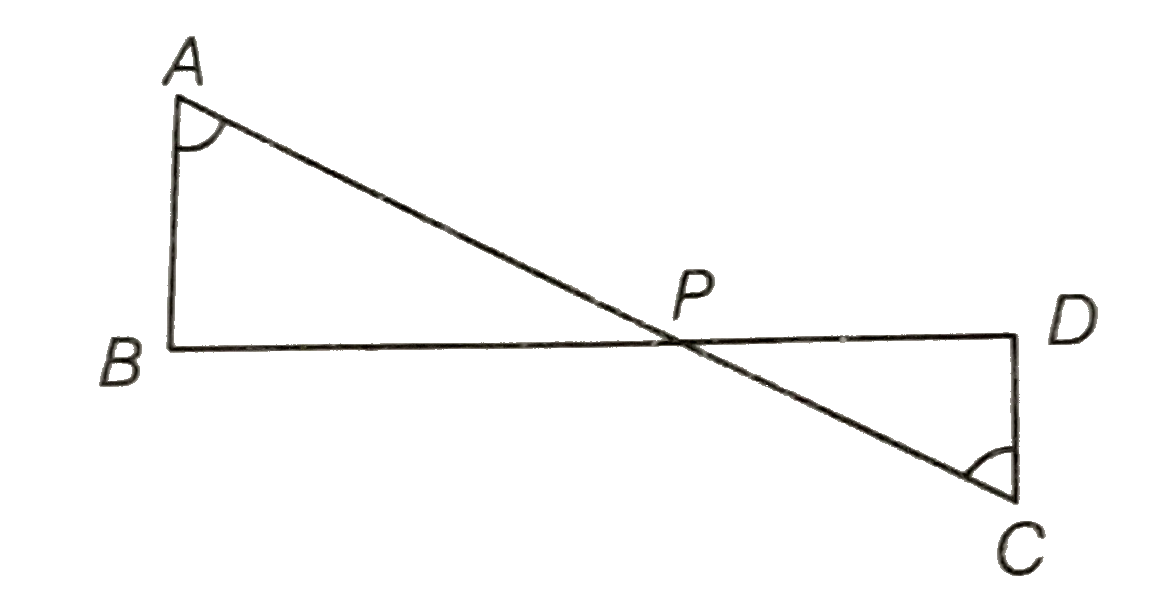
View SolutionIn given figure, if ∠A=∠C, AB= 6 cm, BP = 15 cm, AP = 12 cm and CP= 4 cm, then find the lengths of PD and CD.
View SolutionConstruct an Δ ABC with sides AB = 5 cm, BC = 5.5 cm, and CA = 6 cm.
View Solution
