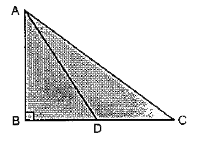
In a triangle ABC,B=90∘ and D is the mid-oint of BC then prove that AC2=AD2+3CD2
To prove that in triangle ABC where B=90∘ and D is the midpoint of BC, the relation AC2=AD2+3CD2 holds, we can follow these steps:
Step 1: Understand the given information
We have triangle ABC with B as the right angle, and D is the midpoint of side BC. This means that BD=DC=CD.
Step 2: Apply the Pythagorean theorem in triangle ABC
Using the Pythagorean theorem in triangle ABC:
AC2=AB2+BC2
Since D is the midpoint of BC, we can express BC in terms of CD:
BC=2CD
Substituting this into the Pythagorean theorem gives:
AC2=AB2+(2CD)2
This simplifies to:
AC2=AB2+4CD2(Equation 1)
Step 3: Apply the Pythagorean theorem in triangle ABD
Now, we apply the Pythagorean theorem in triangle ABD:
AD2=AB2+BD2
Since D is the midpoint of BC, we have BD=CD. Therefore, we can replace BD with CD:
AD2=AB2+CD2(Equation 2)
Step 4: Subtract Equation 2 from Equation 1
Now, we will subtract Equation 2 from Equation 1:
AC2−AD2=(AB2+4CD2)−(AB2+CD2)
This simplifies to:
AC2−AD2=4CD2−CD2
AC2−AD2=3CD2
Step 5: Rearranging the equation
Rearranging the equation gives us:
AC2=AD2+3CD2
Conclusion
Thus, we have proved that:
AC2=AD2+3CD2
We have triangle ABC with B as the right angle, and D is the midpoint of side BC. This means that BD=DC=CD.
Using the Pythagorean theorem in triangle ABC:
AC2=AB2+BC2
Since D is the midpoint of BC, we can express BC in terms of CD:
BC=2CD
Substituting this into the Pythagorean theorem gives:
AC2=AB2+(2CD)2
This simplifies to:
AC2=AB2+4CD2(Equation 1)
Now, we apply the Pythagorean theorem in triangle ABD:
AD2=AB2+BD2
Since D is the midpoint of BC, we have BD=CD. Therefore, we can replace BD with CD:
AD2=AB2+CD2(Equation 2)
Now, we will subtract Equation 2 from Equation 1:
AC2−AD2=(AB2+4CD2)−(AB2+CD2)
This simplifies to:
AC2−AD2=4CD2−CD2
AC2−AD2=3CD2
Rearranging the equation gives us:
AC2=AD2+3CD2
Thus, we have proved that:
AC2=AD2+3CD2
Topper's Solved these Questions
Similar Questions
In the given figure, triangle ABC is a right triangle with ∠B=90∘andD is mid-point of side BC. Prove that :
AC2=AD2+3CD2
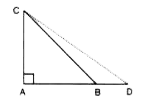
View SolutionIn right-angled triangle ABC in which ∠C=90∘ , if D is the mid-point of BC , prove that AB2=4 AD2−3 AC2 .
View SolutionIn Fig. 3, ABC is a right triangle, right angled at C and D is the mid-point of BC. Prove that AB2=4AD2−3AC2.
View SolutionIn an isosceles triangle ABC, AB = AC and D is a point on BC produced. Prove that :
AD2=AC2+BD.CD
View SolutionIn a right-angled triangle ABC,∠ABC=90∘ and D is mid-point of AC. Prove that BD=12AC.
View SolutionABC is a triangle in which AB = AC and D is any point on BC. Prove that:
AB2−AD2=BD.CD
View SolutionIn a triangle ABC,D is the mid - point of BC, AD is produced upto E so that DE = AD.
Prove that :
AB=EC
View SolutionIn a △ ABC,AB=2,BC=4,CA=3. If D is the mid-point of BC, then the correct statement(s) is/are
View SolutionIn triangle ABC, angle A=90∘,CA=AB and D is a point on AB produced. Prove that:
DC2−BD2=2AB.AD.
View SolutionIn an acute angled triangle ABC, AD is the median in it. then :
AD2 =
View Solution
