All concentric circles are..... to each other.
Solution:
1. Understanding Concentric Circles: Concentric circles are circles that share the same center but have different radii. For example, if we have two circles, Circle A and Circle B, both centered at point O, they are concentric.
2. Identifying Properties: Since concentric circles share the same center, the distance from the center to any point on the circumference of each circle is different. However, the angles subtended at the center by any two points on the circumference of the circles are the same.
3. Conclusion: Given that all concentric circles have the same center and maintain a proportional relationship in their dimensions, we can conclude that all concentric circles are similar to each other.
Thus, the complete statement is: "All concentric circles are similar to each other."
---
Similar Questions
Knowledge Check
All radii of a circle are .....
AUnequalBequalCwe cannot sayDNone of theseA and B are centers of two circles that touch each other externally, as shown in the figure.What is the area of the circle whose diameter is AB?
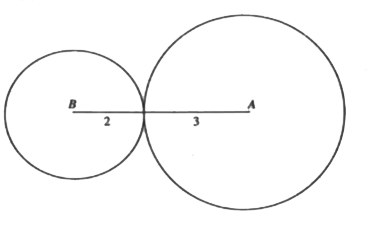 A4πB25π/4C9πD16π
A4πB25π/4C9πD16π
If the radii of two concentric circles are 4 cm and 5 cm, then find the length of each chord of one circle which is tangent to the other circle.
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View SolutionOrder of the differential equation of the family of all concentric circles centred at (h,k) is
View SolutionIn two concentric circles prove that all chords of the outer circle which touch the inner circle are of equal length.
View SolutionProve that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
View Solution