All concentric circles are..... to each other.
To solve the question, we need to fill in the blank in the sentence: "All concentric circles are ______ to each other."
1. Understand the Definition of Concentric Circles:
- Concentric circles are circles that share the same center but have different radii.
2. Analyze the Relationship Between Concentric Circles:
- Since all concentric circles have the same center, they are similar in shape. Similar shapes have the same angles and their corresponding sides are in proportion.
3. Fill in the Blank:
- Given that concentric circles are similar in shape due to having the same center, we can conclude that the appropriate word to fill in the blank is "similar".
4. Final Sentence:
- Therefore, the completed sentence is: "All concentric circles are similar to each other."
- Concentric circles are circles that share the same center but have different radii.
- Since all concentric circles have the same center, they are similar in shape. Similar shapes have the same angles and their corresponding sides are in proportion.
- Given that concentric circles are similar in shape due to having the same center, we can conclude that the appropriate word to fill in the blank is "similar".
- Therefore, the completed sentence is: "All concentric circles are similar to each other."
Similar Questions
Knowledge Check
All radii of a circle are .....
AUnequalBequalCwe cannot sayDNone of theseA and B are centers of two circles that touch each other externally, as shown in the figure.What is the area of the circle whose diameter is AB?
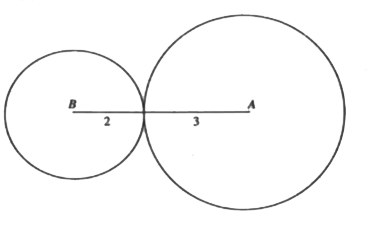 A4πB25π/4C9πD16π
A4πB25π/4C9πD16π
If the radii of two concentric circles are 4 cm and 5 cm, then find the length of each chord of one circle which is tangent to the other circle.
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View SolutionOrder of the differential equation of the family of all concentric circles centred at (h,k) is
View SolutionIn two concentric circles prove that all chords of the outer circle which touch the inner circle are of equal length.
View SolutionProve that in two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
View Solution
