For each natural number k, let Ck denotes the circle radius k centimeters in the counter-clockwise direction.After completing its motion on Ck, the particle moves to Ck+1 in the radial direction. The motion of the particle continues in this manner. The particle starts at (1,0).If the particle crosses the the positive direction of the x-axis for first time on the circle Cn,then n equal to
To solve the problem step by step, let's break down the motion of the particle and find the value of n when it crosses the positive direction of the x-axis for the first time on the circle Cn.
Step 1: Understanding the Motion
The particle starts at the point (1, 0) on the circle C1 with a radius of 1 cm. It moves in a counter-clockwise direction along the circumference of the circle.
Step 2: Calculate the Angles
The angle θk for each circle can be calculated using the formula:
θk=arc lengthradius.
For each circle Ck:
- For C1: θ1=11=1 radian.
- For C2: θ2=22=1 radian.
- For C3: θ3=33=1 radian.
- Continuing this pattern, we see that θk=1 radian for each circle Ck.
Step 3: Total Angle Calculation
The total angle after completing the motion on circles C1,C2,…,Cn is:
Total angle=θ1+θ2+θ3+…+θn=n⋅1=n radians.
Step 4: Condition for Crossing the X-axis
The particle crosses the positive direction of the x-axis when the total angle is a multiple of 2π:
n=2πkfor some integer k.
The smallest positive n that satisfies this condition is when k=1:
n=2π≈6.28.
Step 5: Finding the Integer Value of n
Since n must be a natural number, we round 6.28 up to the next whole number:
n=7.
Conclusion
Thus, the particle crosses the positive direction of the x-axis for the first time on the circle Cn when n=7.
The particle starts at the point (1, 0) on the circle C1 with a radius of 1 cm. It moves in a counter-clockwise direction along the circumference of the circle.
The angle θk for each circle can be calculated using the formula:
θk=arc lengthradius.
For each circle Ck:
- For C1: θ1=11=1 radian.
- For C2: θ2=22=1 radian.
- For C3: θ3=33=1 radian.
- Continuing this pattern, we see that θk=1 radian for each circle Ck.
The total angle after completing the motion on circles C1,C2,…,Cn is:
Total angle=θ1+θ2+θ3+…+θn=n⋅1=n radians.
The particle crosses the positive direction of the x-axis when the total angle is a multiple of 2π:
n=2πkfor some integer k.
The smallest positive n that satisfies this condition is when k=1:
n=2π≈6.28.
Since n must be a natural number, we round 6.28 up to the next whole number:
n=7.
Thus, the particle crosses the positive direction of the x-axis for the first time on the circle Cn when n=7.
Topper's Solved these Questions
Similar Questions
The velocity of a particle moving in the positive direction of the X-axis varies as V=K√S where K is a positive constant. Draw V-t graph.
View SolutionA particle moving along the X-axis executes simple harmonic motion, then the force acting on it is given by
where, A and K are positive constants.
View SolutionA particle is moving on a circle of radius R such that at every instant the tangential and radial accelerations are equal in magnitude. If the velocity of the particle be v0 at t=0, the time for the completion of the half of the first revolution will be
View SolutionThe position of a particle moving along x-axis varies eith time t as x=4t−t2+1. Find the time interval(s) during which the particle is moving along positive x-direction.
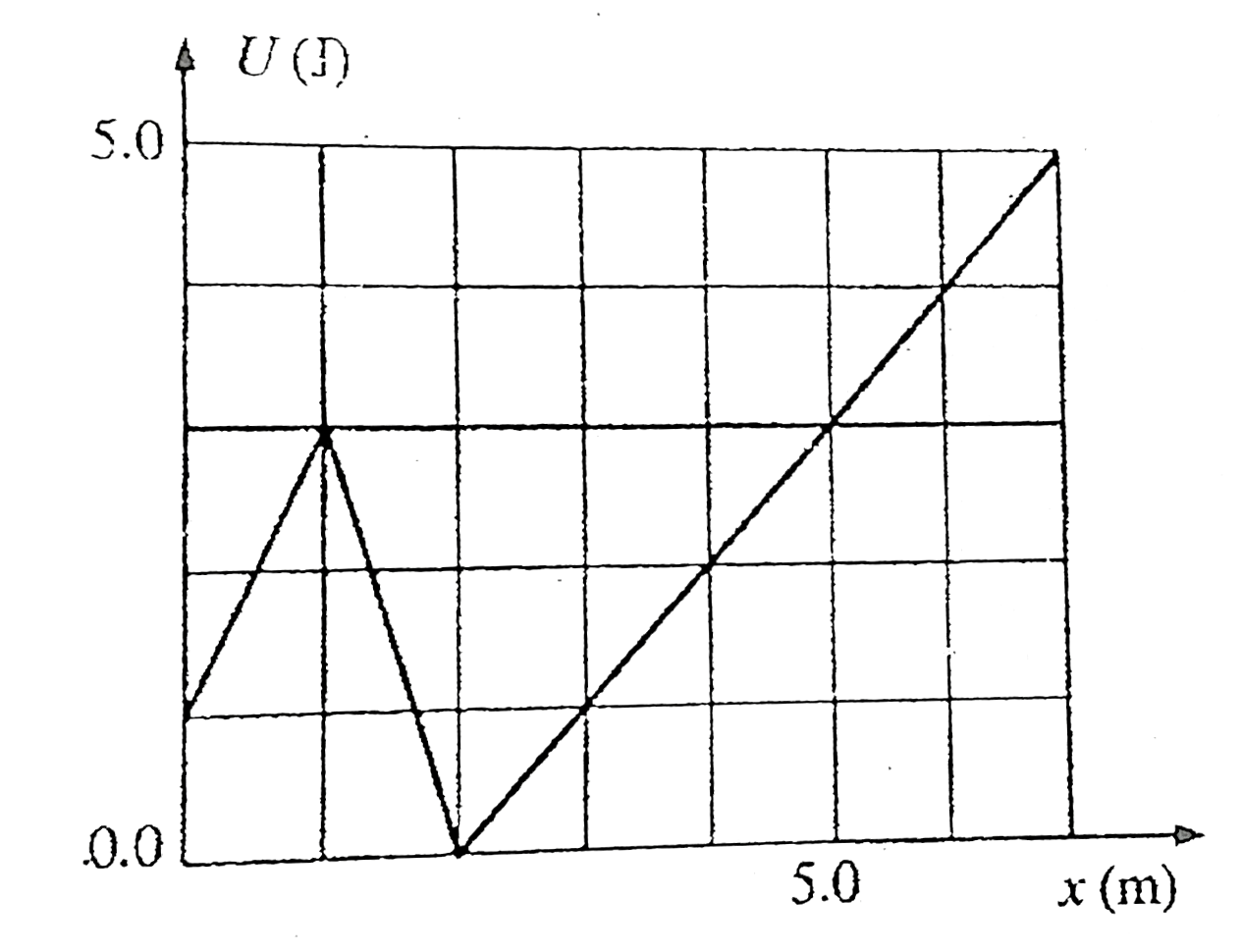
View SolutionA particle with the potentila energy shown in the graph is moving to the right. It has 1.0J of kinetic energy at x=1.0m. Where the particle reverses its direction of motion?
View SolutionA particle of mass m moves along a horizontal circle of radius R such that normal acceleration of particle varies with time as an=kt2. where k is a constant.
Total force on particle at time t s is
View SolutionA force F=−K(yˆi+xˆj) (where K is a positive constant) acts on a particle moving in the x-y plane. Starting from the origin, the particle is taken along the positive x-axis to the point (a,0), and then parallel to the y-axis to the point (a,a). The total work done by the force F on the particle is
View SolutionA force F=−kx2(x≠0) acts on a particle in x-direction. Find the work done by this force in displacing the particle from. x=+a to x=+2a. Where, k is a positive constant.
View SolutionA force F=−kx2(x≠0) acts on a particle in x-direction. Find the work done by this force in displacing the particle from. x=+a to x=2a. Here, k is a positive constant.
View SolutionA particle of mass m moves along a horizontal circle of radius R such that normal acceleration of particle varies with time as an=kt2. where k is a constant.
Tangential force on particle at t s is
View Solution