Find the number of solutions of sinx=x10
Video Solution
Text Solution
Here, let f(x)=sinx and g(x)=x10.
Also, we know that −1≤sinx≤1 or −1≤x10≤1 or −10≤x≤10
Now, sketch both the curves when x∈[−10,10].
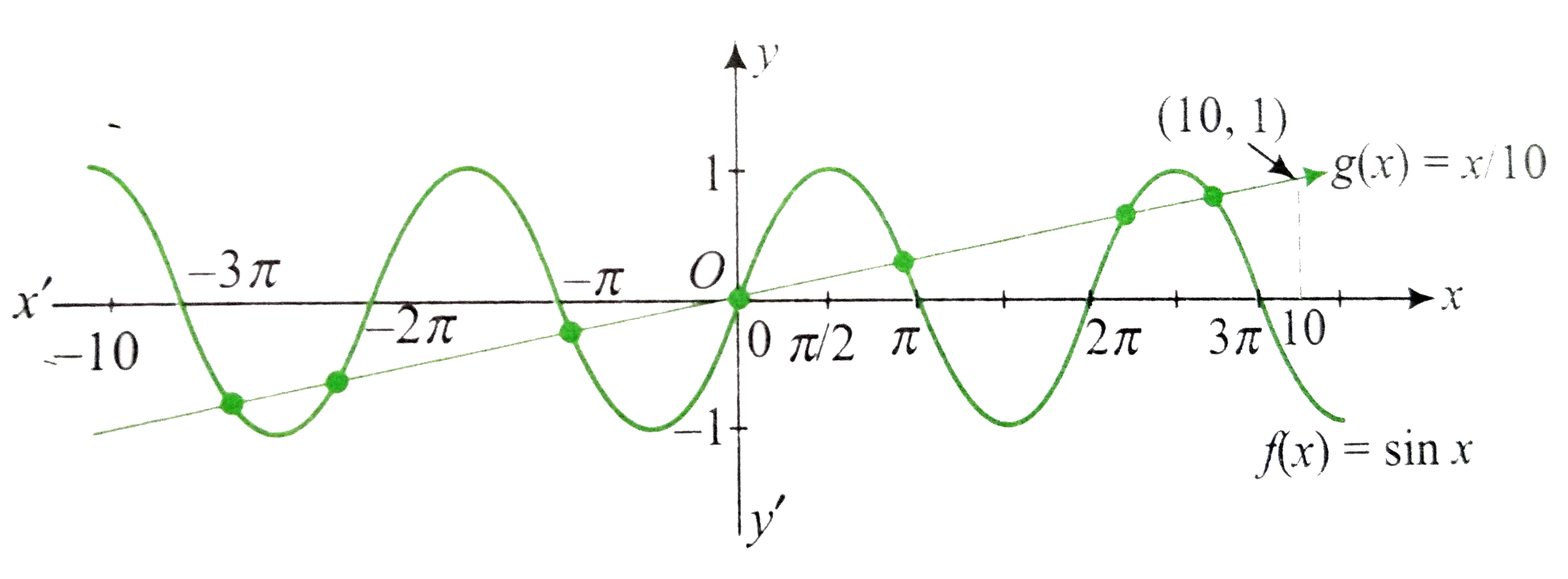
From the figure, f(x)=sinx and g(x)=x10 intersect at 7 points.
So, number of solutions is 7.
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Find the number of solutions of sin2x−sinx−1=0∈[−2π,2π]
View SolutionFind the number of solutions to cosx=x10,x>0.
View SolutionFind the number of solution of [cosx]+∣sinx=1∈π≤x≤3π where [] denotes the greatest integer function.
View SolutionFind the total number of solutions of sinπx=|ln|x∣∣
View SolutionFind the number of solutions of 4{x}=x+[x].
View SolutionTotal number of solutions of sinx=|x|10 is equal to
View SolutionFind the number of solutions of
2|x|=sinx2View SolutionFind the number of solutions of 2cosx=|sinx| when x∈[0,4π].
View SolutionFind the number of solution of [cosx]+|sinx|=1,x∈π≤x≤3π (where [ ] denotes the greatest integer function).
View SolutionFind the number of solutions of |x|⋅3|x|=1.
View Solution

