Show that there exists no polynomial $f (x)$ with integral coefficients which satisfy $f (a) = b, f (b) = c, f (c) = a,$ where $a, b, c,$ are distinct integers.

Video Solution

Answer

Step by step video & image solution for Show that there exists no polynomial f(x) with integral coefficients which satisfy f(a)=b ,f(b)=c ,f(c)=a , where a , b , c , are distinct integers. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS RELATIONS AND FUNCTIONS

Similar Questions

Let a,b be integers and f(x) be a polynomial with integer coefficients such that f(b)-f(a)=1. Then, the value of b-a, is
View Solution
Let f(x) be a polynomial with integral coefficients. If f(1) and f(2) both are odd integers, prove that f(x) = 0 can' t have any integral root.
View Solution
If f(x) is a polynomial of degree at least two with integral co-efficients then the remainder when it is divided by(x-a) (x-b) is , where $a \neq b$
(1) $x [\frac{f (a) - f (b)}{b - a}] + \frac{a f (b) - b f (a)}{a - b}$ (2) $x [\frac{f (a) - f (b)}{a - b}] + \frac{a f (b) - b f (a)}{a - b}$
(3) $x [\frac{f (b) - f (a)}{a - b}] + \frac{a f (b) - b f (a)}{a - b}$ (4) $x [\frac{f (b) - f (a)}{a - b}] + \frac{b f (a) - a f (a)}{a - b}$
View Solution
Let $f (x) = a + b | x | + c {| x |}^{2}$ , where a,b,c are real constants. The, f'(0) exists if
View Solution
Let f (x) be a polynomial function of degree 3 where $a < b < c and f (a) = f (b) = f (c) .$ If the graph of f (x) is as shown, which of the following statements are INCORRECT ? (Where $c > | a |)$

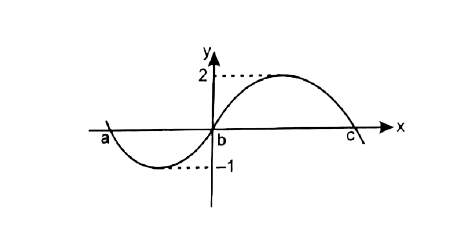
View Solution
If f(x) is a twice differentiable function such that f(a)=0, f(b)=2, f(c)=-1,f(d)=2, f(e)=0 where a < b < c < d e, then the minimum number of zeroes of $g (x) = f' {(x)}^{2} + f'' (x) f (x)$ in the interval [a, e] is
View Solution
If $a and b$ are two distinct real roots of the polynomial $f (x)$ such that $a < b$ , then there exists a real number c lying between $a and b$ , such that
View Solution
For all real numbers x, let the mapping $f (x) = \frac{1}{x - i}$ . where $i = \sqrt{-} 1$ . If there exist real number $a, b, c and d$ for which $f (a), f (b), f (c) and f (d)$ form a square on the complex plane. Find the area of the square.
View Solution
The figure shows the variation of photocurrent with anode potential for a photosensitve surface for three different radiations. Let $l_{a}, l_{b} and l_{c}$ be the curves a, b and c, respectively
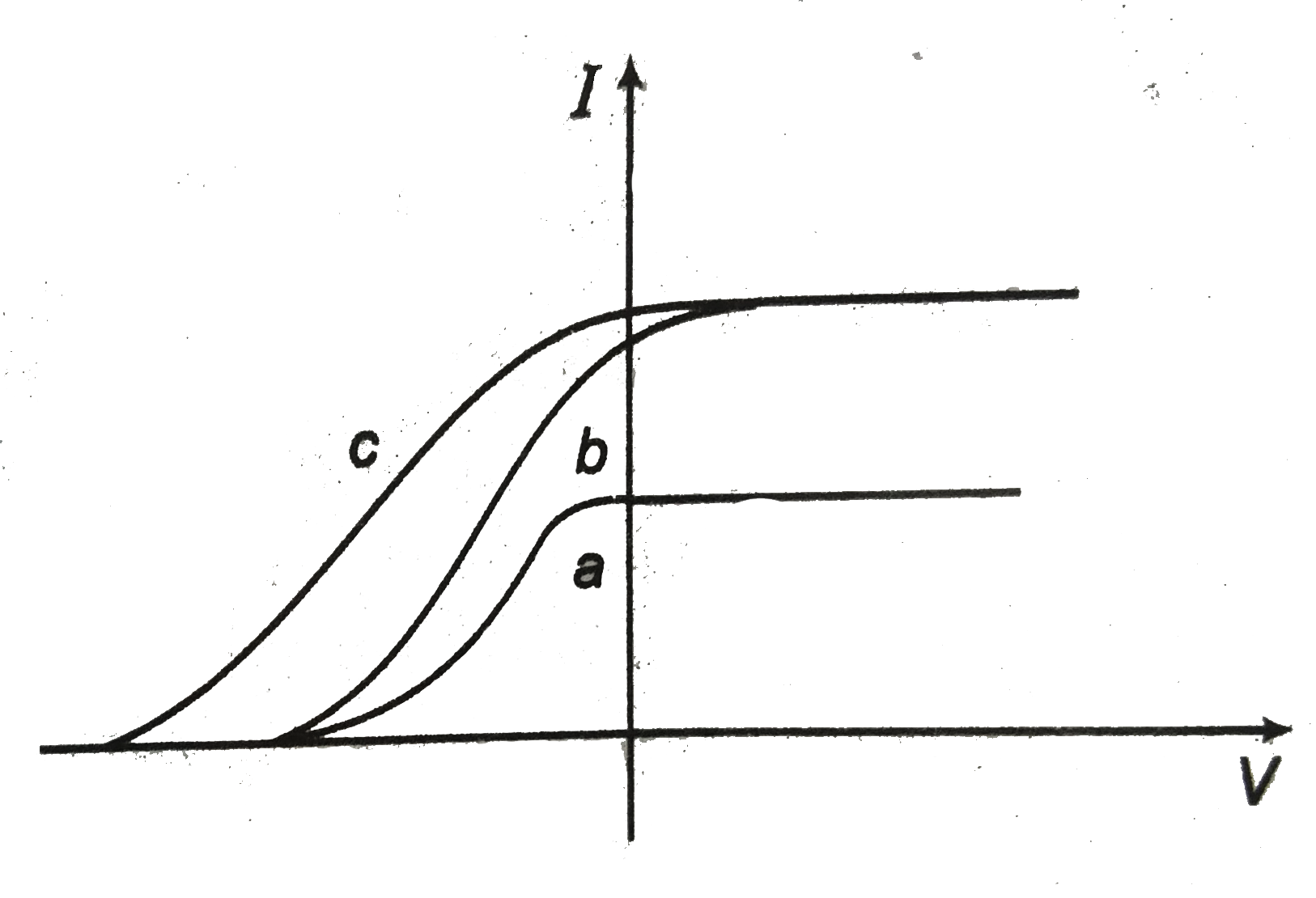
(a) $f_{a} = f_{b} and l_{a} \neq l_{b}$ (b) $f_{a} = f_{c} and l_{a} = l_{c}$
(c ) $f_{a} = f_{b} and l_{a} = l_{b}$ (d) $f_{b} = f_{c} and l_{b} = l_{c}$
View Solution
If f"(x) exists for all points in $[a, b]$ and
$\frac{f (c) - f (a)}{c - a} = \frac{f (b) - f (c)}{b - c}, where a < c < b,$ then show that there exists a number 'k' such that f"(k)=0.
View Solution

Show that there exists no polynomial f(x) with integral coefficients which satisfy f(a)=b,f(b)=c,f(c)=a, where a,b,c, are distinct integers.

Similar Questions

Recommended Questions

Show that there exists no polynomial $f (x)$ with integral coefficients which satisfy $f (a) = b, f (b) = c, f (c) = a,$ where $a, b, c,$ are distinct integers.