Assertion: There are infinite geometric progressions of for which 27,8 and 12 are three of its terms (not necessarily consecutive). Reason: Given terms are integers. (A) Both assertion and reason are correct and Reason is the correct explanation of assertion. (B) Both assertion and reason are correct and Reason is not correct explanation of assertion. (C) Assertion is correct and reason is false. (D) assertion is false and reason is correct.
To solve the given question, we need to analyze the assertion and reason provided.
1. Understanding the Assertion: The assertion states that there are infinite geometric progressions (GPs) for which 27, 8, and 12 are three of its terms (not necessarily consecutive).
2. Setting Up the GP: Let's assume that the first term a of the GP is 8. We denote the common ratio as r. The m-th term of the GP can be expressed as:
am=a⋅rm−1
Therefore, the m-th term is:
am=8⋅rm−1
3. Finding the Terms: We know that:
- The m-th term equals 12:
12=8⋅rm−1
From this, we can solve for rm−1:
rm−1=128=32
- The n-th term equals 27:
27=8⋅rn−1
Similarly, we can solve for rn−1:
rn−1=278=278
4. Equating the Powers of r: Now we have two equations:
rm−1=32
rn−1=278
We can express these in terms of r:
rm−1=(32)andrn−1=(278)
5. Relating m and n: Since both expressions are equal to r raised to some power, we can set up the equation:
rn−1=(rm−1)3
This implies:
n−1=3(m−1)
Rearranging gives:
n=3m−2
This shows that for different values of m, we can find corresponding values of n, indicating that there are indeed infinite GPs.
6. Conclusion on Assertion: The assertion is correct; there are infinite geometric progressions that can contain the terms 27, 8, and 12.
7. Analyzing the Reason: The reason states that the given terms are integers. However, the common ratio r derived from the equations is 32 and 278, which are not integers. Therefore, not all terms of the GP can be integers.
8. Final Conclusion: The assertion is correct, but the reason is false. Thus, the correct option is (C) Assertion is correct and reason is false.
am=a⋅rm−1
Therefore, the m-th term is:
am=8⋅rm−1
- The m-th term equals 12:
12=8⋅rm−1
From this, we can solve for rm−1:
rm−1=128=32
- The n-th term equals 27:
27=8⋅rn−1
Similarly, we can solve for rn−1:
rn−1=278=278
rm−1=32
rn−1=278
We can express these in terms of r:
rm−1=(32)andrn−1=(278)
rn−1=(rm−1)3
This implies:
n−1=3(m−1)
Rearranging gives:
n=3m−2
This shows that for different values of m, we can find corresponding values of n, indicating that there are indeed infinite GPs.
Topper's Solved these Questions
Similar Questions
Assertion (A) Copper sulphate can be stored in zinc vessel.
Reason(R) Zinc is less reactive than copper.
(a) Both assertion and reason are true and the reason is the correct explanation of assertion.
(b) Both assertion and reason are true and reason is not the correct explanation of assertion.
(c) Assertion is true but the reason is false.
(d) Both assertion and reason are false.
View SolutionAssertion(A) Mercury cell does not give steady potential
Reason (R) In the cell reaction, ions are not involved in solution.
(a) Both assertion and reason are true and the reason is the correct explanation of assertion.
(b) Both assertion and reason are true and reason is not the correct explanation of assertion.
(c) Assertion is false but the reason is true.
(d) Both assertion and reason are false.
View Solution
Knowledge Check
Assertion : Cooking food is difficult on hills.
Reason : The boiling point decreases with increase in pressure a) If both assertion and reason are true and reason is the correct explanation of assertion. b) If both assertion and reason are true and reason is not the correct explanation of assertion. c) If assertion is true and reason is false. d) If both assertion and reason are false.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true and reason is not the correct explanation of assertion.CIf assertion is true and reason is false.DIf both assertion and reason are false.Asseration : Deuteromlucetes is known as fungi imperfecti.
Reason : In Deuteromycentes, only the asexual phase is known.1.If both assertion and reason and true and reason is the correct explanation of assertion.2.If both assertion and reason and true and reason is not the correct explanation of assertion. 3.If assertion is true but reason is false. 4.If both assertion and reason are false.AIf both assertion and reason and true and reason is the correct explanation of assertion.BIf both assertion and reason and true and reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.
These questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following five reponses
(a) If both Assertion and Reason arecorrect and Reason is the correct explanation of Asserrtion.
(b) If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion.
(c ) If Assertion is true but Reason is false.
(d) If Assertion is false but Reason is true.
Assertion Spring force is a conservative force.
Reason Potential energy is defined only for conservative forces.
View SolutionThese questions consists of two statements each printed as Assertion and Reason. While answering these question you are required to choose any one of the following five reponses
(a) If both Assertion and Reason arecorrect and Reason is the correct explanation of Asserrtion.
(b) If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion.
(c ) If Assertion is true but Reason is false.
(d) If Assertion is false but Reason is true.
Assertion Total work done by spring may be positive ,negative or zero.
Reason Direction of spring force is always towards mean position.
View SolutionAssertion : Half of the ring is uniformly positively charged and other half uniformly negatively charged. Then, electric field is zero at centre. Then , electric field is zero at centre.
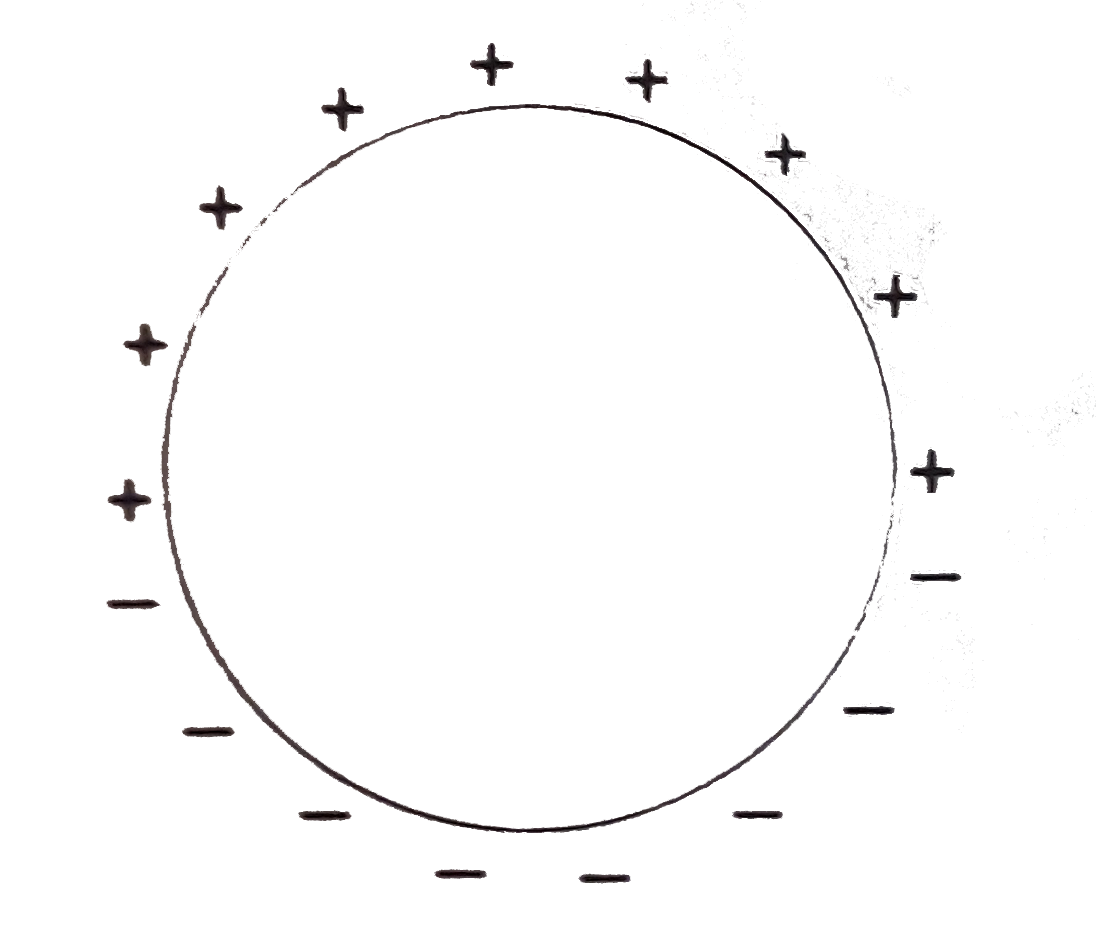
Reason : At the centre of uniformly charged ring, electric field is zero. 1)If both Assertion and Reason are correct and Reason is the correct explanation of Assertion 2)If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion 3)If Assertion is true but Reason is false 4)If Assertion is false but Reason is true
View SolutionAssertion: Glucose reacts with hydroxylamine to form an oxime and alsoadds a molecule of hydrogen cyanide to give cyanohydrin.
Reason: The carbonyl group is present in the open chain structure of glucose.
(a) Both assertion and reason are correct statements, and reason is the correct explanation of the assertion
(b) Both assertion and reason are correct statements, but reason is not the correct explanation of the assertion
(c) Assertion is correct, but reason is wrong statement.
(d) Assertion is wrong, but reason is correct statement.
View SolutionAssertion(A) For measuring resistance of an ionic solution an AC source is used.
Reason (R) Concentration of ionic solution will change if DC source is used.
(a) Both assertion and reason are true and the reason is the correct explanation of assertion.
(b) Both assertion and reason are true and reason is not the correct explanation of assertion.
(c) Assertion is true but the reason is false.
(d) Both assertion and reason are false.
View SolutionAssertion : Threatened species are those living species which have been greatly reduced in their number and are liable to become extinct if the causative factors continue.
Reason : IUCN is an international organisation which maintains the IUCN red list of threatened species, to assess the conservation status of different species.
(a) If both assertion and reason are true and reason is the correct explanation of assertion.
(b) If both assertion and reason are true but reason is not the correct explanation of assertion.
(c) If assertion is true but reason is false.
(d) If both assertion and reason are false.
View Solution