Find the ratio of .20Crand.25Cr when each of them has the greatest possible value.
To find the ratio of (20r) and (25r) when each of them has the greatest possible value, we can follow these steps:
Step 1: Determine the value of r for (20r)
The binomial coefficient (nr) is maximized when r is around n2. For n=20:
r=202=10
Thus, (20r) is maximized at r=10.
Step 2: Determine the value of r for (25r)
Similarly, for n=25:
r=25+12=13(for the upper half)
r=25−12=12(for the lower half)
Thus, (25r) is maximized at both r=12 and r=13.
Step 3: Calculate the ratio (2010)(2512) or (2010)(2513)
We can calculate the ratio using either (2512) or (2513) since they will yield the same maximum value. Let's use (2512):
Ratio=(2010)(2512)=20!10!⋅10!25!12!⋅13!
Step 4: Simplify the ratio
This can be rewritten as:
=20!⋅12!⋅13!10!⋅10!⋅25!
Step 5: Expand the factorials
Now, we can expand 25! as follows:
25!=25⋅24⋅23⋅22⋅21⋅20!
Substituting this back into the ratio gives:
=20!⋅12!⋅13!10!⋅10!⋅(25⋅24⋅23⋅22⋅21⋅20!)
Step 6: Cancel out 20!
Cancelling 20! from the numerator and denominator:
=12!⋅13!10!⋅10!⋅(25⋅24⋅23⋅22⋅21)
Step 7: Further simplify 12! and 13!
We can express 13! as 13⋅12!:
=12!⋅13⋅12!10!⋅10!⋅(25⋅24⋅23⋅22⋅21)
Step 8: Final simplification
Now we can cancel 12!:
=13(25⋅24⋅23⋅22⋅21)⋅10!10!
Conclusion
Thus, the final ratio is:
13⋅1125⋅24⋅23⋅22⋅21
r=202=10
Thus, (20r) is maximized at r=10.
r=25+12=13(for the upper half)
r=25−12=12(for the lower half)
Thus, (25r) is maximized at both r=12 and r=13.
=20!⋅12!⋅13!10!⋅10!⋅25!
25!=25⋅24⋅23⋅22⋅21⋅20!
=20!⋅12!⋅13!10!⋅10!⋅(25⋅24⋅23⋅22⋅21⋅20!)
=12!⋅13!10!⋅10!⋅(25⋅24⋅23⋅22⋅21)
=12!⋅13⋅12!10!⋅10!⋅(25⋅24⋅23⋅22⋅21)
=13(25⋅24⋅23⋅22⋅21)⋅10!10!
13⋅1125⋅24⋅23⋅22⋅21
Topper's Solved these Questions
Similar Questions
Find the ratio of ^20Crand25Cr when each of them has the greatest possible value.
View SolutionFind the value of :50C4+6∑r=1.56−rC3.
View SolutionIf a,b,c∈R−{0} and a2=bc and a+b+c=abc then the least possible value of a2 is
View SolutionIf .nPr=720and.nCr=120 then find the value of r.
View SolutionThe maximum value of .nCr is obtained when r is equal to
View SolutionIn the circuit shown in figure E1,E2=20V,R1=R2=10kΩ and C=1μF. Find the current through R1,R2 and C when
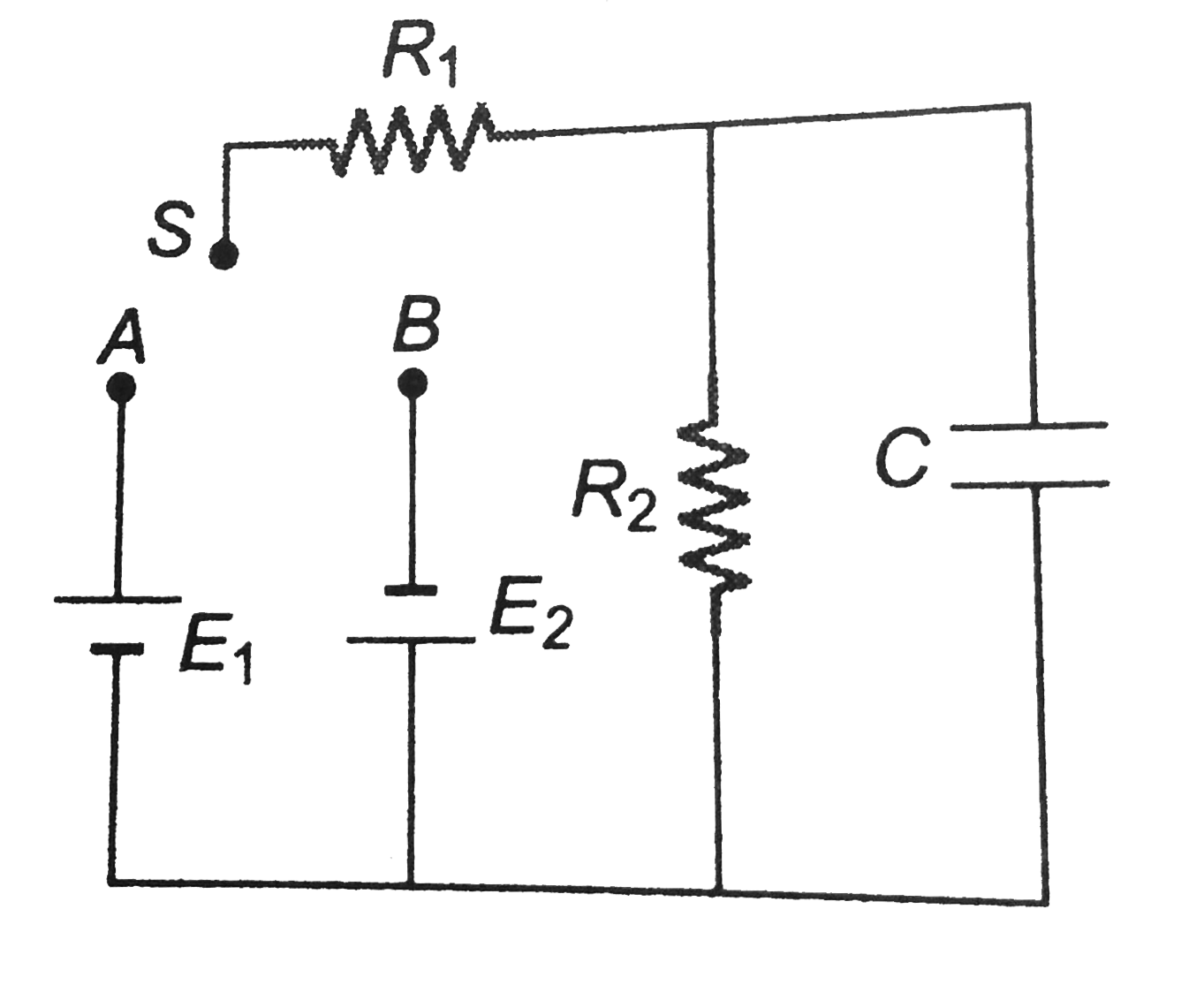
(a) S has been kept connected to A for a long time.
(b) The switch is suddenly shifted to B.
View SolutionFind the rate of change of the area of a circle with respect to its radius r when(a) r=3cm (b) r=4cm
View SolutionIn two similar triangles ABC and PQR , if their corresponding altitudes AD and PS are in the ratio 4:9, find the ratio of the areas of triangle ABC and triangle PQR .
View SolutionIf .15Cr:.15CR−1=11:5, then find the value of 'r'.
View SolutionA body has maximum range R1 when projected up the plane. The same body when projected down the inclined plane, it has maximum range R2. Find the maximum horizontal range. Assume equal speed of projection in each case and the body is projected onto the inclined plane in the line of the greatest slope.
View Solution
