A continuous and differentiable function y=f(x) is such that its graph cuts line y=mx+c at n distinct points. Then the minimum number of points at which f''(x)=0 is/are (a)n−1 (b) n−3 (c)n−2 (d) cannot say
To solve the problem, we need to analyze the relationship between the function y=f(x) and the line y=mx+c where they intersect at n distinct points.
1. Understanding the Intersection:
The function f(x) intersects the line y=mx+c at n distinct points. This means that the equation f(x)=mx+c has n distinct roots.
2. Formulating the Equation:
Since f(x)=mx+c has n roots, we can express this as:
f(x)−(mx+c)=0
This is a polynomial equation of degree n (assuming f(x) is a polynomial).
3. First Derivative:
By applying the first derivative, we differentiate both sides:
f′(x)−m=0
This implies:
f′(x)=m
Since f(x)−(mx+c)=0 has n roots, the first derivative f′(x) will have n−1 roots (by the Fundamental Theorem of Algebra).
4. Second Derivative:
Now, we differentiate again to find the second derivative:
f′′(x)=0
The second derivative f′′(x) will have n−2 roots because the first derivative f′(x) has n−1 roots.
5. Conclusion:
Therefore, the minimum number of points at which f′′(x)=0 is n−2.
Final Answer:
The minimum number of points at which f′′(x)=0 is n−2.
The function f(x) intersects the line y=mx+c at n distinct points. This means that the equation f(x)=mx+c has n distinct roots.
Since f(x)=mx+c has n roots, we can express this as:
f(x)−(mx+c)=0
This is a polynomial equation of degree n (assuming f(x) is a polynomial).
By applying the first derivative, we differentiate both sides:
f′(x)−m=0
This implies:
f′(x)=m
Since f(x)−(mx+c)=0 has n roots, the first derivative f′(x) will have n−1 roots (by the Fundamental Theorem of Algebra).
Now, we differentiate again to find the second derivative:
f′′(x)=0
The second derivative f′′(x) will have n−2 roots because the first derivative f′(x) has n−1 roots.
Therefore, the minimum number of points at which f′′(x)=0 is n−2.
The minimum number of points at which f′′(x)=0 is n−2.
Similar Questions
A continuous and differentiable function y=f(x) is such that its graph cuts line y=mx+c at n distinct points. Then the minimum number of points at which f''(x)=0 is/are
View SolutionIf f(x) is continuous and differerntiable function such that f(1n)=0 for all n∈N, then
View SolutionIf function y=f(x) has only two points of discontinuity say x1,x2, where x1,x2<0, then the number of points of discontinuity of y=f(−|x+1|) is (a) 0 (b) 2 (c) 6 (d) 4
View SolutionFind the conditions that the straight lines y=m1x+c1,y=m2x+c2and y=m3x+c3 may meet in a point.
View SolutionIf f(x) is a real-valued function discontinuous at all integral points lying in [0,n] and if (f(x))2=1,∀x∈[0,n], then number of functions f(x) are
View SolutionQuestion 1 and 2 refer to the information and graph below.
Let function f be defined by the graph in the accompanying figure.
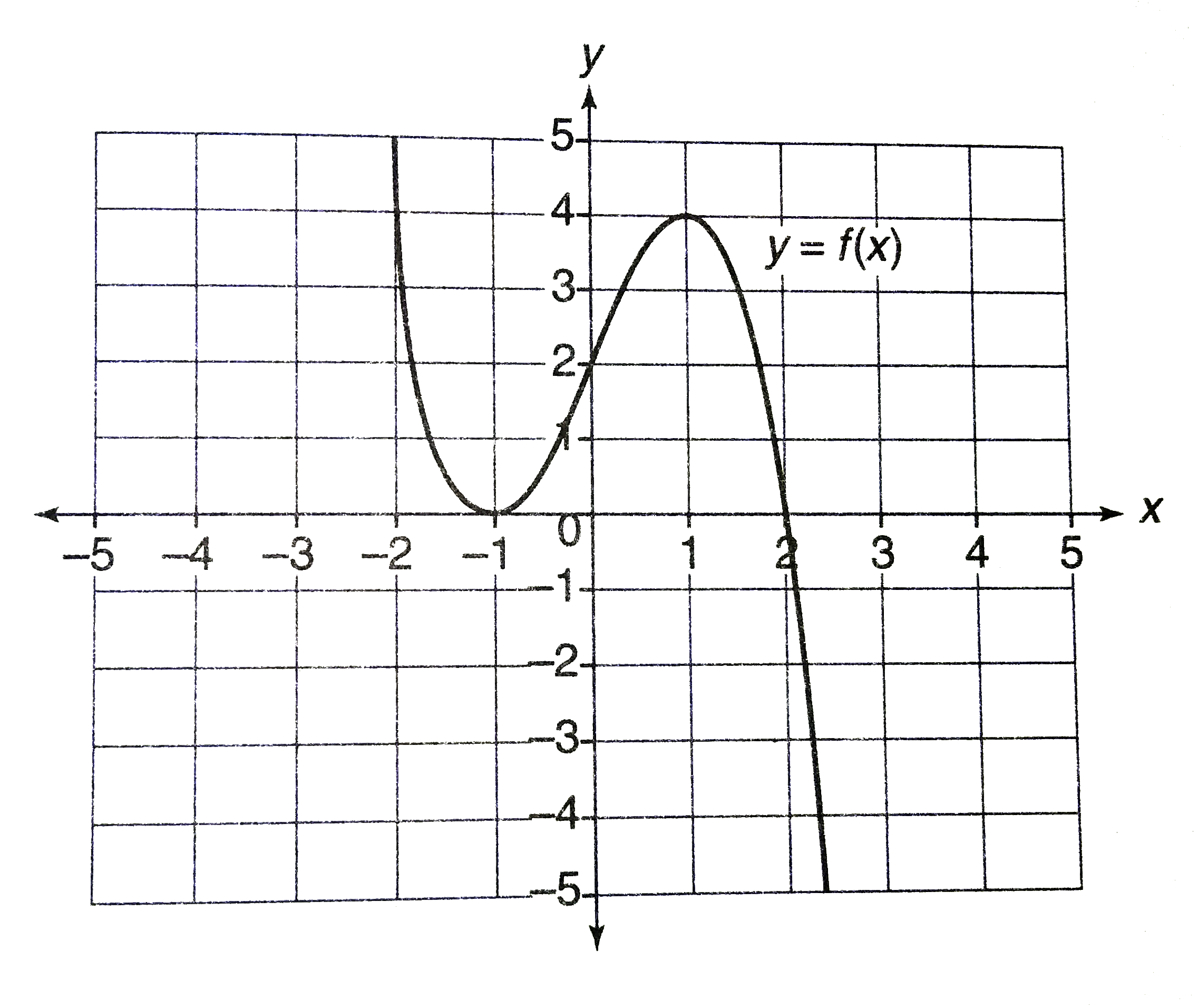
Q. Let m represents the number of points at which the graphs of y=f(x)andg(x)=3 intersect. Let n represents the number of points at which the graphs of y=f(x)−1andg(x)=3 intersect. What is the value of m+n?
View SolutionProve that (xa)n+(yb)n=2 touches the straight line xa+yb=2 for all n∈N , at the point (a, b) .
View SolutionThe graph y=2x3−4x+2andy=x3+2x−1 intersect in exactly 3 distinct points. Then find the slope of the line passing through two of these points.
View SolutionThe graph y=2x3−4x+2andy=x3+2x−1 intersect in exactly 3 distinct points. Then find the slope of the line passing through two of these points.
View SolutionA function y=f(x) has a second-order derivative f''(x)=6(x−1). It its graph passes through the point (2,1) and at that point tangent to the graph is y=3x−5, then the value of f(0) is 1 (b) −1 (c) 2 (d) 0
View Solution
