A continuous and differentiable function y=f(x) is such that its graph cuts line y=mx+c at n distinct points. Then the minimum number of points at which f''(x)=0 is/are
n−1
n−3
n−2
cannot say
The correct Answer is:C
To solve the problem step by step, we need to analyze the given information about the function y=f(x) and the line y=mx+c.
Step 1: Understand the problem
We have a continuous and differentiable function y=f(x) that intersects the line y=mx+c at n distinct points. We need to determine the minimum number of points where the second derivative f′′(x)=0.
Step 2: Identify the implications of the intersections
Since the function f(x) intersects the line y=mx+c at n distinct points, we can denote these points as x1,x2,…,xn. At each of these points, we have:
f(xi)=mxi+cfor i=1,2,…,n
Step 3: Apply the Mean Value Theorem
According to the Mean Value Theorem (MVT), if f(x) is continuous on the closed interval (xi,xi+1) and differentiable on the open interval (xi,xi+1), there exists at least one point ci in (xi,xi+1) such that:
f′(ci)=f(xi+1)−f(xi)xi+1−xi
Since f(xi+1)=mxi+1+c and f(xi)=mxi+c, we can simplify this to:
f′(ci)=m
for each interval (xi,xi+1).
Step 4: Count the intervals
With n distinct points, we have n−1 intervals:
1. (x1,x2)
2. (x2,x3)
3. ...
4. (xn−1,xn)
Step 5: Apply Rolle's Theorem
Since f′(ci)=m for each of the n−1 intervals, we can apply Rolle's Theorem to each interval. This theorem states that if a function is continuous on a closed interval and differentiable on the open interval, then there exists at least one point di in (xi,xi+1) such that:
f′′(di)=0
Thus, for n−1 intervals, we will have at least n−2 points where f′′(x)=0.
Conclusion
Therefore, the minimum number of points at which f′′(x)=0 is:
n−2
We have a continuous and differentiable function y=f(x) that intersects the line y=mx+c at n distinct points. We need to determine the minimum number of points where the second derivative f′′(x)=0.
Since the function f(x) intersects the line y=mx+c at n distinct points, we can denote these points as x1,x2,…,xn. At each of these points, we have:
f(xi)=mxi+cfor i=1,2,…,n
According to the Mean Value Theorem (MVT), if f(x) is continuous on the closed interval (xi,xi+1) and differentiable on the open interval (xi,xi+1), there exists at least one point ci in (xi,xi+1) such that:
f′(ci)=f(xi+1)−f(xi)xi+1−xi
Since f(xi+1)=mxi+1+c and f(xi)=mxi+c, we can simplify this to:
f′(ci)=m
for each interval (xi,xi+1).
With n distinct points, we have n−1 intervals:
1. (x1,x2)
2. (x2,x3)
3. ...
4. (xn−1,xn)
Since f′(ci)=m for each of the n−1 intervals, we can apply Rolle's Theorem to each interval. This theorem states that if a function is continuous on a closed interval and differentiable on the open interval, then there exists at least one point di in (xi,xi+1) such that:
f′′(di)=0
Thus, for n−1 intervals, we will have at least n−2 points where f′′(x)=0.
Therefore, the minimum number of points at which f′′(x)=0 is:
n−2
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - MULTIPLE CORRECT ANSWER TYPE
16 Videos
- APPLICATION OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - LINKED COMPREHENSION TYPE
8 Videos
- APPLICATION OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - CONCEPT APPLICATION EXERCISE 5.8
9 Videos
- 3D COORDINATION SYSTEM BOOK - CENGAGE ENGLISHCHAPTER - 3D COORDINATION SYSTEM EXERCISE - DPP 3.1
11 Videos
- APPLICATION OF INTEGRALSBOOK - CENGAGE ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - All Questions
142 Videos
Similar Questions
The absolute value function y=f(x)=|x| Draw its graph.
View Solutionif f(x) is differentiable function such that f(1) = sin 1, f (2)= sin 4 and f(3) = sin 9, then the minimum number of distinct roots of f'(x) = 2x cosx2 in (1,3) is _______
View SolutionIf the graph of the function y = f(x) is symmetrical about the line x = 2, then
View SolutionIf the graph of the function y=f(x) is as shown :

the graph of y=12(|f(x)|−f(x)) is
View SolutionQuestion 1 and 2 refer to the information and graph below.
Let function f be defined by the graph in the accompanying figure.
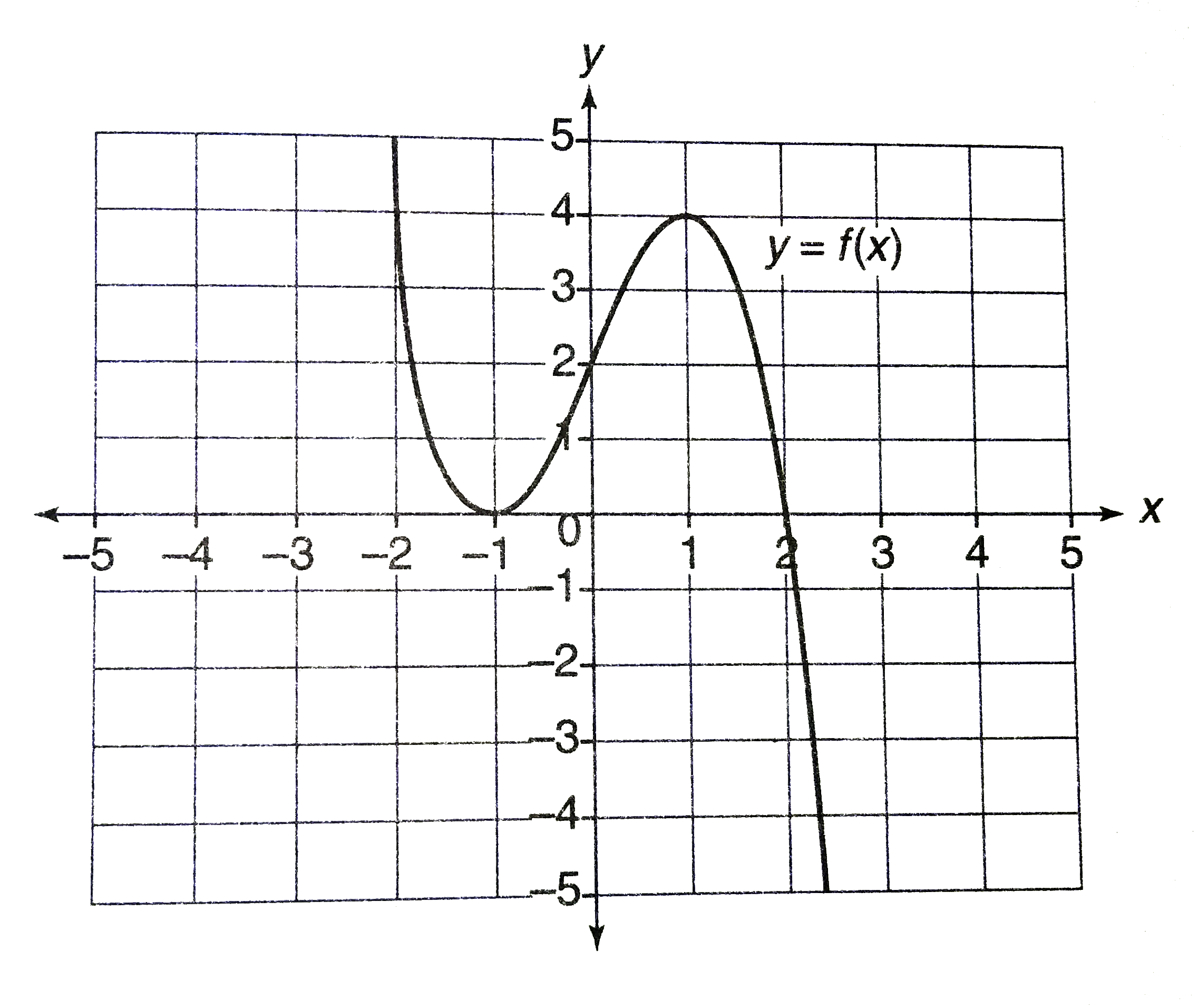
Q. Let m represents the number of points at which the graphs of y=f(x)andg(x)=3 intersect. Let n represents the number of points at which the graphs of y=f(x)−1andg(x)=3 intersect. What is the value of m+n?
View SolutionLet a differentiable function f:R→R be such that for all x and y in R
2|f(x)−f(y)|≤|x−y| and f′(x)≥12. So then the number of points of intersection of the graph y=f(x) with
View SolutionLet f(x)=(lim)n→∞n−1∑r=0x(rx+1){(r+1)x+1} . Then (A) f(x) is continuous but not differentiable at x=0 (B) f(x) is both continuous but not differentiable at x=0 (C) f(x) is neither continuous not differentiable at x=0 (D) f(x) is a periodic function.
View SolutionA differentiable function y=f(x) satisfies f'(x)=(f(x))2+5 and f(0)=1. Then the equation of tangent at the point where the curve crosses y-axis, is
View SolutionLet f be a continuous, differentiable, and bijective function. If the tangent to y=f(x)atx=a is also the normal to y=f(x)atx=b, then there exists at least one c∈(a,b) such that f′(c)=0 (b) f′(c)>0 f′(c)<0 (d) none of these
View SolutionIf the line y=mx−(m−1) cuts the circle x2+y2=4 at two real and distinct points then total values of m are
View Solution
