Column I, Column II stick of length 10 units rests against the floor and a wall of a room. If the stick begins to slide on the floor then the locus of its middle point is (Assume the origin to be the intersection of the floor and the wall and the coordinate axes along the floor and the wall), p. √6 ∀′ is the major axis of the ellipse 3x2+2y2+6x−4y−1=0 and P is a variable point on it. Then the greatest area of triangle APA' is, q. 2√7 The distance between the foci of the curve represented by the equation x=1+4cosθ,y=2+3sinθ is, r. 1283 Tangents are drawn to the ellipse x216+y27=1 at the endpoints of the latus rectum. The area of the quadrilateral so formed is, s. √5
To solve the given questions step by step, we will break down each problem and provide a detailed solution.
Question 1: Locus of the Middle Point of a Sliding Stick
1. Understanding the Problem: A stick of length 10 units rests against the floor (x-axis) and a wall (y-axis). We need to find the locus of the midpoint of the stick as it slides.
2. Setting Up Coordinates: Let the endpoints of the stick be A(a, 0) on the x-axis and B(0, b) on the y-axis. The length of the stick gives us the equation:
√a2+b2=10
Squaring both sides, we have:
a2+b2=100
3. Midpoint Coordinates: The midpoint M of the stick is given by:
M(a2,b2)
Let x1=a2 and y1=b2. Therefore, a=2x1 and b=2y1.
4. Substituting into the Length Equation: Substitute a and b into the equation a2+b2=100:
(2x1)2+(2y1)2=100
Simplifying gives:
4x21+4y21=100
Dividing by 4:
x21+y21=25
5. Conclusion: The locus of the midpoint M is a circle with radius 5 centered at the origin.
Question 2: Area of Triangle APA′
1. Ellipse Equation: The given ellipse is:
3x2+2y2+6x−4y−1=0
Rearranging gives:
3(x2+2x)+2(y2−2y)=1
Completing the square:
3((x+1)2−1)+2((y−1)2−1)=1
Simplifying:
3(x+1)2+2(y−1)2=6
Dividing by 6 gives:
(x+1)22+(y−1)23=1
2. Identifying Major and Minor Axes: Here, a2=2 and b2=3, so a=√2 and b=√3.
3. Finding Maximum Area: The area A of triangle APA′ is given by:
A=12×base×height=12×2a×b=ab
Substituting values:
A=√2×√3=√6
Question 3: Distance Between Foci
1. Parametric Equations: The given equations are:
x=1+4cosθ,y=2+3sinθ
2. Converting to Standard Form: Rearranging gives:
x−14=cosθ,y−23=sinθ
Using the identity sin2θ+cos2θ=1:
(x−14)2+(y−23)2=1
3. Identifying Parameters: This is an ellipse with a2=16 (so a=4) and b2=9 (so b=3).
4. Finding Distance Between Foci: The distance between the foci is given by:
2cwhere c=√a2−b2=√16−9=√7
Thus, the distance is:
2√7
Question 4: Area of Quadrilateral Formed by Tangents
1. Ellipse Equation: The ellipse is given by:
x216+y27=1
The endpoints of the latus rectum are at:
(0,±b2/a)=(0,±7/4)
2. Finding Tangents: The tangents at these points create a quadrilateral with vertices at the endpoints of the latus rectum.
3. Calculating Area: The area of the quadrilateral formed by the tangents can be calculated using the formula:
Area=2ab=2×4×74=7
Final Answers:
1. Locus of midpoint: p=5
2. Maximum area of triangle APA′: q=2√7
3. Distance between foci: r=1283
4. Area of quadrilateral: s=√5
√a2+b2=10
Squaring both sides, we have:
a2+b2=100
M(a2,b2)
Let x1=a2 and y1=b2. Therefore, a=2x1 and b=2y1.
(2x1)2+(2y1)2=100
Simplifying gives:
4x21+4y21=100
Dividing by 4:
x21+y21=25
3x2+2y2+6x−4y−1=0
Rearranging gives:
3(x2+2x)+2(y2−2y)=1
Completing the square:
3((x+1)2−1)+2((y−1)2−1)=1
Simplifying:
3(x+1)2+2(y−1)2=6
Dividing by 6 gives:
(x+1)22+(y−1)23=1
A=12×base×height=12×2a×b=ab
Substituting values:
A=√2×√3=√6
x=1+4cosθ,y=2+3sinθ
x−14=cosθ,y−23=sinθ
Using the identity sin2θ+cos2θ=1:
(x−14)2+(y−23)2=1
2cwhere c=√a2−b2=√16−9=√7
Thus, the distance is:
2√7
x216+y27=1
The endpoints of the latus rectum are at:
(0,±b2/a)=(0,±7/4)
Area=2ab=2×4×74=7
1. Locus of midpoint: p=5
2. Maximum area of triangle APA′: q=2√7
3. Distance between foci: r=1283
4. Area of quadrilateral: s=√5
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions886videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
Similar Questions
A stick of length 10 units rests against the floor and a wall of a room. If the stick begins to slide ontfloor then the locus of its middle point is:
View SolutionA ladder 5m long rests against a vertical wall. If its top slides down at the rate of 10cm/sec, then, when the foot of the ladder is 4m away from the wall, the angle between the floor and the ladder is decreasing at the rate of
View Solution
Knowledge Check
- Question 1 - Select One
A 3m along ladder weighing 20kg leans on a frictionless wall. Its feet rest on the floor 1m from the wall. Find the rection forces of the wall and the floor.
A25√2 N, 203 NB50√2 N, 230 NC203 N, 25√2 ND230N,50√2 N
A rod of AB of length 3 rests on a wall as follows :
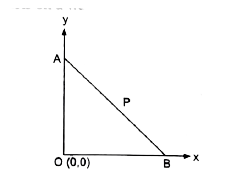
P is a point on AB such that AP:PB=1:2 If the rod slides along the wall, then the locus of P lies onView SolutionThe length of a hall is 18m and the width 12m. The sum of the areas of the floor and the flat roof is equal to the sum of the areas of the four walls. Find the height of the hall.
View SolutionThe length of a hall is 18m and the width 12m. The sum of the areas of the floor and the flat roof is equal to the sum of the areas of the four walls. Find the height of the hall.
View SolutionA 3m along ladder weighing 20kg leans on a frictionless wall. Its feet rest on the floor 1m from the wall. Find the rection forces of the wall and the floor.
View SolutionShow that the product of the areas of the floor and two adjacent walls of a cuboid is the square of its volume.
View SolutionA thin rod of length L and mass M is held vertically with one end on the floor and is allowed to fall. Find the velocity of the other end when it hits the floor, assuming that the end on the floor does not slip?
View SolutionA room is in the shape of a square of side 4m. Calculate the area of the floor. What is the cost of tiling the floor at the rate of ₹ 24 per square metre?
View Solution

