The mean deviation about the mean of the following distribution is
1
1.25
1.5
1.75
The correct Answer is:B
To find the mean deviation about the mean for the given distribution, we will follow these steps:
Step 1: Organize the Data
We have the following data:
| Size (Xi) | Frequency (Fi) |
|-----------|----------------|
| 20 | 6 |
| 21 | 4 |
| 22 | 5 |
| 23 | 1 |
| 24 | 4 |
Step 2: Calculate Fi * Xi
Next, we calculate the product of frequency and size for each class:
- For Xi = 20: Fi * Xi = 6 * 20 = 120
- For Xi = 21: Fi * Xi = 4 * 21 = 84
- For Xi = 22: Fi * Xi = 5 * 22 = 110
- For Xi = 23: Fi * Xi = 1 * 23 = 23
- For Xi = 24: Fi * Xi = 4 * 24 = 96
Now, we can summarize this in a table:
| Size (Xi) | Frequency (Fi) | Fi * Xi |
|-----------|----------------|---------|
| 20 | 6 | 120 |
| 21 | 4 | 84 |
| 22 | 5 | 110 |
| 23 | 1 | 23 |
| 24 | 4 | 96 |
| Total | 20 | 483 |
Step 3: Calculate the Mean (X̄)
To find the mean (X̄), we use the formula:
Xˉ=∑(Fi∗Xi)∑Fi
Substituting the values:
Xˉ=48320=24.15
Step 4: Calculate Deviations (Di)
Now we calculate the deviations from the mean (X̄):
- For Xi = 20: Di = 20 - 21.65 = -1.65
- For Xi = 21: Di = 21 - 21.65 = -0.65
- For Xi = 22: Di = 22 - 21.65 = 0.35
- For Xi = 23: Di = 23 - 21.65 = 1.35
- For Xi = 24: Di = 24 - 21.65 = 2.35
Step 5: Calculate Fi * |Di|
Next, we calculate the absolute deviations multiplied by frequency:
| Size (Xi) | Frequency (Fi) | |Di| | Fi * |Di| |
|-----------|----------------|-------|---------|
| 20 | 6 | 1.65 | 9.90 |
| 21 | 4 | 0.65 | 2.60 |
| 22 | 5 | 0.35 | 1.75 |
| 23 | 1 | 1.35 | 1.35 |
| 24 | 4 | 2.35 | 9.40 |
| Total | 20 | | 25.00 |
Step 6: Calculate Mean Deviation
Finally, we calculate the mean deviation about the mean using the formula:
Mean Deviation=∑(Fi∗|Di|)∑Fi
Substituting the values:
Mean Deviation=25.0020=1.25
Conclusion
The mean deviation about the mean of the given distribution is 1.25.
---
We have the following data:
|-----------|----------------|
| 20 | 6 |
| 21 | 4 |
| 22 | 5 |
| 23 | 1 |
| 24 | 4 |
Next, we calculate the product of frequency and size for each class:
- For Xi = 21: Fi * Xi = 4 * 21 = 84
- For Xi = 22: Fi * Xi = 5 * 22 = 110
- For Xi = 23: Fi * Xi = 1 * 23 = 23
- For Xi = 24: Fi * Xi = 4 * 24 = 96
|-----------|----------------|---------|
| 20 | 6 | 120 |
| 21 | 4 | 84 |
| 22 | 5 | 110 |
| 23 | 1 | 23 |
| 24 | 4 | 96 |
| Total | 20 | 483 |
To find the mean (X̄), we use the formula:
Now we calculate the deviations from the mean (X̄):
- For Xi = 21: Di = 21 - 21.65 = -0.65
- For Xi = 22: Di = 22 - 21.65 = 0.35
- For Xi = 23: Di = 23 - 21.65 = 1.35
- For Xi = 24: Di = 24 - 21.65 = 2.35
Next, we calculate the absolute deviations multiplied by frequency:
|-----------|----------------|-------|---------|
| 20 | 6 | 1.65 | 9.90 |
| 21 | 4 | 0.65 | 2.60 |
| 22 | 5 | 0.35 | 1.75 |
| 23 | 1 | 1.35 | 1.35 |
| 24 | 4 | 2.35 | 9.40 |
| Total | 20 | | 25.00 |
Finally, we calculate the mean deviation about the mean using the formula:
The mean deviation about the mean of the given distribution is 1.25.
Topper's Solved these Questions
- STATISTICSBOOK - CENGAGE ENGLISHCHAPTER - STATISTICSEXERCISE - Exercise 11.1
5 Videos
- STATISTICSBOOK - CENGAGE ENGLISHCHAPTER - STATISTICSEXERCISE - Exercise 11.2
6 Videos
- SOLUTIONS AND PROPERTIES OF TRIANGLE BOOK - CENGAGE ENGLISHCHAPTER - SOLUTIONS AND PROPERTIES OF TRIANGLE EXERCISE - Comprehension Type
6 Videos
- STRAIGHT LINEBOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
Similar Questions
Find the mean deviation about the mean for the following data.
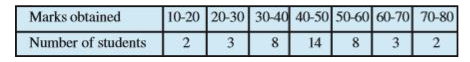
View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about mean for the following data:
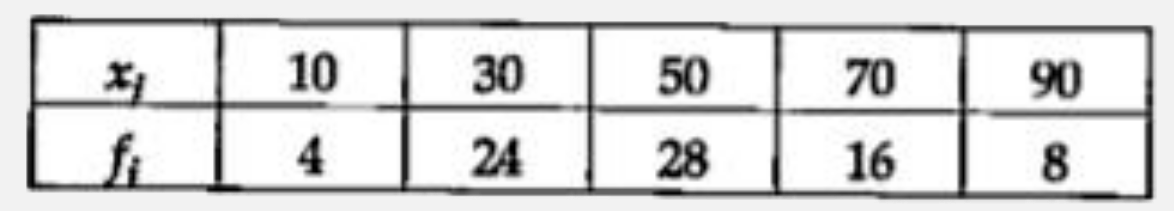
View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following continuous distribution.

View SolutionFind the mean for the following frequency distribution :
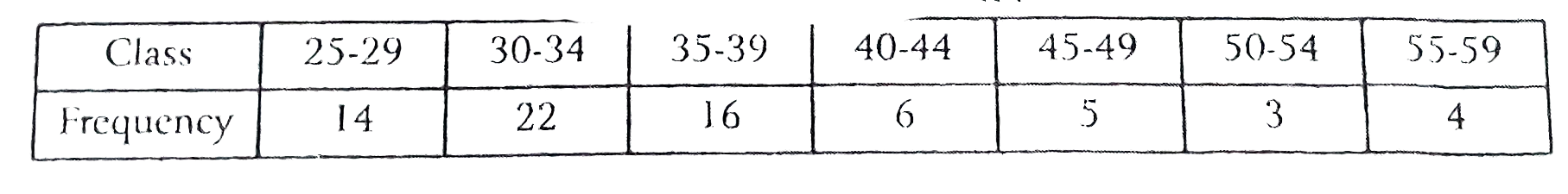
View SolutionFind the mean deviation about the median for the following frequency distribution.

View SolutionFind the mean deviation about the median for the following continuous distribution.
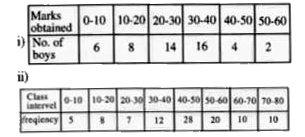
View SolutionThe mean deviation about the median of the following distribution is
Marks obtained1011121415Number of students23834
View Solution
