Find The standard deviation of the following frequency distribution is
X234567f491614116
1.38
1.42
1.45
1.60
The correct Answer is:A
To find the standard deviation of the given frequency distribution, we will follow these steps:
Step 1: Create a frequency table
We will create a table with the values of X, their corresponding frequencies f, the deviation d (where d=X−A and A is the assumed mean), f⋅d, and f⋅d2.
Given:
- X={2,3,4,5,6,7}
- f={4,9,16,14,11,6}
Assume A=4 (the mean value).
| X | f | d=X−A | f⋅d | f⋅d2 |
|-------|-------|----------------|----------------|------------------|
| 2 | 4 | -2 | -8 | 16 |
| 3 | 9 | -1 | -9 | 9 |
| 4 | 16 | 0 | 0 | 0 |
| 5 | 14 | 1 | 14 | 14 |
| 6 | 11 | 2 | 22 | 44 |
| 7 | 6 | 3 | 18 | 54 |
Step 2: Calculate the sums
Now we will calculate the sums of f, f⋅d, and f⋅d2.
- N=∑f=4+9+16+14+11+6=60
- ∑(f⋅d)=−8−9+0+14+22+18=37
- ∑(f⋅d2)=16+9+0+14+44+54=137
Step 3: Apply the standard deviation formula
The formula for standard deviation σ for a frequency distribution is given by:
σ=
⎷∑(f⋅d2)N−(∑(f⋅d)N)2
Substituting the values we calculated:
σ=√13760−(3760)2
Step 4: Calculate each component
1. Calculate 13760:
13760≈2.2833
2. Calculate 3760:
3760≈0.6167
3. Now, square 3760:
(3760)2≈0.3794
Step 5: Final calculation
Now substitute back into the formula:
σ=√2.2833−0.3794≈√1.9039≈1.38
Conclusion
The standard deviation of the given frequency distribution is approximately 1.38.
---
We will create a table with the values of X, their corresponding frequencies f, the deviation d (where d=X−A and A is the assumed mean), f⋅d, and f⋅d2.
- X={2,3,4,5,6,7}
- f={4,9,16,14,11,6}
|-------|-------|----------------|----------------|------------------|
| 2 | 4 | -2 | -8 | 16 |
| 3 | 9 | -1 | -9 | 9 |
| 4 | 16 | 0 | 0 | 0 |
| 5 | 14 | 1 | 14 | 14 |
| 6 | 11 | 2 | 22 | 44 |
| 7 | 6 | 3 | 18 | 54 |
Now we will calculate the sums of f, f⋅d, and f⋅d2.
- ∑(f⋅d)=−8−9+0+14+22+18=37
- ∑(f⋅d2)=16+9+0+14+44+54=137
The formula for standard deviation σ for a frequency distribution is given by:
⎷∑(f⋅d2)N−(∑(f⋅d)N)2
1. Calculate 13760:
13760≈2.2833
3760≈0.6167
(3760)2≈0.3794
Now substitute back into the formula:
The standard deviation of the given frequency distribution is approximately 1.38.
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - STATISTICSEXERCISE - Exercise 11.15videos
STATISTICS
- BOOK - CENGAGE ENGLISHCHAPTER - STATISTICSEXERCISE - Exercise 11.26videos
STATISTICS
- BOOK - CENGAGE ENGLISHCHAPTER - SOLUTIONS AND PROPERTIES OF TRIANGLE EXERCISE - Comprehension Type6videos
SOLUTIONS AND PROPERTIES OF TRIANGLE
- BOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
Similar Questions
Find the mean and standard deviation of the following frequency distribution :
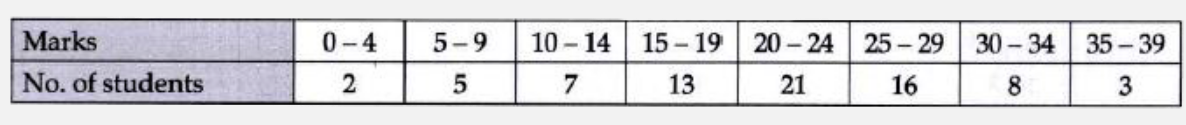 View Solution
View SolutionIn the following frequency distribution :
x01356810f2672119153
The mode isView SolutionFind the variance and standard deviation of the following frequency distribution: Variable (xi) , 2, 4, 6, 8, 10, 12, 14, 16 Frequency (fi) , 4, 4, 5, 15, 8, 5, 4, 5
View SolutionThe median for the following frequency distribution is :
xi :123456789fi :810111620251596View SolutionFind the median from the following frequency distribution :
x34567891011f1390811176627622View SolutionFind the standard deviation for the following data :5,9,8,12,6,10,6,8
View SolutionFind the mean deviation about the median of the following frequency distribution: Class:, 0-6, 6-12, 12-18, 18-24, 24-30 Frequency:, 8, 10, 12, 9, 5
View SolutionFind the mean deviation about the median of the following frequency distribution: Class:, 0-6, 6-12, 12-18, 18-24, 24-30 Frequency:, 8, 10, 12, 9, 5
View SolutionFind the mean of the following frequency distribution: Variable (xi) , 2, 4, 6, 8, 10, 12, 14, 16 Frequency (fi) , 4, 4, 5, 15, 8, 5, 4, 5
View SolutionCalculate mean, variance and standard deviation of the following frequency distribution: Class 1-10 10-20 20-30 30-40 40-50 0-60 Frequency: 11 29 18 4 5 3
View Solution

