A line $L_{1} \equiv 3 y - 2 x - 6 = 0$ is rotated about its point of intersection with the y-axis in the clockwise direction to make it $L_{2}$ such that the are formed by $L_{1}, L_{2}$ the x-axis, and line $x = 5$ is $\frac{49}{3} s q . u n i t s$ if its point of intersection with $x = 5$ lies below the x-axis. Find the equation of $L_{2} .$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:x+y=2

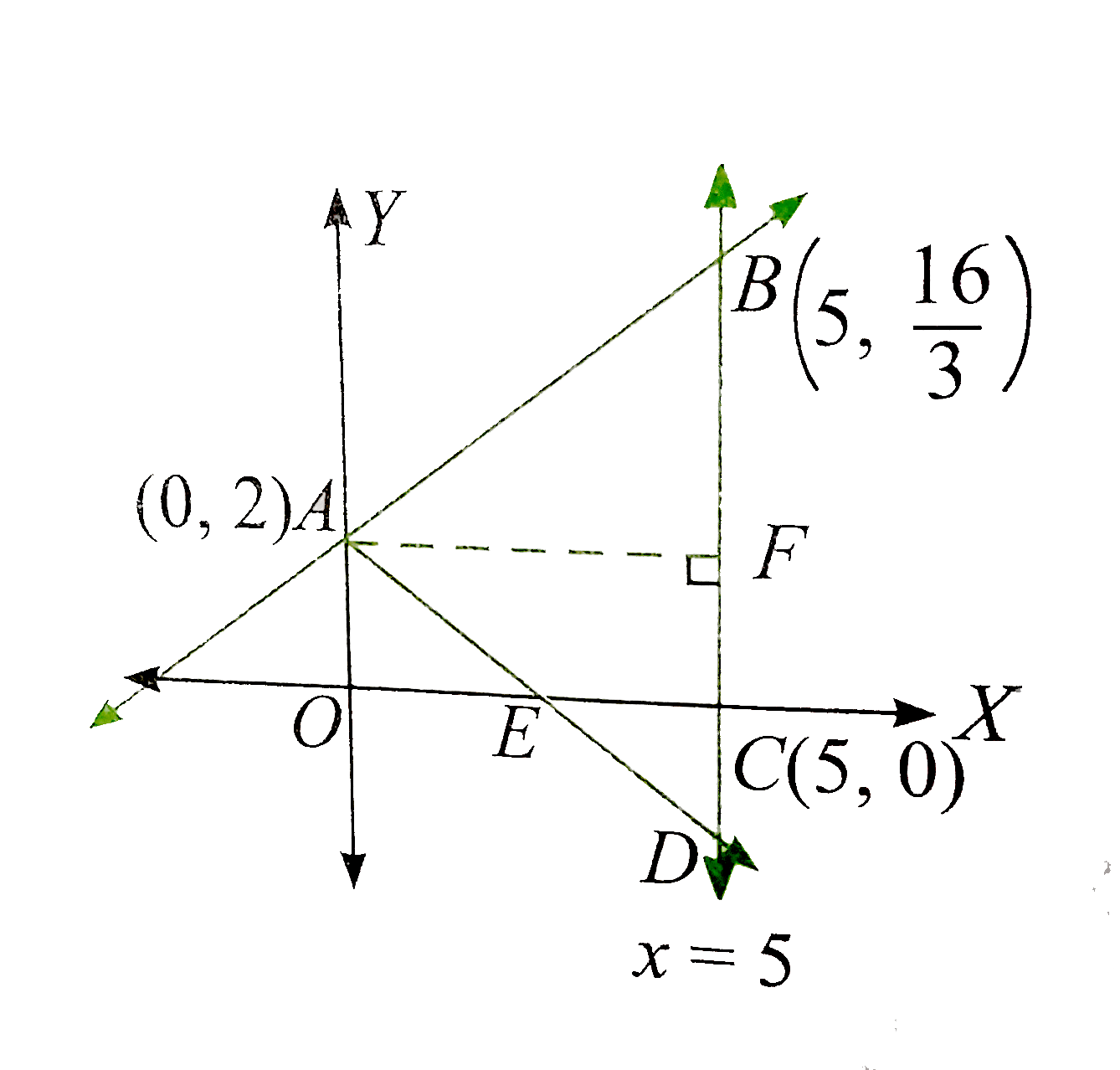
$L_{1} = 3 y - 2 x - 6 = 0$
The point about which the line is rotated is A = (0,2). Let the equation of $L_{2}$ be y = mx +2. As $L_{2}$ will be cutting line x=5 below the x-axis, we have
$A \equiv (0, 2), B \equiv (5, \frac{16}{3}), C \equiv (5, 0),$
$D \equiv (5, 5 m + 2), E \equiv (\frac{- 2}{m}, 0)$
$Now, Area of AECB = Area of Δ A D B - Area of Δ E C D$
$or \frac{49}{3} = \frac{1}{2} B D \times A F - \frac{1}{2} E C \times C D$
$= \frac{1}{2} [\frac{16}{3} - (5 m + 2)] \times 5 - \frac{1}{2} \times (5 + \frac{2}{m}) [- (5 m + 2)]$
$or \frac{49}{3} = \frac{110 m + 12}{6 m} or m = - 1$
$Hence, the equation of L_{2} is x + y = 2 .$

|

Updated on:21/07/2023

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

CENGAGE ENGLISH-STRAIGHT LINES-CONCEPT APPLICATION EXERCISE 2.1

Home
Ask Doubt
Profile