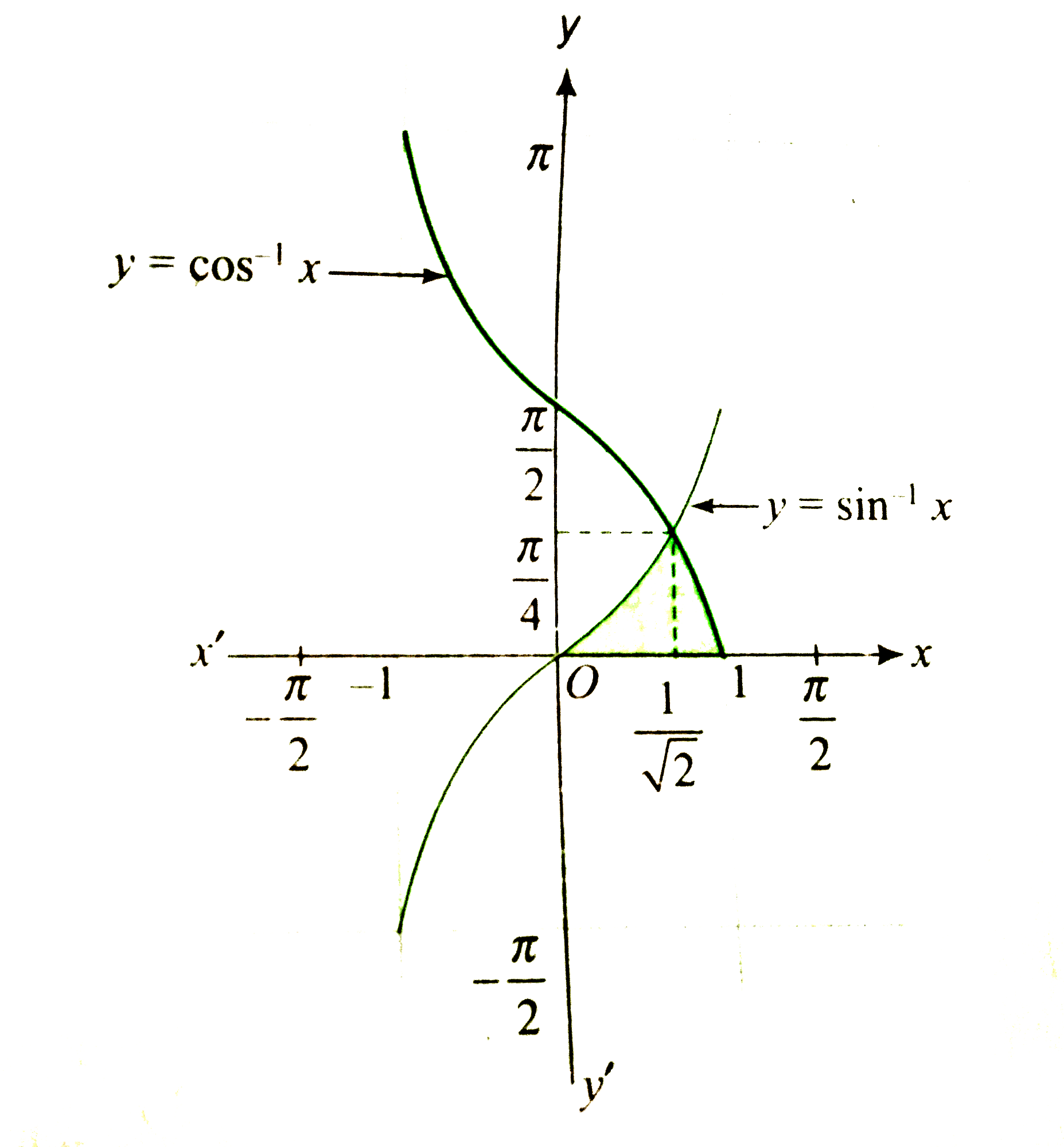
Find the area bounded by y=sin−1x,y=cos−1x ,and the X-axis.
We have to find the area bounded by y=sin−1x,y=cos−1x, and the x-axis. If integration along x-axis is used then
A=1/√2∫0sin−1xdx+1∫1√2cos−1xdx
If integration along y-axis is used, then
A=π/4∫0(cosy−siny)dy
=[siny+cosy]π/40
=[1√2+1√2−1]
=√2−1
Topper's Solved these Questions
- AREABOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Solved Examples
10 Videos
- AREABOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Concept Application Exercise 9.1
9 Videos
- APPLICATIONS OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Comprehension Type
5 Videos
- BINOMIAL THEOREMBOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix
4 Videos
Similar Questions
Find the area bounded by y=tan−1x,y=cot−1x,and y-axis in the first quadrant.
View SolutionFind the area bounded by y=cosx,y=x+1,y=0
View SolutionFide the area bounded by y=sin−1(sinx) and the x-axis for x∈[0,100π].
View SolutionFind the area bounded by the curve y=e−x the X-axis and the Y-axis.
View SolutionFind the area bounded by y=sin−1(sinx) and x-axis for x∈[0,100π]
View SolutionFind the area bounded by y=1+2sin2x,X-axis,X=0 and x=π.
View SolutionFind the area bounded by y=sin−1(sinx) and x-axis for x in [0,100π]
View SolutionThe area bounded by the curve y=sin−1(sinx) and the x - axis from x=0 to x=4π is equal to the area bounded by the curve y=cos−1(cosx) and the x - axis from x=−π to x=a, then the value of a is equal to
View SolutionFind the area bounded by the curve y=x(x−1)(x−2) and the x-axis.
View SolutionFind the area bounded by the curve y=sin−1x and the line x=0,|y|=π2.
View Solution
