The area inside the parabola 5x2−y=0 but outside the parabola 2x2−y+9=0 is (a) 12√3 sq units (b) 6√3 sq units (c) 8√3 sq units (d) 4√3 sq units
12√3 sq. units
6√3 sq. units
8√3 sq. units
4√3 sq. units
The correct Answer is:A
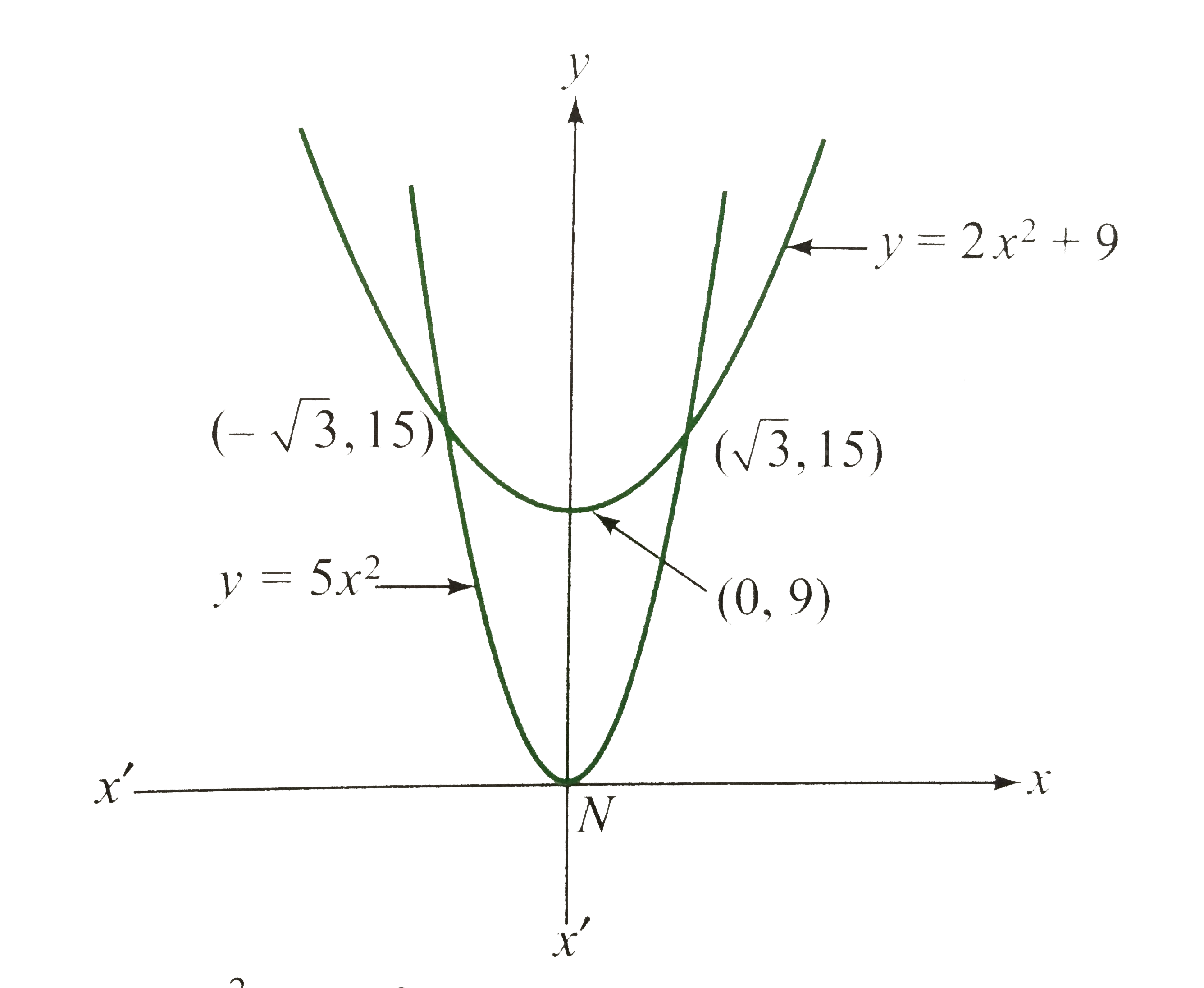
Given 5x2−y=0 ...(1)
and 2x2−y+9=0 ...(2)
5x2−(2x2+9)=0
or 3x2=9orx=−√3,√3
∴ Required area =2∫√30((2x2+9)−5x2)dx
=2∫√30(9−3x2)dx
=2[9x−x3]√30
=2[9√3−3√3]
=12√3 sq. units
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Multiple Correct Answers Type13videos
AREA
- BOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Linkded Comprehension Type21videos
AREA
- BOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Concept Application Exercise 9.37videos
AREA
- BOOK - CENGAGE ENGLISHCHAPTER - APPLICATIONS OF DERIVATIVESEXERCISE - Comprehension Type5videos
APPLICATIONS OF DERIVATIVES
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix4videos
BINOMIAL THEOREM
Similar Questions
The area inside the parabola 5x2−y=0 but outside the parabola 2x3−y+9=0 is
View SolutionThe area inside the parabola 5x2−y=0 but outside the parabola 2x2−y+9=0 is 12√3sq.units 6√3sq.units 8√3sq.units (d) 4√3sq.units
View Solutionthe distance between the points (3,−2) and (−3,2) is
a) √52 units
b) 4√10 units
c) 2√10 units
d) √40 unitsView SolutionThe area enclosed by 2|x|+3|y|≤6 is (a)3 sq. units (b) 4 sq. units (c)12 sq. units (d) 24 sq. units
View SolutionArea of the equilateral triangle inscribed in the parabola y2=4x, having one vertex at the vertex of the parabola is (A) 48 sq. untis (B) 48√3 sq. units (C) 16√3 sq. untis (D) none of these
View SolutionThe area bounded by the curves y=|x|−1andy=−|x|+1 is 1 sq. units (b) 2 sq. units 2√2 sq. units (d) 4 sq. units
View SolutionThe area bounded by the curves y=|x|−1andy=−|x|+1 is 1 sq. units (b) 2 sq. units 2√2 sq. units (d) 4 sq. units
View SolutionThe area bounded by the parabolas y=(x+1)2 and y=(x−1)2andy=(x−1)2 and the line y=14 is 4 sq. units (b) 1/6 sq. units 4/3 sq. units (d) 1/3 sq. units
View SolutionThe area bounded by the curve y=4x−x2 and x-axis is (A) 307 sq. units (B) 317 sq. units (C) 323 sq. units (D) 343 sq. units
View SolutionA parallelopiped is formed by planes drawn through the points (1,2,3) and (9,8,5) parallel to the coordinate planes. The length of its diagonal is (A) 2√14 units (B) 2√26 units (C) 6√3 units (D) 2√21 units
View Solution

