At a point P on the ellipse $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$ tangents PQ is drawn. If the point Q be at a distance $\frac{1}{p}$ from the point P, where 'p' is distance of the tangent from the origin, then the locus of the point Q is

A

(a) $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1 + \frac{1}{a^{2} b^{2}}$

B

(b) $\frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1 - \frac{1}{a^{2} b^{2}}$

C

(c) $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = \frac{1}{a^{2} b^{2}}$

D

(d) $\frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = \frac{1}{a^{2} b^{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Equation of the tangent at P is
$\frac{x - a cos θ}{a sin θ} = \frac{y - b sin θ}{- b cos θ}$

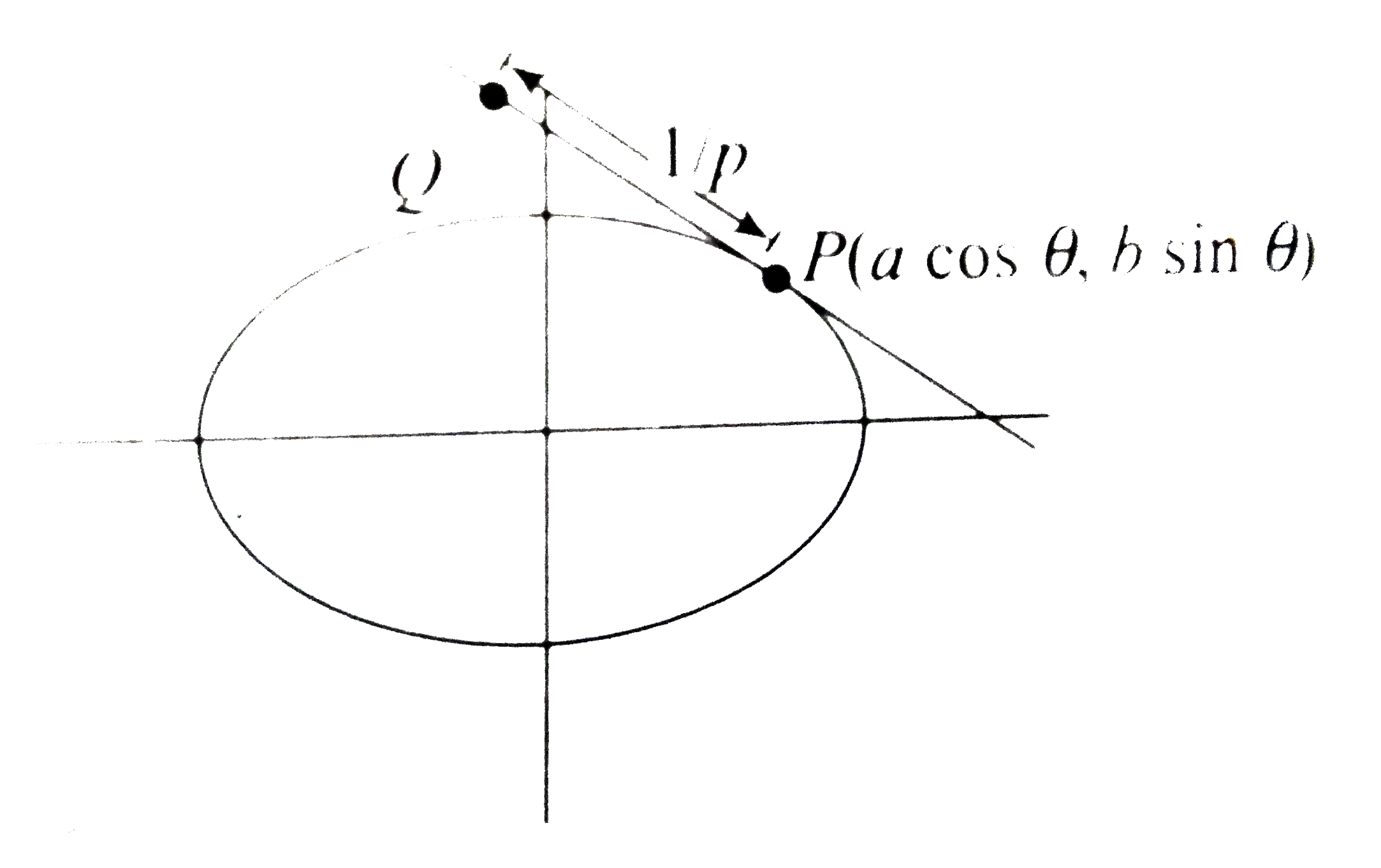
The distance of the tangent from the origin is
$p = ∣ ∣ ∣ ∣ \frac{a b}{\sqrt{b^{2} {cos}^{2} θ + a^{2} {sin}^{2} θ}} ∣ ∣ ∣ ∣$
$\Rightarrow \frac{1}{p} = \frac{\sqrt{b^{2} {cos}^{2} θ + a^{2} {sin}^{2} θ}}{a b}$
Now the coordinates of the point Q are given as follows
$\frac{\frac{x - a cos θ}{- a sin θ}}{\sqrt{b^{2} {cos}^{2} θ + a^{2} {sin}^{2} θ}} = \frac{\frac{y - b sin θ}{b cos θ}}{\sqrt{b^{2} {cos}^{2} θ + a^{2} {sin}^{2} θ}} = \frac{1}{p} = \frac{\sqrt{b^{2} {cos}^{2} θ + a^{2} {sin}^{2} θ}}{a b}$
$\Rightarrow x = a cos θ - \frac{a sin θ}{a b}$ and $y = b sin θ \frac{b cos θ}{a b}$
$\Rightarrow {(\frac{x}{a})}^{2} + {(\frac{y}{b})}^{2} = 1 + \frac{1}{a^{2} b^{2}}$ is the required locus.

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If P is the point (1,0) and Q is any point on the parabola $y^{2} = 8 x$ then the locus of mid - point of PQ is
A $P_{1}$ lies on $C_{2} and Q_{1}$ lies on $C_{1}$
B $P Q \geq min {P P_{1}, Q Q_{1}}$
Cpoint $P_{0}$ on $C_{1}$ such that $P_{0} Q_{0} \leq P Q$ for all pairs of points (P,Q) is $(\frac{1}{3}, \frac{10}{9})$
Dpoint $Q_{0}$ on $C_{2}$ such that $P_{0} Q_{0} \leq P Q$ for all pairs of points (P,Q) is $(\frac{10}{9}, \frac{1}{3})$

CENGAGE ENGLISH-ELLIPSE -Single Correct Answer Type

Home
Ask Doubt
Profile