Find the equation of the circle which is touched by y=x , has its center on the positive direction of the x=axis and cuts off a chord of length 2 units along the line √3y−x=0
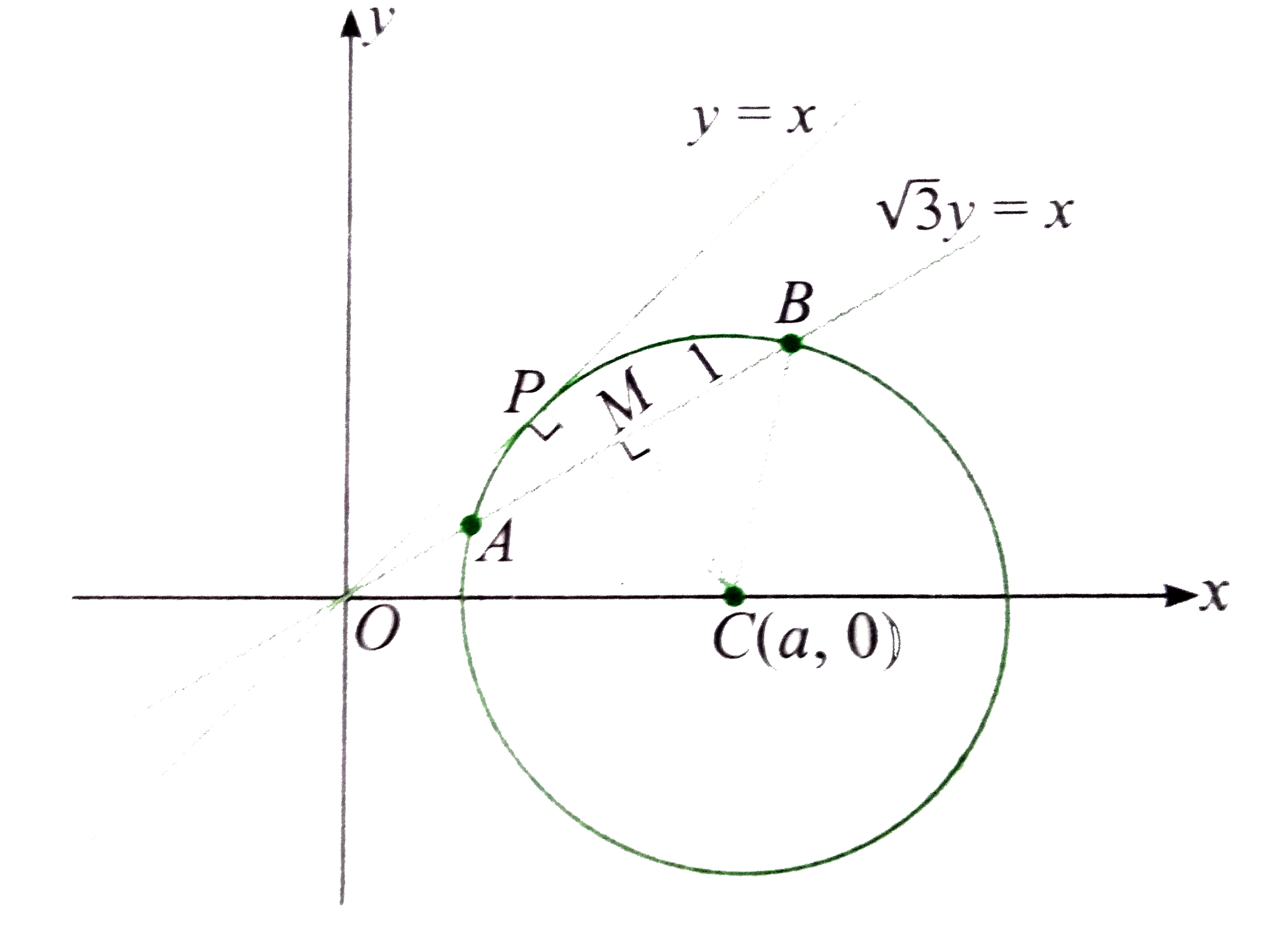
Centre C lies on x-axis.
∴C≡(a,0)
Line y=x touches the circle at point P.
So, radius of circle is the length of perpendicular (CP) from the centre C on the line y=x.
Also, length of chord (AB) of circle on line x−√3y=0 is 2 units.
∴MB=1( where m is the midpoint of AB).
Now, CP=CB (radii of circle)
∴CP2=CB2
⇒CP2=CM2+MB2
⇒⎛⎝|a−0|√12+(−1)2⎞⎠2=⎛⎜
⎜⎝∣∣a−√3(0)∣∣√(3)2+(1)2⎞⎟
⎟⎠2+12
⇒a22=a24+1
⇒a24=1
⇒a=2 ( as centre lies in first quadrant)
Also, radius CP=a√2=√2
Therefore , equation of the circle is
(x−2)2+(y−0)2=2
or x2+y2−4x+2=0
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Examples
13 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.1
1 Videos
- BINOMIAL THEOREMBOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix
4 Videos
- CIRCLESBOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
Find the equation of the line which cuts off an intercept 4 on the positive direction of x-axis and an intercept 3 on the negative direction of y-axis.
View SolutionFind the equation of the line which intercepts a length 2 on the positive direction of the x-axis and is inclined at an angle of 135∘ with the positive direction of y-axis .
View SolutionFind the equation of the line which cuts off an intercept 4 on negative direction of x-axis and an intercept of 2 on positive direction of y-axis.
View SolutionFind the equation of the circle which passes through the origin and cuts off chords of lengths 4 and 6 on the positive side of the x-axis and y-axis respectively.
View SolutionThe equation of the cirlce with centre (0,0) and which cuts off a chord of length 4 units on x+2y=5 is
View SolutionFind the equation of the straight line perpendicular to 2x−3y=5 and cutting off an intercept 1 on the positives direction of the x-axis.
View SolutionFind the equation of the circle which touches the y-axis at a distance of +4 from the origin and cuts off an intercept 6 from the x-axis.
View SolutionFind the equation of the circles which touch the axis of x at a distance of 4 from the origin and cut off an intercept of 6 from the axis of y.
View SolutionFind the equation of the circle which touches the axis of x at a distance 3 from the origin and cuts an intercept of length 6 on the axis of y.
View SolutionEquation to the circles which pass through the point (2,3) and cut off equal chords of length 6 units along the lines y-x-1=0 and y+x-5=0 is
View Solution
