Question

A circle touches the y-axis at the point (0, 4) and cuts the x-axis in
a chord of length 6 units. Then find the radius of the circle.

Explanation

Circles touch the y-axis at point P(0,4).

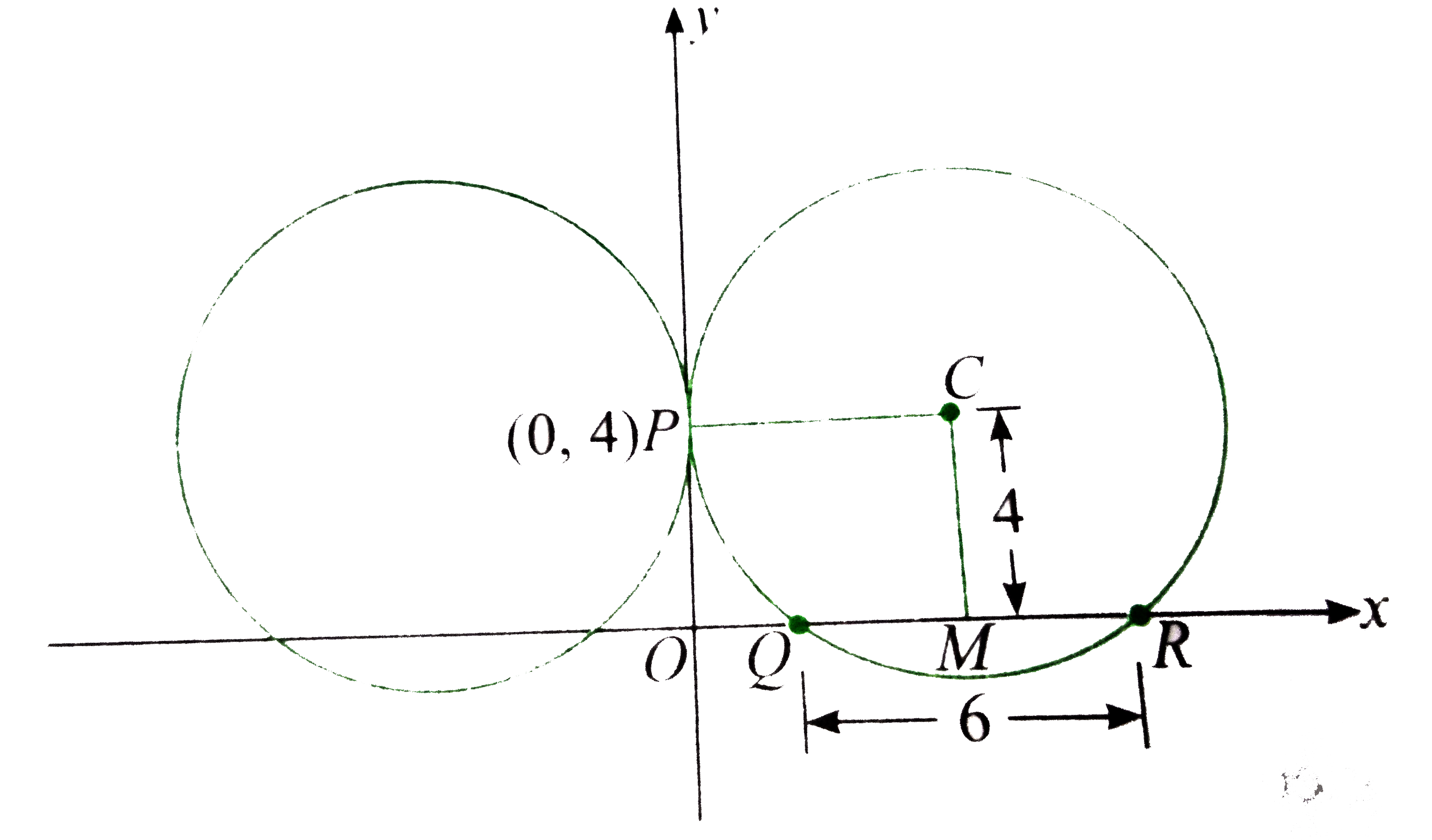
Intercept QR of circle on x-axis is 6 units
`:. QM =3`
Also, C is centre of one of the circles
`:. CM=4`
In triangle CMQ , we have
`CQ^(2)=QM^(2)+CM^(2)=3^(2)+4^(2)`
`:. CQ=5=CP=` radius
Therefore, centre of the circle lying in first quadrant is C(5,4). Thus, equation of circle is `(x-5)^(2)+(y-4)^(2)=25`.
Equation of circle having centre C(-5,4) in secong quadrant is `(x+5)^(2)+(y-4)^(2)=25`.