Find the area of the region in which the points satisfy the inequaties 4<x2+y2<16 and 3x2−y2>0
Video Solution
Text Solution
The points satisfying 4<x2+y2<16 lie in the region outside the circle x2+y2=4 and inside the circle x2+y2=16 as shown in the following figure.
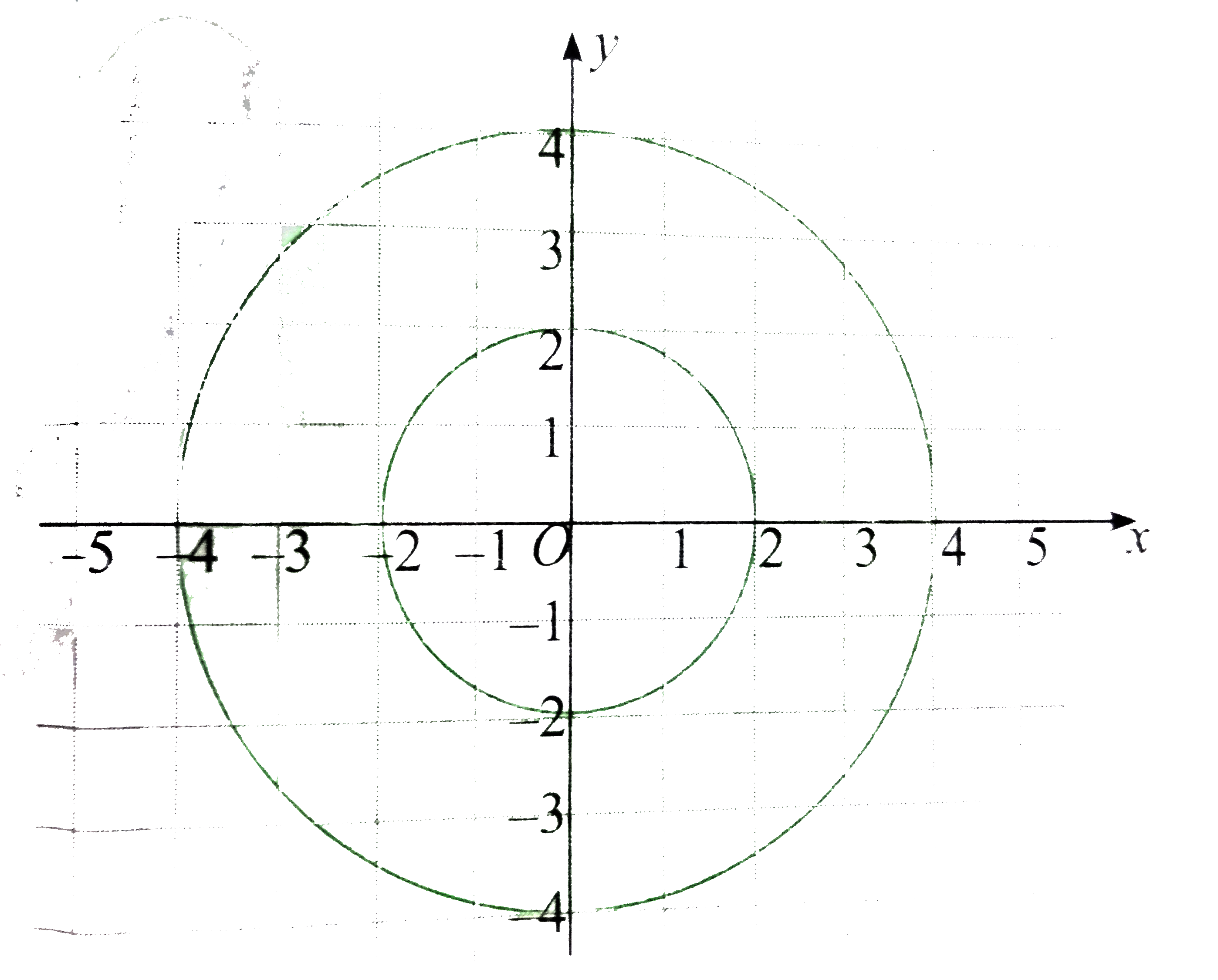
Now, 3x2−y2>0
∴(√3x−y)(√3x+y)>0
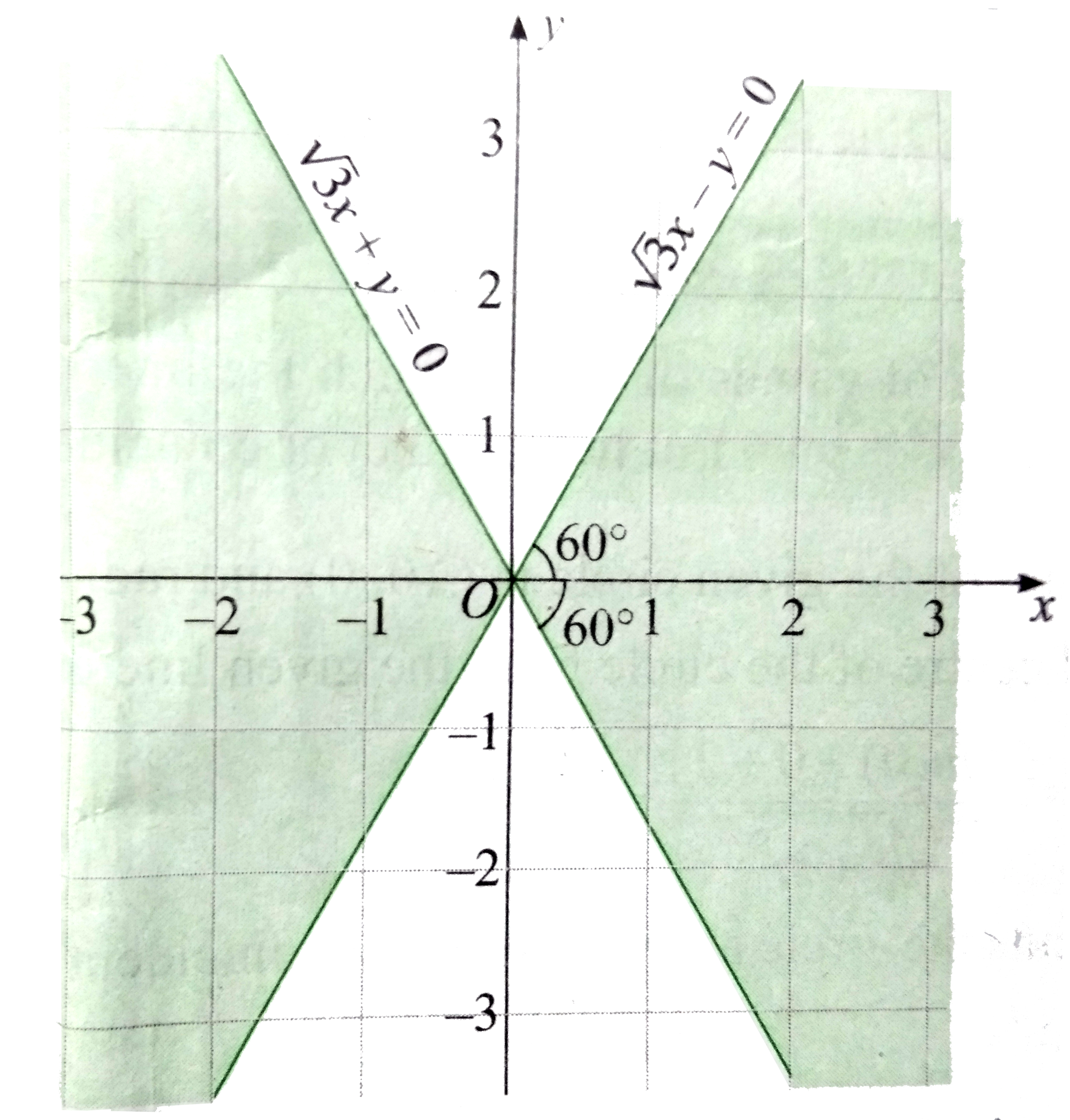
Now, draw the lines √3x−y=0 and √3x+y=0.
Therefore, 3x2−y2>0 represents the following region :
Hence, the region of the points satisfying both the inequalities is shown in the following figure.
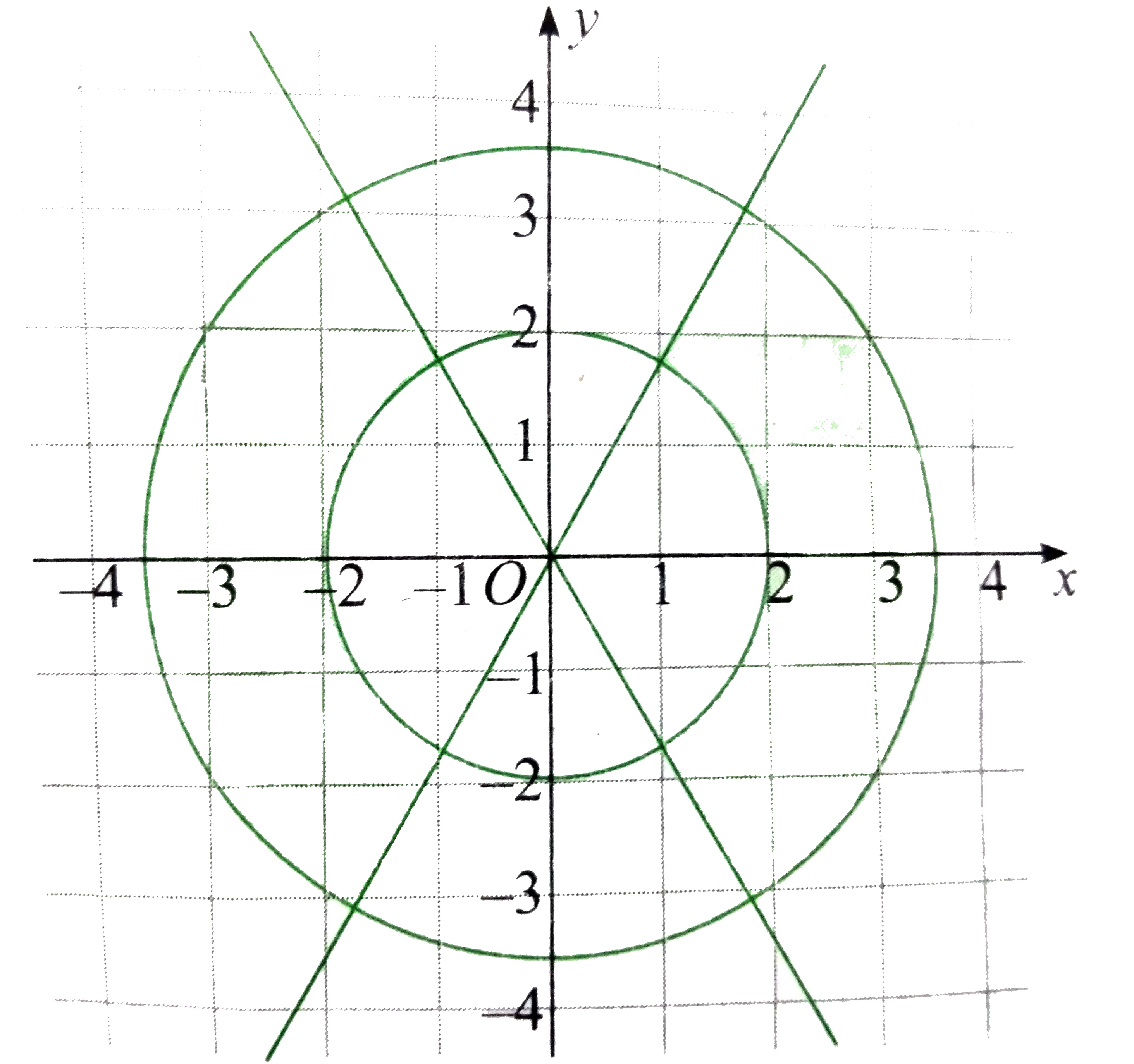
Hence, the required area is
46[π(4)2−π(2)2]=8π
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Examples13videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.11videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix4videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
Similar Questions
Find the area of the region in which the points satisfy the inequaties 4<x2+y2<16 and 3x2−y2≥0.
View SolutionFind the area of the region in which points satisfy
3≤|x|+|y|≤5.View SolutionFind the area of the shaded region in figure.
 View Solution
View SolutionFind the area of the shaded region in Figure.
View SolutionFind the area of the region which is inside the parabola satisfying the condition |x−2y|+|x+2y|≤8 and xy≥2.
View SolutionFind tha area of the region containing the points (x,y) satisfying 4≤x2+y2≤2(|x|+|y|).
View SolutionFind the area of the shaded region in the adjoing figure :
 View Solution
View SolutionFind the area of the shaded region in the adjoing figure :
 View Solution
View SolutionFind the area of the shaded region in the adjoing figure :
 View Solution
View SolutionFind the area of the shaded region in the adjoing figure :
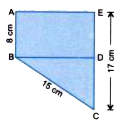 View Solution
View Solution

