Find the coordinates of the point at which the circles $x^{2} + y^{2} - 4 x - 2 y + 4 = 0$ and $x^{2} + y^{2} - 12 x - 8 y + 36 = 0$ touch each other. Also, find equations of common tangents touching the circles the distinct points.

Video Solution

Text Solution

Verified by Experts

The given circles are
$x^{2} + y^{2} - 4 x - 2 y + 4 = 0$ (1)
and $x^{2} + y^{2} - 12 x - 8 y + 36 = 0$ (2)
with centers $C_{1} (2, 1)$ and $C_{2} (6, 4)$ and radii 1 and 4 , respectively. Also, $C_{1} C_{2} = 5$ .
As $r_{1} + r_{2} = C_{1} C_{2}$ , the two circles touch each other externally at P.

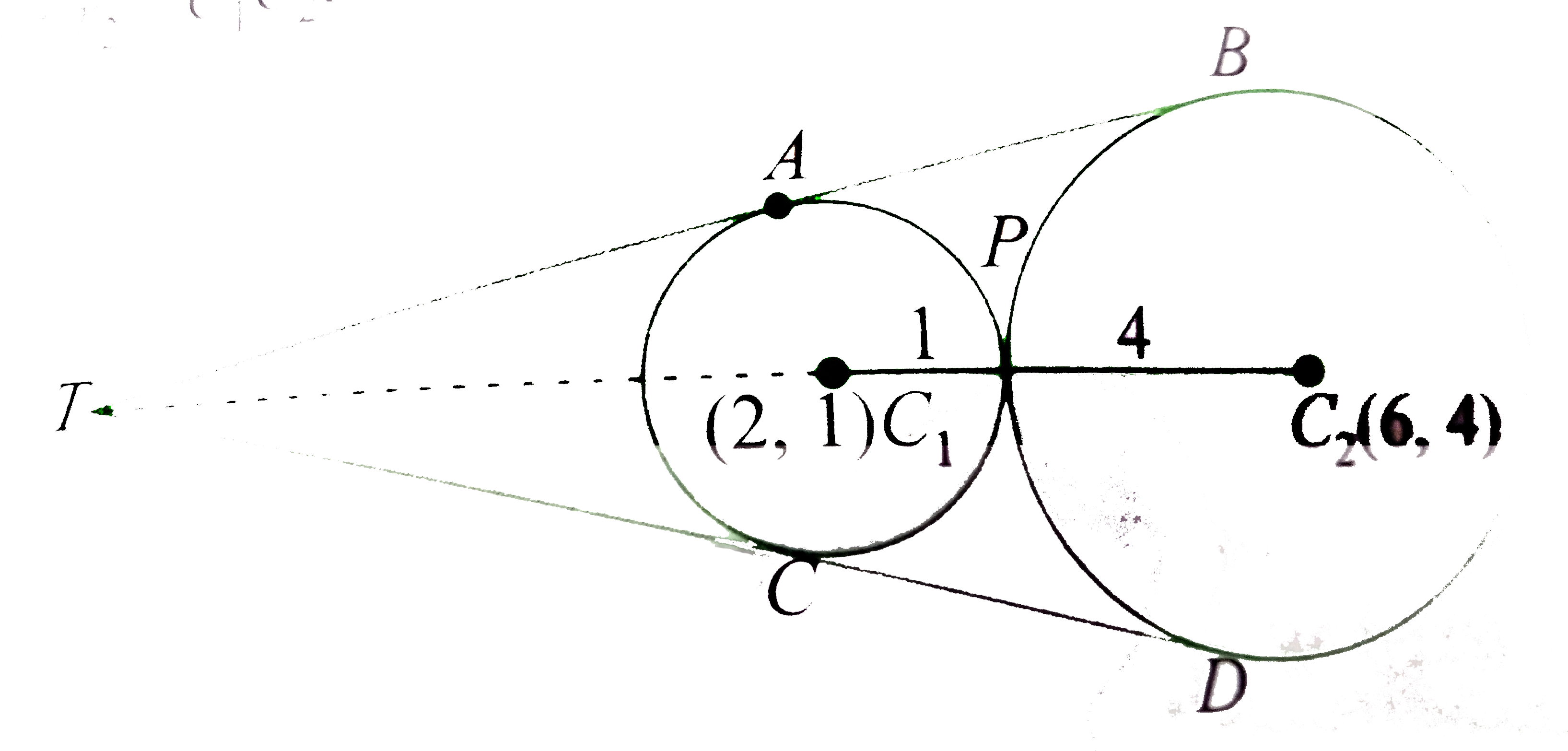
Clearly, P divides $C_{1} C_{2}$ in the ratio $1 : 4$ $, < b r > T h e r e f or e, t h e c \infty r d \in a t e s o f P a r e < b r >$ ((1xx6+4xx2)/(1+4),(1xx4+4xx1)/(1+4))-=((14)/(5),(8)/(5)) $< b r > L e t A B and C D b e t w o c o m m o n tan \geq n t s o f t h e g i v e n \circ \leq s, m e e t \in g e a c h o t h e r a t T . < b r > T h e n T \div s$ C_(1)C_(2) $e x t e r n a l l y \in t h e r a t i o$ 1:4 $. H e n c e, < b r >$ T-= ((1 xx 6-4xx2)/(1-4),(1xx4-4xx1)/(1-4))-=((2)/(3),0) $< b r > L e t m b e t h e s l o p e o f t h e tan \geq n t . T h e n t h e e q u a t i o n o f tan \geq n t t h r o u g h$ (2//3,0) $i s < b r >$ y-0=m(x-(2)/(3)) $< b r > or$ y-mx+(2)/(3)m=0 $< b r > N o w, t h e \leq n > h o f p e r p e n d i c a - - r o m (2, 1) \to t h e a b o v e tan \geq n t i s t h e r a d i u s o f t h e \circ \leq . T h e r e f or e, < b r >$ |(1-2m+(2)/(3)m)/(sqrt(m^(2))+1)|=1 $< b r > or$ (3-4m)^(2)=9(m^(2)+1) $< b r > or$ 9-24m+16m^(2)=9m^(2)+9 $< b r > or$ 7m^(2)-24m=0 $< b r > or$ m=0,(24)/(7) $< b r > T h u s, t h e e q u a t i o n s o f t h e tan \geq n t s a r e$ y=0 $and$ 7y-24x+16=0.

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

CENGAGE ENGLISH-CIRCLE -CONCEPT APPLICATION EXERCISE 4.10

Home
Ask Doubt
Profile