In a ΔABC, if median AD is perpendicular to AB, the tanA+2tanB is equal to
tan A + tan B = 0
2 tan A + tan B = 0
tan A + 2tan B = 0
none of these
The correct Answer is:C
We have BD = DC and ∠DAB=90∘. Draw CN perpendicular to BA produced, then in ΔBCN, we have DA=12CN and AB = AN

Let ∠CAN=α
∵tanA=tan(π−α)
=−tanα
=−CNNA
=−2ADAB(∵ΔBAD~ΔBNC and AC=2BD)
=−2tanB
⇒tanA+2tanB=0
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Archives JEE Advanced Multiple correct answers type
1 Video
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)
1 Video
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Matrix Match Type
1 Video
Similar Questions
tan3A−tan2A−tanA= is equal to
View SolutionIn a ΔABC, the median AD is perpendicular to AC. If b = 5 and c = 11, then a =
View SolutionIf the median of a triangle through A is perpendicular to AB, then
View SolutionIn the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that :
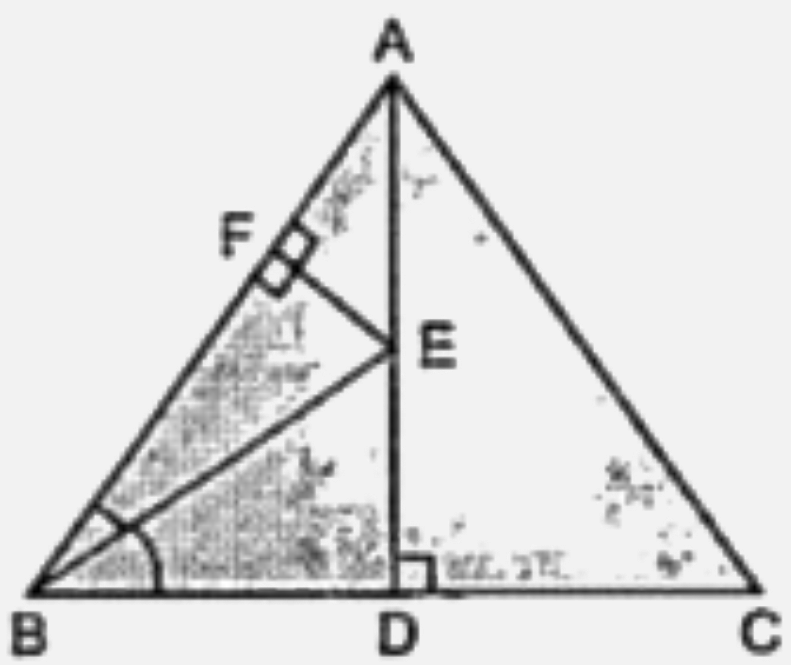
ED=EF
View SolutionIn the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that : ED=EF
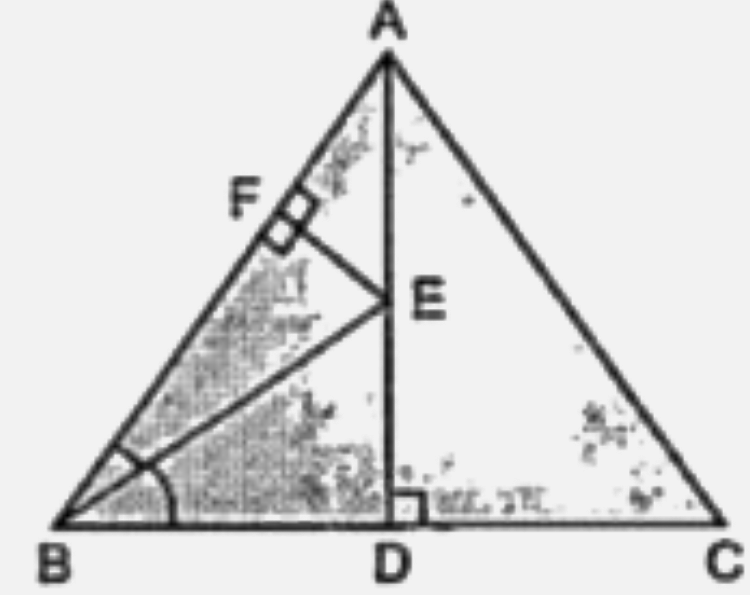
View SolutionIf the median of triangle ABC through A is perpendicular AB, then the value of sinA cosB + 2sinB cosA is equal to
View SolutionIn Triangle ABC, AD is perpendicular to BC, tanB=34tanC=512 and BC = 56 cm . Calculate the lengths of AD.
View SolutionIn any ΔABC the value of 1−tanB2tanC2 is equal to :-
View SolutionIf A(4,−6),B(3,−2)andC(5,2) are the vertices of a ΔABCandAD is its median, prove that the median AD divides ΔABC into two triangles of equal areas.
View SolutionIf in a triangle ABC, b + c = 3a, then tan(B2)tan(C2) is equal to
View Solution