consider the graph of y=g(x)=f'(x) given that f(c ) =0, where y=f(x) is a polynomial funtion

The graph of y=f(x) will intersect the x axis
A
twice
B
once
C
never
D
none of these
Text Solution
The correct Answer is:2
f(X)≤0∀x∈[a,b] so f(X) is a decreasing funciton and f(c )=0 Thus f(x) cuts x axis once whne x=c
|
Topper's Solved these Questions
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Matrix Match Type
14 Videos
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Linked comprehension Type
2 Videos
- METHODS OF DIFFERENTIATIONBOOK - CENGAGE ENGLISHCHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - Single Correct Answer Type
46 Videos
- MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSEXERCISE - Comprehension Type
6 Videos
Similar Questions
Knowledge Check
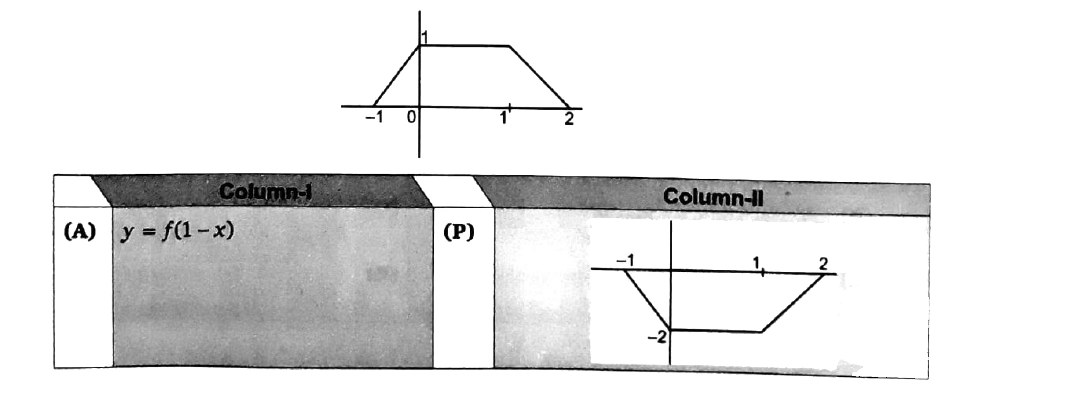
The graph of y=f(x) is given below
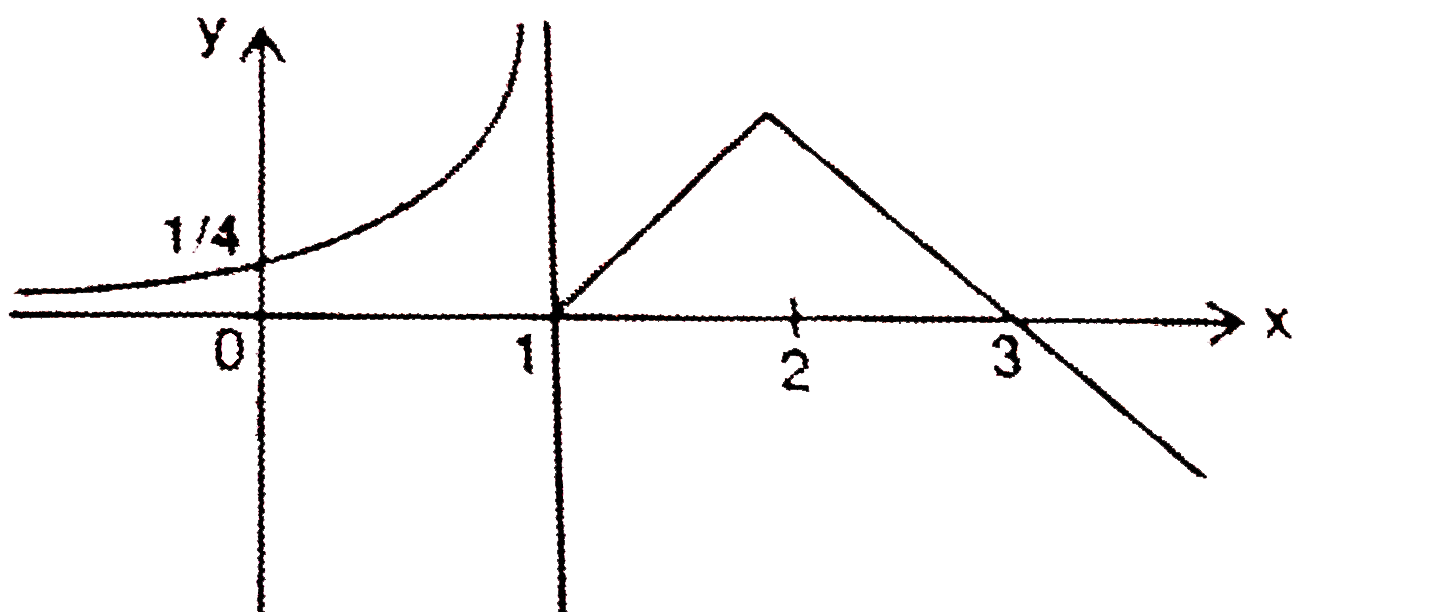
then the graph y=|f(|x|)| is :A B
B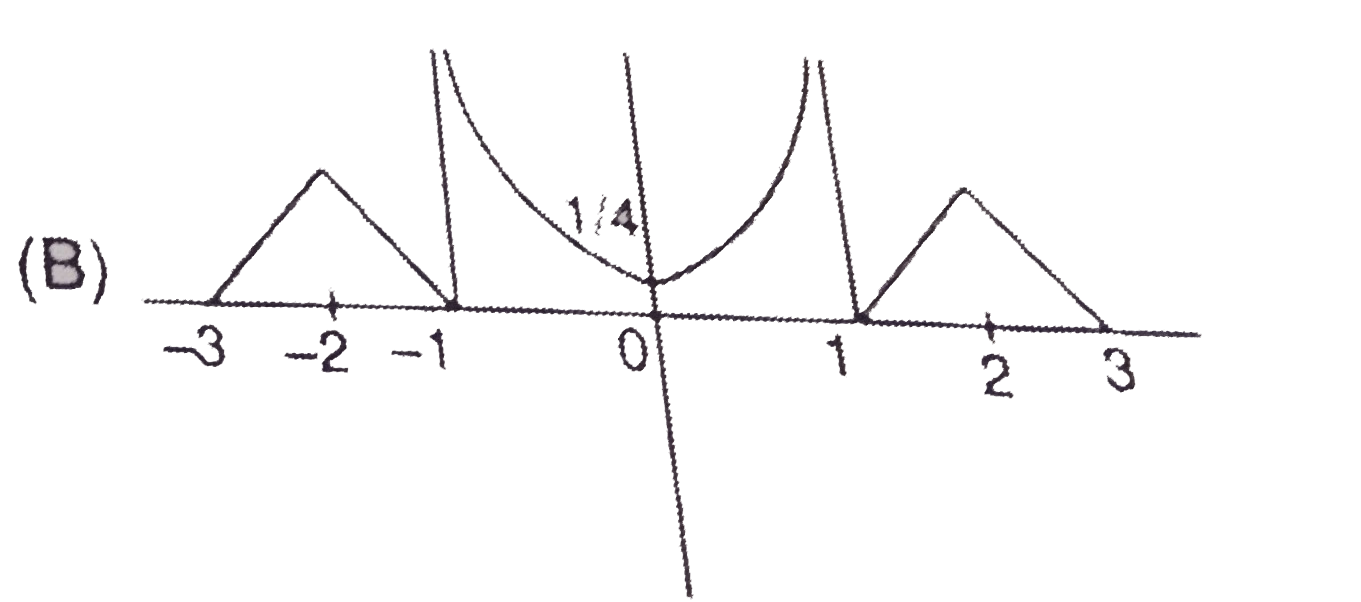 C
C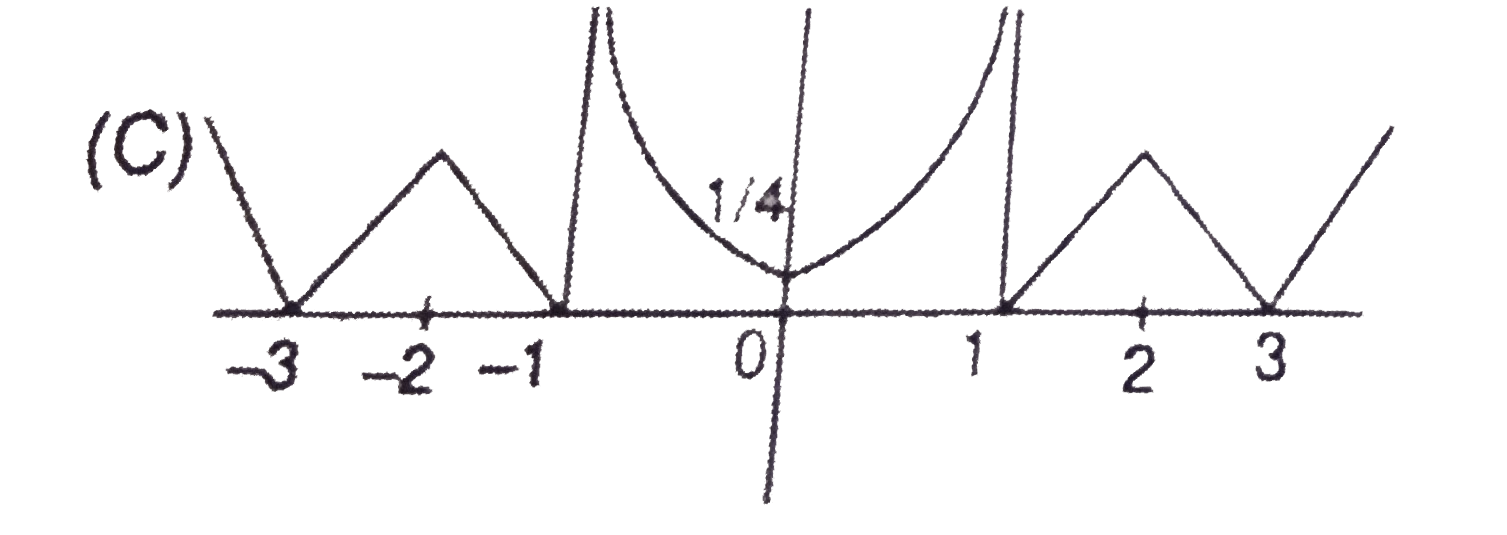 Dnone of these
Dnone of theseIf f (x) is an even function, then the graph y = f (x) will be symmetrical about
Ax-axisBy-axisCBoth the axesDNone of these
Graph of y=f(x) is given, then
View SolutionThe graph of y=f(x) is given below

then the graph y=|f(|x|)| is :
View SolutionThe graph y = f (x) is as shown:
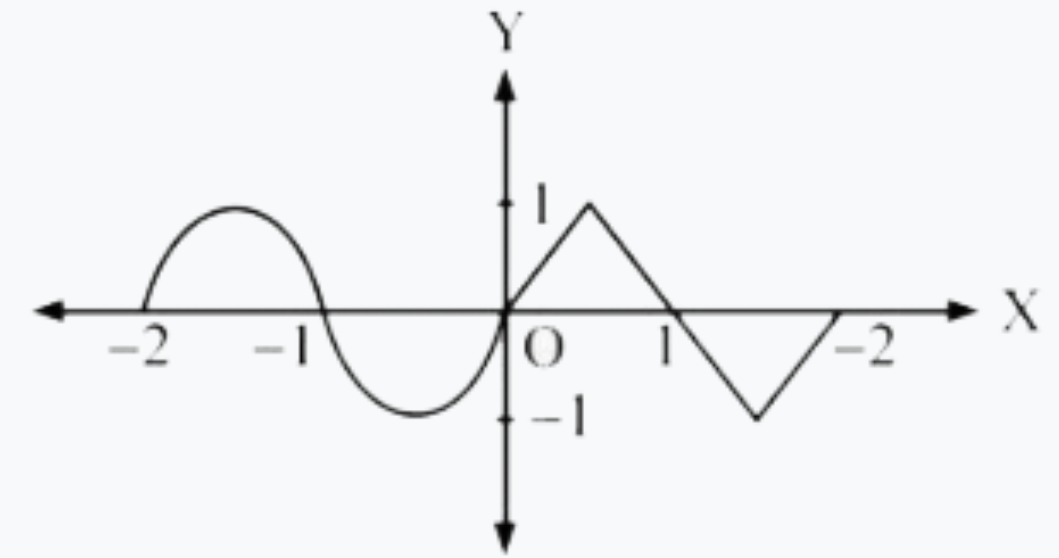
The graph of y = f (–|x|) is:
View SolutionThe graph y = f (x) is as shown:

The graph of y = f (–|x|) is:
View SolutionIf the graph of y=|f(x)| is as shown in figure
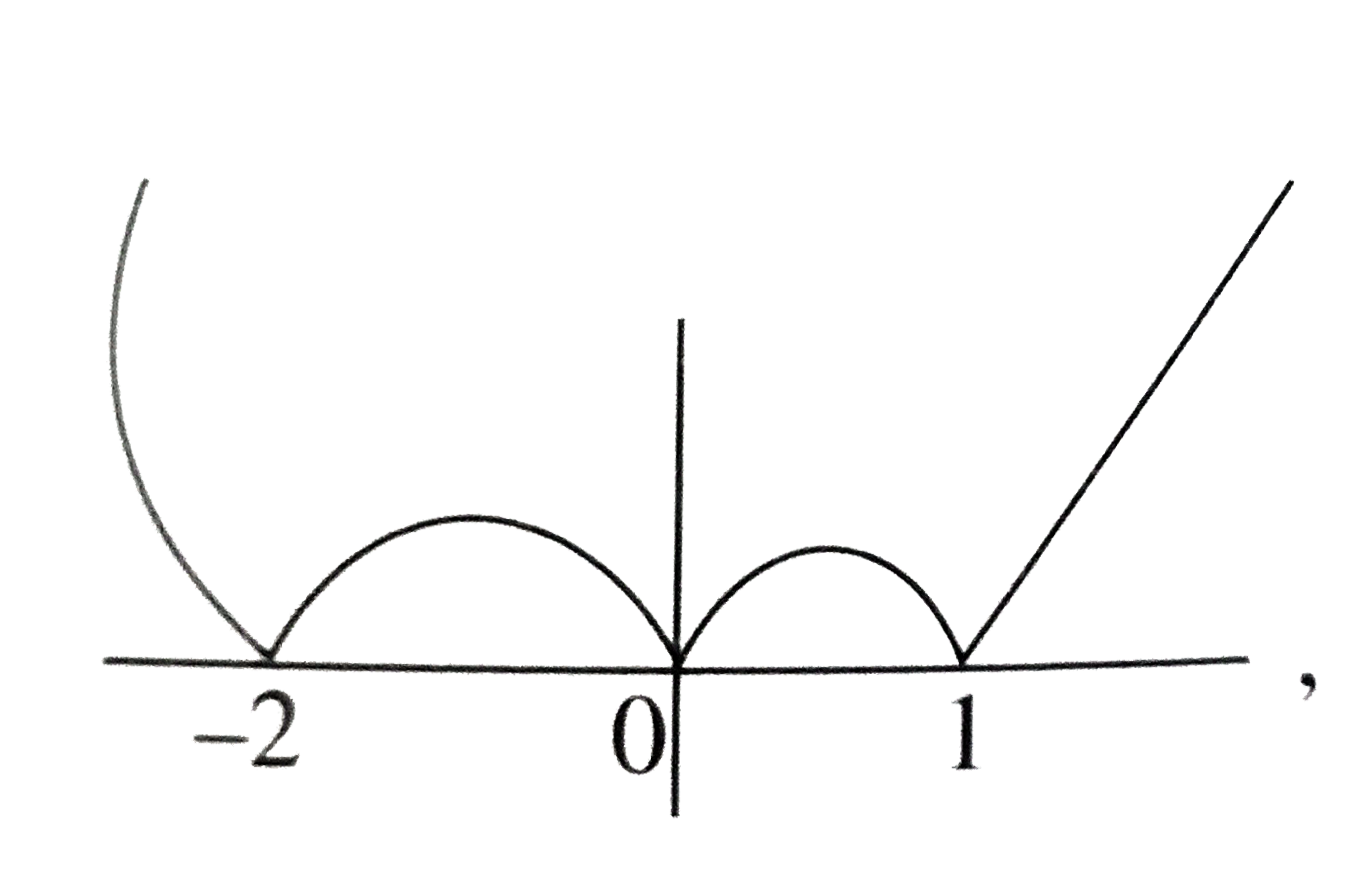 then the graph of y=f(x) may be
then the graph of y=f(x) may be
View SolutionIf the graph of y=|f(x)| is as shown in figure
 then the graph of y=f(x) may be
then the graph of y=f(x) may be
View Solution