A function f is defined on an interval [a, b]. Which of the following statement(s) is are incorrect? (A) If f(a) and f(b) have opposite signs,then there must be a point c∈(a,b) such that f(c)=0.
if f(a ) and f(b) have opposite sings then there must be a point c∈(a,b) such that f(c ) =0
if f is continuous on [a,b],f(a)<0,andf(b)>0, then there must be point c∈(a,b) such that f( c) =0.
if f is continuous on [a,b] , and there is point c in (a,b) such that f( c) -0 , then f(a) and f(b) have opposite signs
if f has no zeros on [a,b] then f(a) and f(b) have the same sign.
The correct Answer is:A, C, D
To determine which statements about the function f defined on the interval (a,b) are incorrect, we need to analyze the given statement (A) and compare it with the properties of continuous functions.
1. Understanding the Statement (A):
- The statement says: "If f(a) and f(b) have opposite signs, then there must be a point c∈(a,b) such that f(c)=0."
- This is a direct application of the Intermediate Value Theorem (IVT), which states that if a function is continuous on an interval and takes on opposite signs at the endpoints, then it must cross zero at some point within the interval.
2. Checking Continuity:
- For the statement to hold true, the function f must be continuous on the interval (a,b). The statement does not specify that f is continuous, but it is implied in the context of the IVT.
3. Conclusion for Statement (A):
- If f(a) and f(b) have opposite signs and f is continuous on (a,b), then there exists at least one point c∈(a,b) such that f(c)=0. Therefore, statement (A) is correct.
4. Analyzing Other Statements:
- Without loss of generality, let's assume there are other statements (B and C) that need to be evaluated similarly.
- For example, if statement (B) states that "If f(a)<0 and f(b)>0, then there exists a point c∈(a,b) such that f(c)=0", this would also be true under the assumption of continuity.
- If statement (C) states that "If f is continuous on (a,b) and f(a) and f(b) have opposite signs, then there exists a point c∈(a,b) such that f(c)=0", this is also true.
5. Identifying Incorrect Statements:
- If any of the statements (B or C) contradict the properties of continuous functions or the IVT, then those would be marked as incorrect. In the provided video transcript, it is suggested that statement (C) is incorrect, but this contradicts the established properties of continuous functions.
Final Answer:
- The statement (A) is correct.
- If statement (C) is indeed claimed to be incorrect, it should be verified against the properties of continuity and the IVT. However, based on standard mathematical principles, statement (C) should also be correct.
- The statement says: "If f(a) and f(b) have opposite signs, then there must be a point c∈(a,b) such that f(c)=0."
- This is a direct application of the Intermediate Value Theorem (IVT), which states that if a function is continuous on an interval and takes on opposite signs at the endpoints, then it must cross zero at some point within the interval.
- For the statement to hold true, the function f must be continuous on the interval (a,b). The statement does not specify that f is continuous, but it is implied in the context of the IVT.
- If f(a) and f(b) have opposite signs and f is continuous on (a,b), then there exists at least one point c∈(a,b) such that f(c)=0. Therefore, statement (A) is correct.
- Without loss of generality, let's assume there are other statements (B and C) that need to be evaluated similarly.
- For example, if statement (B) states that "If f(a)<0 and f(b)>0, then there exists a point c∈(a,b) such that f(c)=0", this would also be true under the assumption of continuity.
- If statement (C) states that "If f is continuous on (a,b) and f(a) and f(b) have opposite signs, then there exists a point c∈(a,b) such that f(c)=0", this is also true.
- If any of the statements (B or C) contradict the properties of continuous functions or the IVT, then those would be marked as incorrect. In the provided video transcript, it is suggested that statement (C) is incorrect, but this contradicts the established properties of continuous functions.
- The statement (A) is correct.
- If statement (C) is indeed claimed to be incorrect, it should be verified against the properties of continuity and the IVT. However, based on standard mathematical principles, statement (C) should also be correct.
Similar Questions
Let f be a differentiable function on the open interval(a, b). Which of the following statements must be true?
(i) f is continuous on the closed interval [a,b],
(ii) f is bounded on the open interval (a,b)
(iii)If a < a1< b1 < b, and f(a1) < 0 < f(b1), then there is a number c such that a1 < c < b, and f(c) = 0
(a)Only I and II
(b)Only I and III
(c)Only II and III
(d)Only III
View SolutionLet fandg be function continuous in [a,b]and differentiable on [a,b].If f(a)=f(b)=0 then show that there is a point c∈(a,b) such that g'(c)f(c)+f'(c)=0.
View Solution
Knowledge Check
If f is an odd function and f(a)=b, which of the following must also be true ?
I. f(a)=−b
II.f(−a)=b
III. f(−a)=−bAonly IBOnly IIICOnly IIIDOnly I and II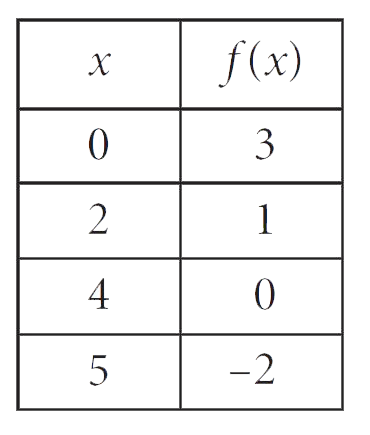
The function f is defined by a polynomial. Some values of x and f x( ) are shown in the table above. Which of the following must be a factor of f x( ) ?Ax-2Bx-3Cx-4Dx-5If point (a,b) lies on the graph of function f, which of the following points must lie on the graph of the inverse f?
A(a,b)B(-a,b)C(a,-b)D(b,a)
Statement 1: If both functions f(t)andg(t) are continuous on the closed interval [a,b], differentiable on the open interval (a,b) and g′(t) is not zero on that open interval, then there exists some c in (a,b) such that f′(c)g′(c)=f(b)−f(a)g(b)−g(a) Statement 2: If f(t)andg(t) are continuous and differentiable in [a, b], then there exists some c in (a,b) such that f′(c)=f(b)−f(a)b−aandg′(c)g(b)−g(a)b−a from Lagranges mean value theorem.
View SolutionLet f be a continuous, differentiable, and bijective function. If the tangent to y=f(x)atx=a is also the normal to y=f(x)atx=b, then there exists at least one c∈(a,b) such that (a)f′(c)=0 (b) f′(c)>0 (c)f′(c)<0 (d) none of these
View SolutionLet f be a continuous, differentiable, and bijective function. If the tangent to y=f(x)atx=a is also the normal to y=f(x)atx=b, then there exists at least one c∈(a,b) such that f′(c)=0 (b) f′(c)>0 f′(c)<0 (d) none of these
View SolutionFor a function f:A→B such that n(A)=a,n(B)=b(a,b∈N) then which of the following statements must be CORRECT?
View SolutionThe function f(x) is defined by f(x)={x2+ax+b , 0≤x<2, 3x+2 , 2≤x≤4, 2ax+5b , x>4 f is continuous them determine the value of a and b
View Solution
