A swimming pool is to be drained by cleaning. If L represents the number of litres of water in the pool t seconds after the pool has been plugged off to drain and L=2000(10−t)2. How fast is the water ruining out at the end of 5 seconds? What is the average rate at which the water flows out during the first 5 seconds?
To solve the problem step by step, we will follow these steps:
Step 1: Understand the given function
We are given the function for the volume of water in the pool as:
L=2000(10−t)2
where L is the number of liters of water in the pool and t is the time in seconds after the pool has been plugged off.
Step 2: Find the derivative dLdt
To find out how fast the water is draining out, we need to compute the derivative of L with respect to t:
dLdt=ddt(2000(10−t)2)
Using the chain rule:
dLdt=2000⋅2(10−t)⋅ddt(10−t)
dLdt=2000⋅2(10−t)⋅(−1)
dLdt=−4000(10−t)
Step 3: Evaluate dLdt at t=5 seconds
Now we will substitute t=5 into the derivative to find how fast the water is draining out at that moment:
dLdt∣∣∣t=5=−4000(10−5)
dLdt∣∣∣t=5=−4000⋅5
dLdt∣∣∣t=5=−20000 liters per second
Step 4: Calculate the average rate of water flow out during the first 5 seconds
To find the average rate of water flow out during the first 5 seconds, we need to find the initial rate at t=0 and the final rate at t=5.
1. Initial rate at t=0:
dLdt∣∣∣t=0=−4000(10−0)
dLdt∣∣∣t=0=−4000⋅10
dLdt∣∣∣t=0=−40000 liters per second
2. Final rate at t=5:
We already calculated this as:
dLdt∣∣∣t=5=−20000 liters per second
3. Average rate:
The average rate can be calculated using:
Average Rate=Initial Rate+Final Rate2
Average Rate=−40000+(−20000)2
Average Rate=−600002
Average Rate=−30000 liters per second
Final Answers:
- The rate at which water is draining out at the end of 5 seconds is 20000 liters per second.
- The average rate at which water flows out during the first 5 seconds is 30000 liters per second.
We are given the function for the volume of water in the pool as:
L=2000(10−t)2
where L is the number of liters of water in the pool and t is the time in seconds after the pool has been plugged off.
To find out how fast the water is draining out, we need to compute the derivative of L with respect to t:
dLdt=ddt(2000(10−t)2)
dLdt=2000⋅2(10−t)⋅ddt(10−t)
dLdt=2000⋅2(10−t)⋅(−1)
dLdt=−4000(10−t)
Now we will substitute t=5 into the derivative to find how fast the water is draining out at that moment:
dLdt∣∣∣t=5=−4000(10−5)
dLdt∣∣∣t=5=−4000⋅5
dLdt∣∣∣t=5=−20000 liters per second
To find the average rate of water flow out during the first 5 seconds, we need to find the initial rate at t=0 and the final rate at t=5.
dLdt∣∣∣t=0=−4000(10−0)
dLdt∣∣∣t=0=−4000⋅10
dLdt∣∣∣t=0=−40000 liters per second
We already calculated this as:
dLdt∣∣∣t=5=−20000 liters per second
The average rate can be calculated using:
Average Rate=Initial Rate+Final Rate2
Average Rate=−40000+(−20000)2
Average Rate=−600002
Average Rate=−30000 liters per second
- The rate at which water is draining out at the end of 5 seconds is 20000 liters per second.
- The average rate at which water flows out during the first 5 seconds is 30000 liters per second.
Topper's Solved these Questions
Similar Questions
A swimming pool is filled by three pipes with uniform flow. The first two pipes operating simultaneously fill the pool in the same time during which the pool is filled by the third pipe alone. The second pipe fills the pool 5 hours faster than the first pipe and 4 hours slower than the third pipe. The time required by the first pipe is 6 hrs b. 10 hrs c. 15 hrs d. 30 hrs
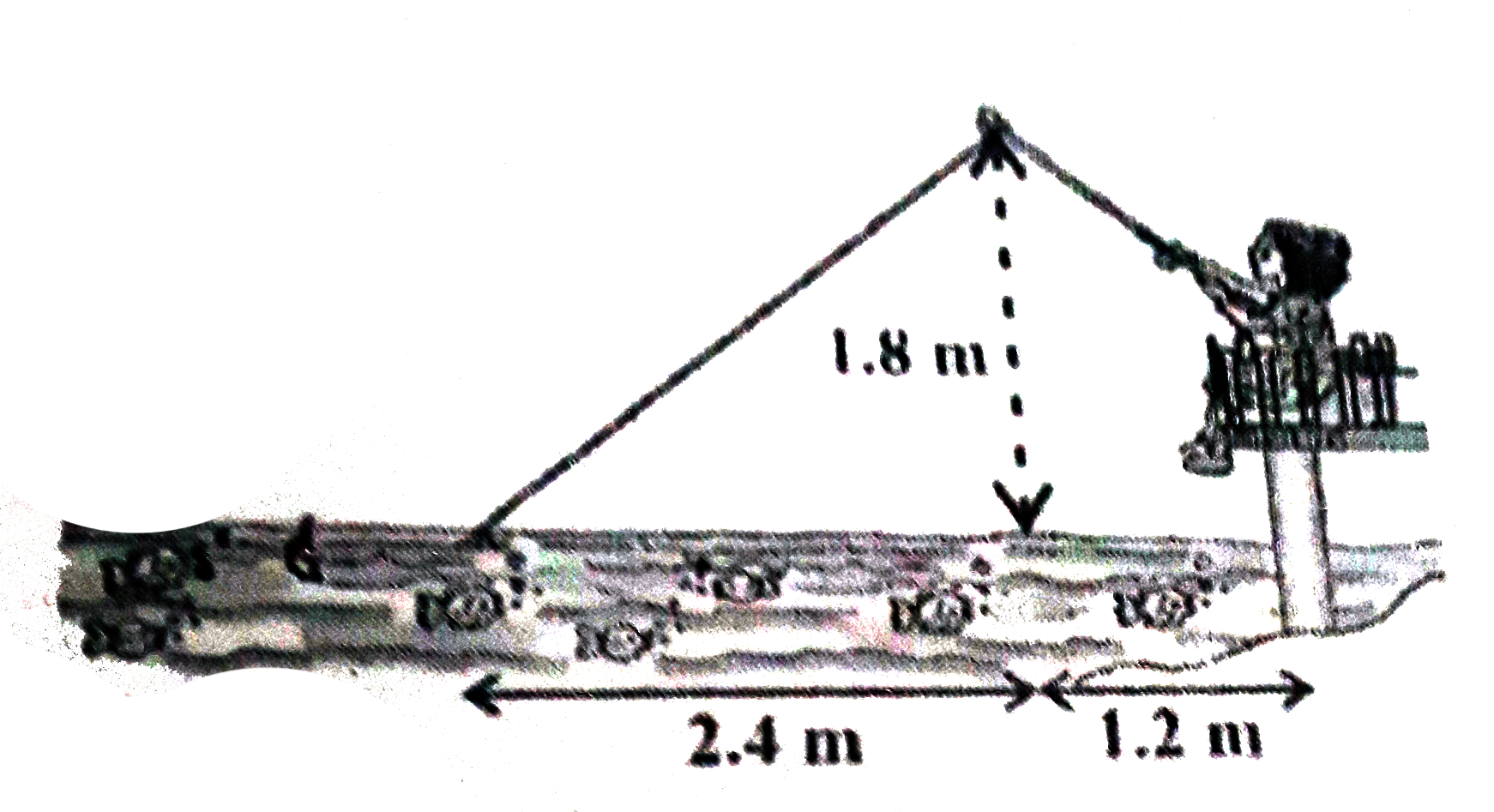
View SolutionNazima is fly fishing in a stream. The tip of her fishing rod is 1.8 m above the surface of the water and the fly at the end of the string rests on the water 3.6 m away and 2.4 m from a point directly under the tip of the rod. Assuming that her string (from the tip of her rod to the fly) is taut, how much string does she have out? If she pulls in the string at the rate of 5 cm per second, what will be the horizontal distance of the fly from her after 12 seconds?
View Solution
Knowledge Check
The amount of water remaining in a certain bathtub as it drains when the plug is pulled is represented by the equation, L=−4t2−8t+128, where L represents the number of liters of water in the bathtub and t represents the amount of time, in minutes, since the plug was pulled. Which expression represents the number of minutes it takes for half of the water that was in the bathtub before the plug was pulled to drain?
A−1+√33B−1+√17C−1+√332D−1+2√172y=−5t2+2t+20
x=2t
A cannonball is shot out of a cannon at a 45∘ angle with an approxiate speed of 283m/s. The cannon from which the ball was fired sits on the edges of a cliff, and its height above the ground is 20 meters. The equations given above represent the cannonball's height above the ground(y) and its horizontal distance (x) from the face of the cliff, t seconds after the ball was fired, where t≥0. How many seconds after the ball was fired does its vertical height above the ground equal its horizontal distance from the cliff?A1B2C3D4
Electric current in a wire is time rate of flow of charge. The charge in coulombs that passes through a wire after t seconds is given by the function q(t)=t2−2t2+5t+2. Determine the average current (in coulmbe per second) during the first two seconds.
View SolutionSushant has a vessel of the form of an inverted cone, open at the top, of height 11 cm and radius of the top as 2.5 cm and is full of water. Metallic spherical balls each of diameter 0.5 cm are put in the vessel due to which two - fifths of the water in the vessel flows out Find how many balls were put in the vessel. Sushant made the arrangement so that the water that flows out irrigates the flower beds. What value has been shown by Sushant ?
View SolutionThe ball is dropped from a bridge 122.5m above a river, After the ball has been falling for 2 s, a second ball is thrown straight down after it. What must its initial velocity be so that both hit the water at the same time ?
View SolutionWater drains out of a vessel filled with water upto height h through a hole of area A in t seconds.If the height of the water is 4 h then how much time will be required for the water to drain out ?[Assume A<<A0 (area of tank ) ]
View SolutionFor his 18th birthday in February Peter plants to turn a hut in the garden of his parents into a swimming pool with an artifical beach. In order to estimate the consts for heating the water and the house , peter obtains the data for the natural gas combustion and its price.
What is the total energy (in MJ) needed for Peter's "winter swimming pool" calculated in 1.3 and 1.4? How much natural gas will he need, if the gas heater has an efficiency of 90.0% ?
What are the different costs for the use of either natural gas or electricity ? Use the values given by PUC for your calculations and assume 100% efficiency for the electric heater.
Table 1: Composition of natural gas
Chemical substancemol fraction xD1H∘(KJmol−1)−1S∘(Jmol−1K−1)−1C∘p(Jmol−1K−1)−1CO2(g)0.0024−393.5213.637.1N2(g)0.01340.0191.629.1CH2(g)0.9732−74.6186.335.7C2H3(g)0.0110−84.0229.252.2H2Og−−285.870.075.3H2Og−−241.8188.833.6H2Og−0.0205.229.4
Equation
J=E(A.Δt)−1=!!λwall.ΔT.d−1, where
J= energy flow E along a temperature gradient (wall direction Z) par area A and time Δt, d-wall thickness , λwall -heat conductivity , ΔT - difference in temperature between the inside and the outside of the house.
View SolutionA rectangular water reservoir is 10.8mby3.75m at the base. Water flows into it at the rate of 18mper second through a pipe having the cross section 7.5cmx4.5cm. Find the height to which the water will rise in the reservoir in 30m∈utes.
View Solution
