Electric current in a wire is time rate of flow of charge. The charge in coulombs that passes through a wire after t seconds is given by the function q(t)=t2−2t2+5t+2. Determine the average current (in coulmbe per second) during the first two seconds.
4A
5A
6A
12A
The correct Answer is:B
To determine the average current during the first two seconds, we will follow these steps:
Step 1: Identify the function for charge
The charge q(t) that passes through the wire after t seconds is given by:
q(t)=t3−2t2+5t+2
Step 2: Calculate the charge at t=0 seconds
Substituting t=0 into the function:
q(0)=(0)3−2(0)2+5(0)+2=2 coulombs
Step 3: Calculate the charge at t=2 seconds
Substituting t=2 into the function:
q(2)=(2)3−2(2)2+5(2)+2
Calculating each term:
=8−8+10+2=12 coulombs
Step 4: Calculate the change in charge (Δq)
The change in charge from t=0 to t=2 seconds is:
Δq=q(2)−q(0)=12 coulombs−2 coulombs=10 coulombs
Step 5: Calculate the change in time (Δt)
The change in time from t=0 to t=2 seconds is:
Δt=2 seconds−0 seconds=2 seconds
Step 6: Calculate the average current (Iavg)
The average current is given by the formula:
Iavg=ΔqΔt
Substituting the values we calculated:
Iavg=10 coulombs2 seconds=5 coulombs per second=5 amperes
Final Answer
The average current during the first two seconds is:
5 A
The charge q(t) that passes through the wire after t seconds is given by:
q(t)=t3−2t2+5t+2
Substituting t=0 into the function:
q(0)=(0)3−2(0)2+5(0)+2=2 coulombs
Substituting t=2 into the function:
q(2)=(2)3−2(2)2+5(2)+2
Calculating each term:
=8−8+10+2=12 coulombs
The change in charge from t=0 to t=2 seconds is:
Δq=q(2)−q(0)=12 coulombs−2 coulombs=10 coulombs
The change in time from t=0 to t=2 seconds is:
Δt=2 seconds−0 seconds=2 seconds
The average current is given by the formula:
Iavg=ΔqΔt
Substituting the values we calculated:
Iavg=10 coulombs2 seconds=5 coulombs per second=5 amperes
The average current during the first two seconds is:
5 A
Topper's Solved these Questions
Similar Questions
Charge through a cross section of a conductor is give by Q=5t2−2t coulomb .Find the average current through the conduction the interval t1=2s to t2=4s .
View SolutionThe heat produced (in calories) in a resistance R when a current I amperes flows through it for t seconds is given by the expression
View Solution
Knowledge Check
- Question 1 - Select One
If an object is dropped from a tall building , then the distance it has fallen after t seconds is given by d(t)=16t2 . Find its average speed , in feet per second, between t=1 second and t=5 seconds.
A198B192C96D80 - Question 2 - Select One
A current in a wire is given by the equation, . I=2t2−3t+1, the charge through cross section of : wire in time interval t=3s to t=5s is
A32.33CB43.34CC45.5CD42C - Question 3 - Select One
When a ball is thrown straight up at an initial velocity of 54 feet per second. The height of the ball t seconds after it is thrown is given by the function h(t)=54t−12t2. How many seconds after the ball is thrown will it return to the ground?
A9.2B6C4.5D4
The current through a wire depends on time as i=(2+3t)A. Calculate the charge crossed through a cross section of the wire in 10sec.
View SolutionThe charge flowing throug a conductor beginning with time to=0 is given by the formula q=2t2+3t+1 (coulombs). Find the current i=dqdt at the end of the 5th seconds.
View SolutionIn the figure shown q is in coulomb and t in second. At time t=1s
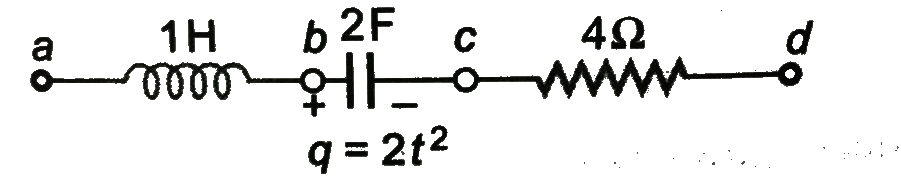 View Solution
View SolutionCharge through a cross-section of a conductor is given by Q=(2t2+5t)C. Find the current through the conductor at the instant t=2s.
View SolutionThe area of a blot of ink is growing such that after t seconds, its area is given by A=(3t2+7)cm2. Calculate the rate of increase of area at t=5 second.
View Solution

