In figure, if PQ⊥PS,PQ∣∣SR,∠SQR=280 and ∠QRT=650, then find the values of x and y. Figure
To solve the problem step by step, we will use the properties of angles in triangles and the relationships between parallel lines and transversals.
Step 1: Identify the Given Information
- PQ⊥PS (PQ is perpendicular to PS)
- PQ∥SR (PQ is parallel to SR)
- ∠SQR=28∘
- ∠QRT=65∘
Step 2: Determine Angle QSR
Since PQ∥SR and PQ is perpendicular to PS, we can use the exterior angle property of triangles. In triangle RSQ:
∠QRT=∠RQS+∠QSR
Substituting the known values:
65∘=∠RQS+28∘
Now, solve for ∠QSR:
∠QSR=65∘−28∘=37∘
Step 3: Find Angle Y
In triangle SQR, we know that the sum of angles in a triangle is 180∘. Since PS is vertical and PQ is horizontal, angle S is 90∘. Therefore:
Y+∠QSR=90∘
Substituting the value of ∠QSR:
Y+37∘=90∘
Now, solve for Y:
Y=90∘−37∘=53∘
Step 4: Find Angle X
Now, we will consider triangle PSQ. The sum of angles in triangle PSQ is also 180∘:
∠SPQ+∠PQS+∠QSP=180∘
We know:
- ∠SPQ=90∘
- ∠QSP=Y=53∘
- Let ∠PQS=X
Substituting the known values:
90∘+X+53∘=180∘
Now, simplify and solve for X:
X+143∘=180∘
X=180∘−143∘=37∘
Final Values
- Y=53∘
- X=37∘
- PQ⊥PS (PQ is perpendicular to PS)
- PQ∥SR (PQ is parallel to SR)
- ∠SQR=28∘
- ∠QRT=65∘
Since PQ∥SR and PQ is perpendicular to PS, we can use the exterior angle property of triangles. In triangle RSQ:
In triangle SQR, we know that the sum of angles in a triangle is 180∘. Since PS is vertical and PQ is horizontal, angle S is 90∘. Therefore:
Now, we will consider triangle PSQ. The sum of angles in triangle PSQ is also 180∘:
- ∠SPQ=90∘
- ∠QSP=Y=53∘
- Let ∠PQS=X
X=180∘−143∘=37∘
- Y=53∘
- X=37∘
Topper's Solved these Questions
Similar Questions
In Figure, if PQ⊥PS, PQSR, ∠SQR=280and ∠QRT=650, then find the values of x and y
View SolutionIn Fig. 6.43, if PQ⊥PS,PQ∣∣SR,∠SQR=28∘ and ∠QRT=65∘, then find the values of x and y.

View SolutionIn Figure , find out ∠PXR, if PQ ∣∣ RS.
View SolutionIn figure, PQ=PR . Show that PS>PQ.
View SolutionIn Figure, if QT⊥PR, ∠TQR=40∘ and ∠SPR=30∘, find x and y.
View SolutionIn Fig. 6.31, if PQ∣∣ST,∠PQR=110∘ and∠RST=130∘, find ∠QRS.
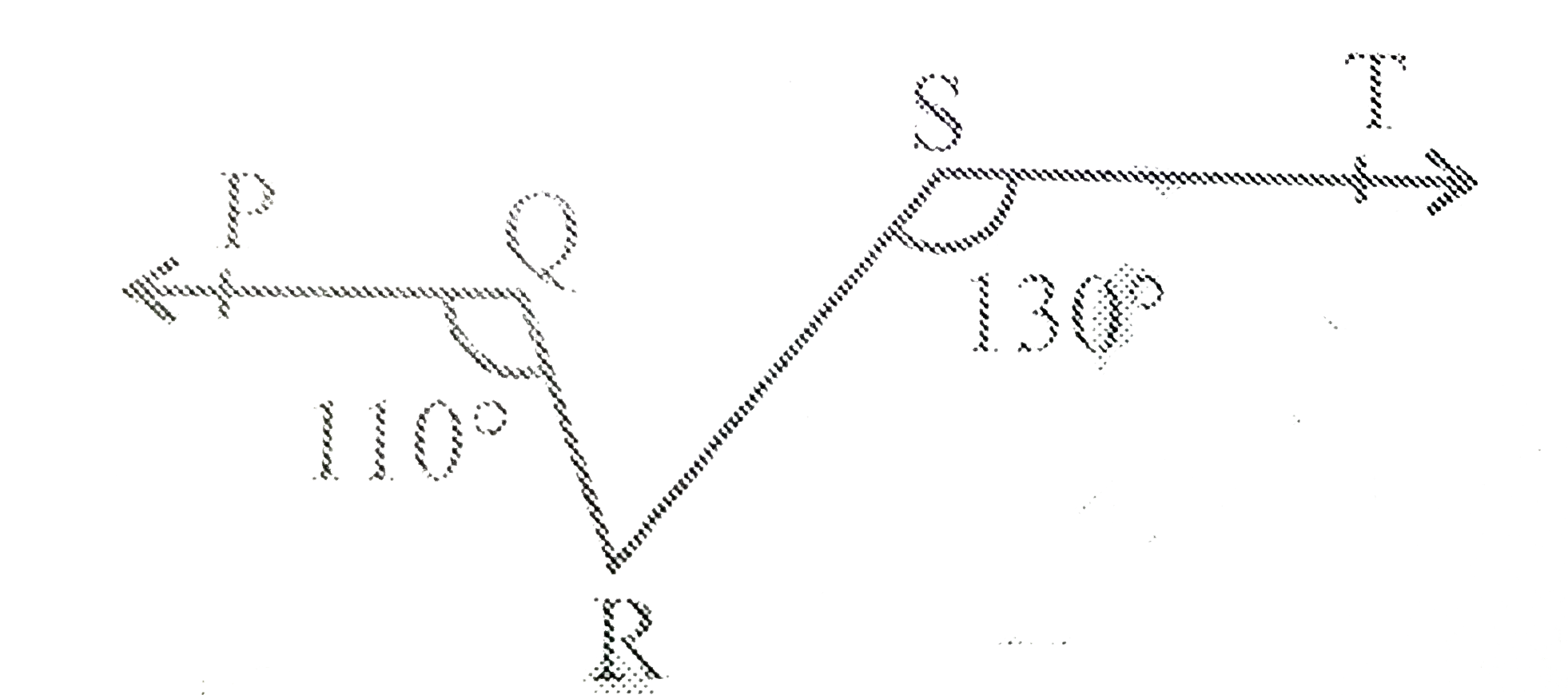
View SolutionIf Figure, if QT⊥PR,∠TQR=400 AND ∠SPR=300, find x and y. Figure
View SolutionIn Figure, OP∣∣RS. Determine ∠PQR
View SolutionIn Figure, if PQ∣∣RS, ∠MXQ=135∘and ∠MYR=40∘,find ∠XMY
View SolutionIn Figure, ∠PQR= ∠PRQ, then prove that ∠PQS= ∠PRT.
View Solution
